If the equation ∣∣x2+bx+c∣∣=k has four real roots, then b2−4c>0and0<k<4c−b24 b2−4c<0and0<k<4c−b24 b2−4c>0andk>4c−b24 none of theseSolution in Tamil
We have ∣∣x2+bx+c∣∣=k
For the equation to have four real roots, the line y=k must intersect y=∣∣x2+bx+c∣∣ at four points, which is not possible if D=0orD<0 as the possible graphas of y=∣∣x2+bx+c∣∣ will be as follows.
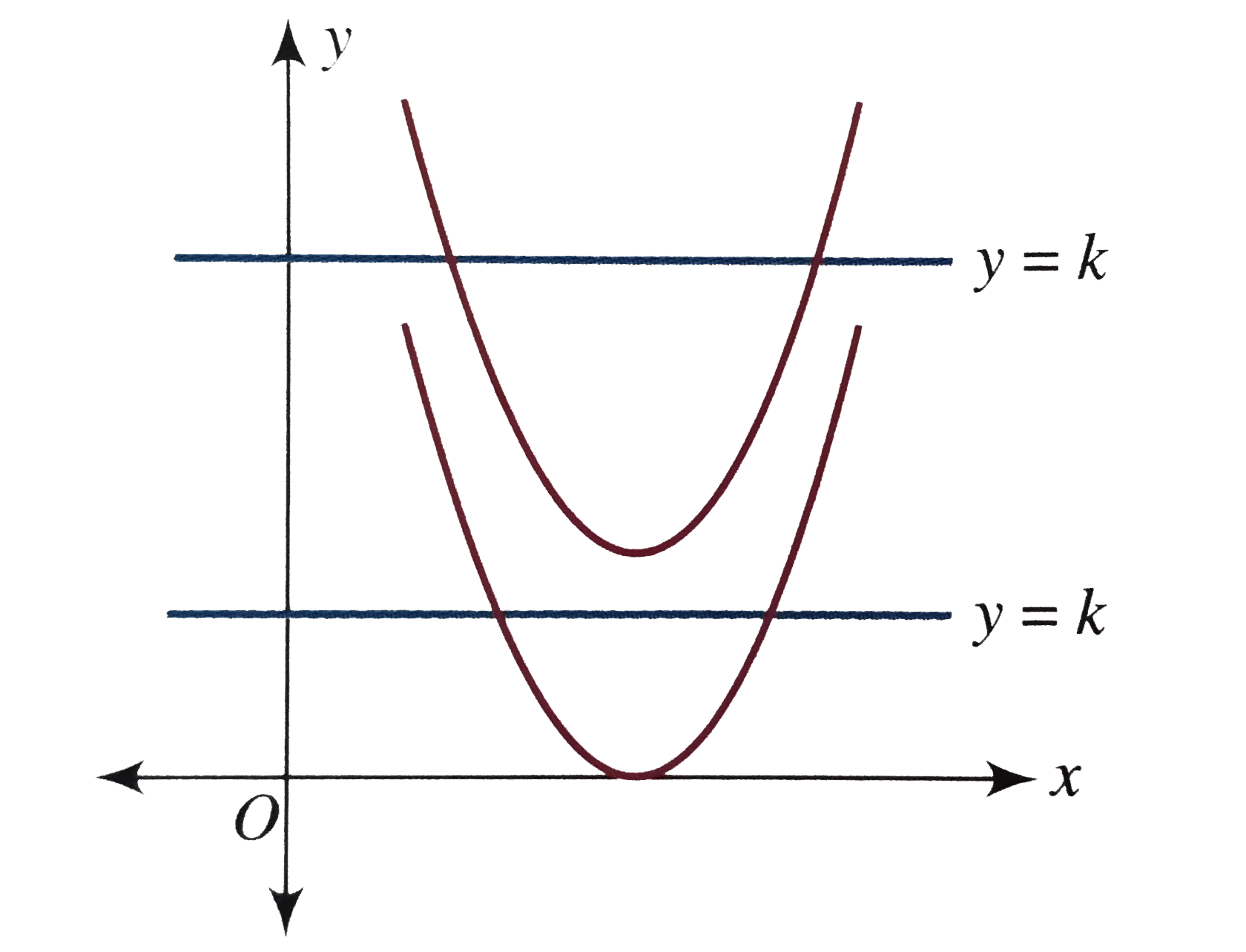
For the above graphs, the equation ∣∣x2+bx+c∣∣=k can have maximum two real roots. So for four real roots, we must have D>0, so the graph of y=x2+bx+c is as follows.
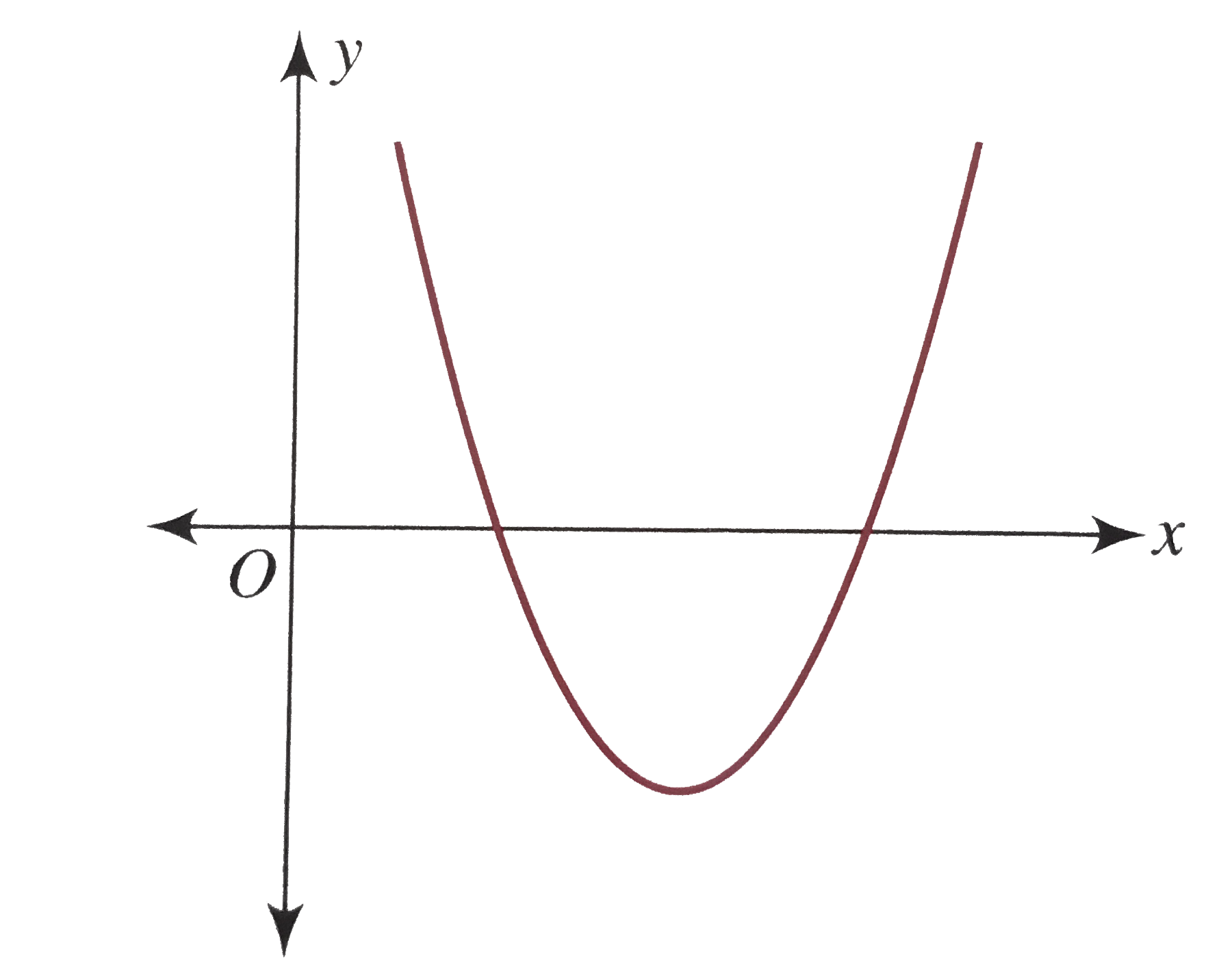
So the graph of y=∣∣x2+bx+c∣∣ will be as follows.
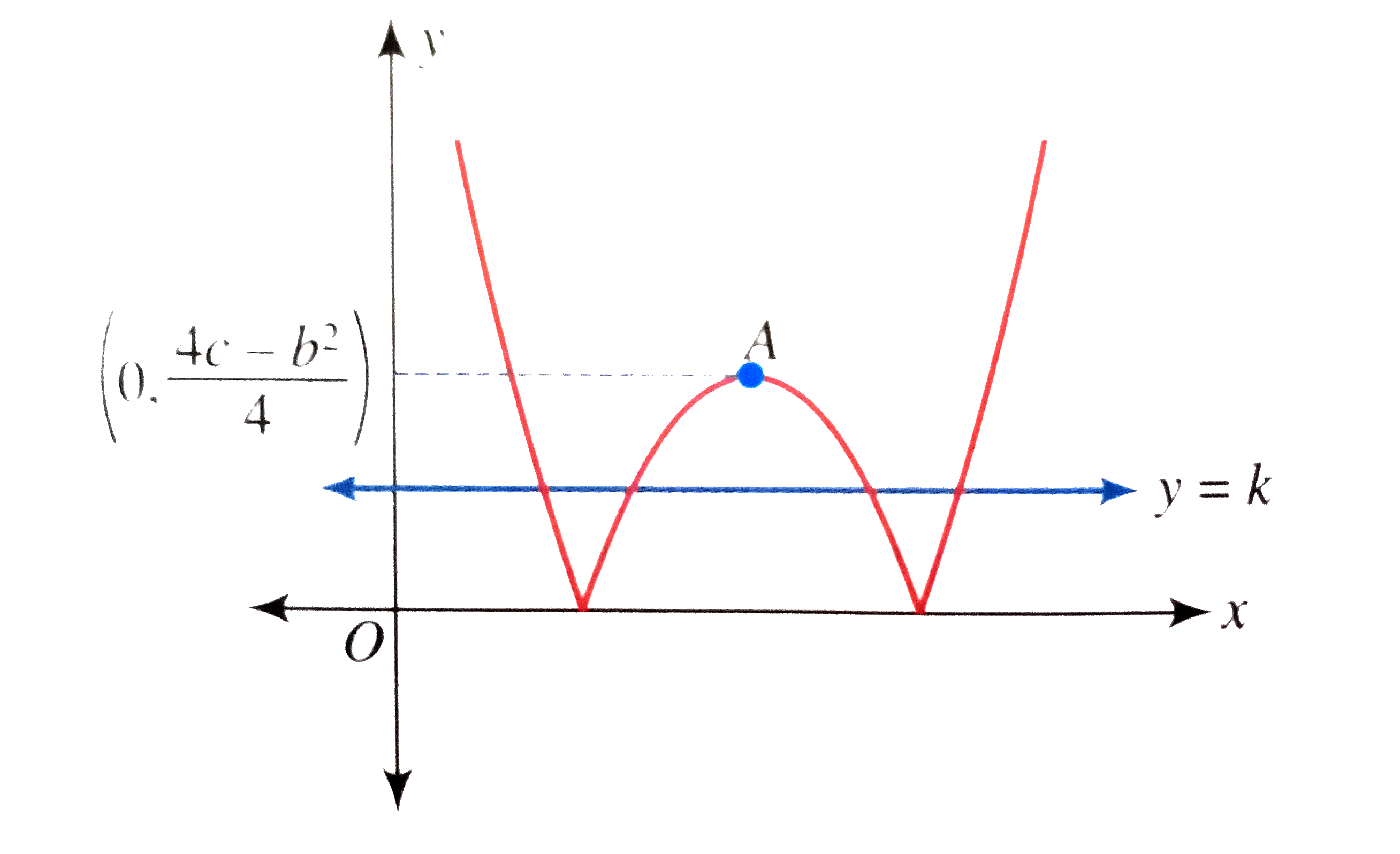
Now the equation ∣∣x2+bx+c∣∣=k will have four real if the line y=k intersects the above graph between the x-axis and point A.
Hence we have 0<k<4c−b24
Also D>0∴b2−4c>0.
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - Exercise14videos
GRAPHICAL TRANSFORMATIONS
- BOOK - CENGAGECHAPTER - GRAPH OF INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise18videos
GRAPH OF INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises4videos
GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONS
Similar Questions
Draw the graph of y=tan−1x−cot−1x.
01:38View SolutionDraw the graph of y=tan−1x+cot−1x
05:00View SolutionSketch the graph of an example of a rational function f that satisfies all the given conditions.
(i) f(0)=0,f(1),limx→∞f(x)=0,f is odd
(ii) limx→0+f(x)=∞,limx→0−f(x)=−∞,limx→∞f(x)=1,limx→−∞f(x)=1
(iii) limx→−2f(x)=∞,limx→−∞f(x)=3,limx→∞f(x)=3,f(0)=003:29View Solutiona^(2)+b^(2)+4c^(2)=2a+2b-4c+3=0
01:50View SolutionIf both roots of the equation ax2+x+c−a=0 are imaginary and c>−1, then a.3a>2+4c b.3a<2+4c c.c<a d. none of these
03:57View SolutionIf the equation ∣∣x2+bx+c∣∣=k has four real roots, then
A. b2−4c>0and0<k<4c−b24
B. b2−4c<0and0<k<4c−b24
C.b2−4c>0andk>4c−b24
D. none of these00:35View Solutiona^(2)-b^(2)-4bc+4c^(2)
02:16View SolutionConsider a circle x2+y2+ax+by+c=0 lying completely in the first quadrant. If m1andm2 are the maximum and minimum values of yx for all ordered pairs (x,y) on the circumference of the circle, then the value of (m1+m2) is (a)a2−4cb2−4c (b) 2abb2−4c (c)2ab4c−b2 (d) 2abb2−4ac
02:14View Solutionb^(2)+c^(2)+2bc+4b+4c
04:44View Solutiona2−b2−4ac+4c2
04:15View SolutionIf the roots of the equation x2−bx+c=0 are two consecutive integers, then find the value of b2−4c
03:43View SolutionIf the equation ∣∣x2+bx+c∣∣=k has four real roots, then
A. b2−4c>0and0<k<4c−b24
B. b2−4c<0and0<k<4c−b24
C.b2−4c>0andk>4c−b24
D. none of these03:20View SolutionConsider a circle x2+y2+ax+by+c=0 lying completely in the first quadrant. If m1andm2 are the maximum and minimum values of yx for all ordered pairs (x,y) on the circumference of the circle, then the value of (m1+m2) is (a)a2−4cb2−4c (b) 2abb2−4c (c)2ab4c−b2 (d) 2abb2−4ac
08:54View SolutionIf the equation ∣∣x2+bx+c∣∣=k has four real roots, then a. b2−4c>0 and 0<k<4c−b24 b. b2−4c<0 and 0<k<4c−b24 c. b2−4c>0 and k>4c−b24 d. none of these
02:06View SolutionConstruct a quadrilateral ABCD in which AB=6cm, BC=4cm, CD=4cm, ∠B=950and ∠C=900.
06:49View SolutionResolve into factors a^4b^4(a^2-b^2)+b^4c^4(b^2-c^2)+c^4a^4(c^2-a^2)
04:02View Solution

