Ifp>q>0andpr<−1<qr, then find the value of tan−1(p−q)1+pq+tan−1(q−r)1+qr+tan−1(r−p)1+rp.
since p,q>0, we have pq>0
tan−1(p−q)1+pq=tan−1p−tan−1q....(i)
Since qr>−1, we have
tan−1(q−r)1+qr=tan−1q−tan−1r...(ii)
Since pr<−1andr<0, we have
tan−1(r−p)1+rp=π+tan−1t−tan−1p....(iii)
On adding Eqs. (i), (ii), and (iii), we get
tan−1(p−q)1+pq+tan−1(q−r)1+qr+tan−1(r−p)1+rp=π
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise 7.112videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGECHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise 7.26videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGECHAPTER - INTRODUCTION TO VECTORS EXERCISE - JEE Previous Year20videos
INTRODUCTION TO VECTORS
- BOOK - CENGAGECHAPTER - JEE 2019EXERCISE - Chapter 109videos
JEE 2019
Similar Questions
If p>qandr<0 , then which is true? (a) pr < qr (b) p r < q r (c) p + r < q + r (d) None of these
View SolutionIf a,bandc are in A.P., and pandp' are respectively, A.M. and G.M. between aandbwhileq,q' are , respectively, the A.M. and G.M. between bandc, then p2+q2=p'2+q'2 b. pq=p'q' c. p2−q2=p'2−q'2 d. none of these
View Solution
Knowledge Check
- Question 1 - Select One
If rthand(r+1)th terms in the expansion of (p+q)n are equal, then (n+1)qr(p+q) is
A12B14C1D0 - Question 2 - Select One
If roots of the equation (q−r)x2+(r−p)x+(p−q)=0 are equal, then p,q and r are in
AAPBGPCHPDAGP
If P and Q are the mid points of the sides AB and CD of a parallelogram ABCD, prove that DP and BQ cut the diagonal AC in its points of trisection which are also the points of trisection of DP and BQ respectively.
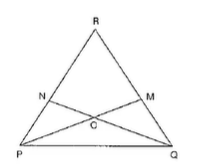
View SolutionIn Figure, ∠QPR= ∠PQR and M and N are respectively on sides QR and PR of PQR such that QM=PN. Prove that OP=OQ, where O is the point of intersection of PM and QN
 View Solution
View SolutionIf p, q, r are not in G.P. and ∣∣ ∣ ∣∣1qpα+qp1rpα+rqpα+qqα+r0∣∣ ∣ ∣∣=0 Show that pα2+2qα+r=0
View SolutionIn Figure, ∠QPR=∠PQR and M and N are respectively on sides QR and PR of PQR such that QM=PN . Prove that OP=OQ, where O is the point of intersection of PM and QN.
 View Solution
View SolutionIf rth and (r+1)th term in the expansion of (p+q)n are equal, then (n+1)qr(p+q) is
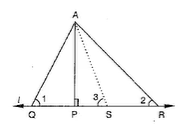
View SolutionIn Figure, AP ⊥l and PR>PQ . Show that AR>AQ Given: AP⊥l and PR>PQ To Prove: AR>AQ Construction: Mark a point S on PR such that PS=PQ. Join AS
 View Solution
View Solution

