Let cos−1(4x3−3x)=a+bcos−1x
If x∈(12,1], then the value of limy→abcos(y) is
−1/3
−3
13
3
The correct Answer is:D
Let cos−1x=θ or x=cosθ, where θ∈[0,π]
cos−1(4x3−3x)=cos−1(4cos3θ−3cosθ)=cos−1(cos3θ)=cos−1(cosα)
where α=3θ∈[0,3π]
Refer to the graph of y=cos−1(cosα),α∈[0,3π]
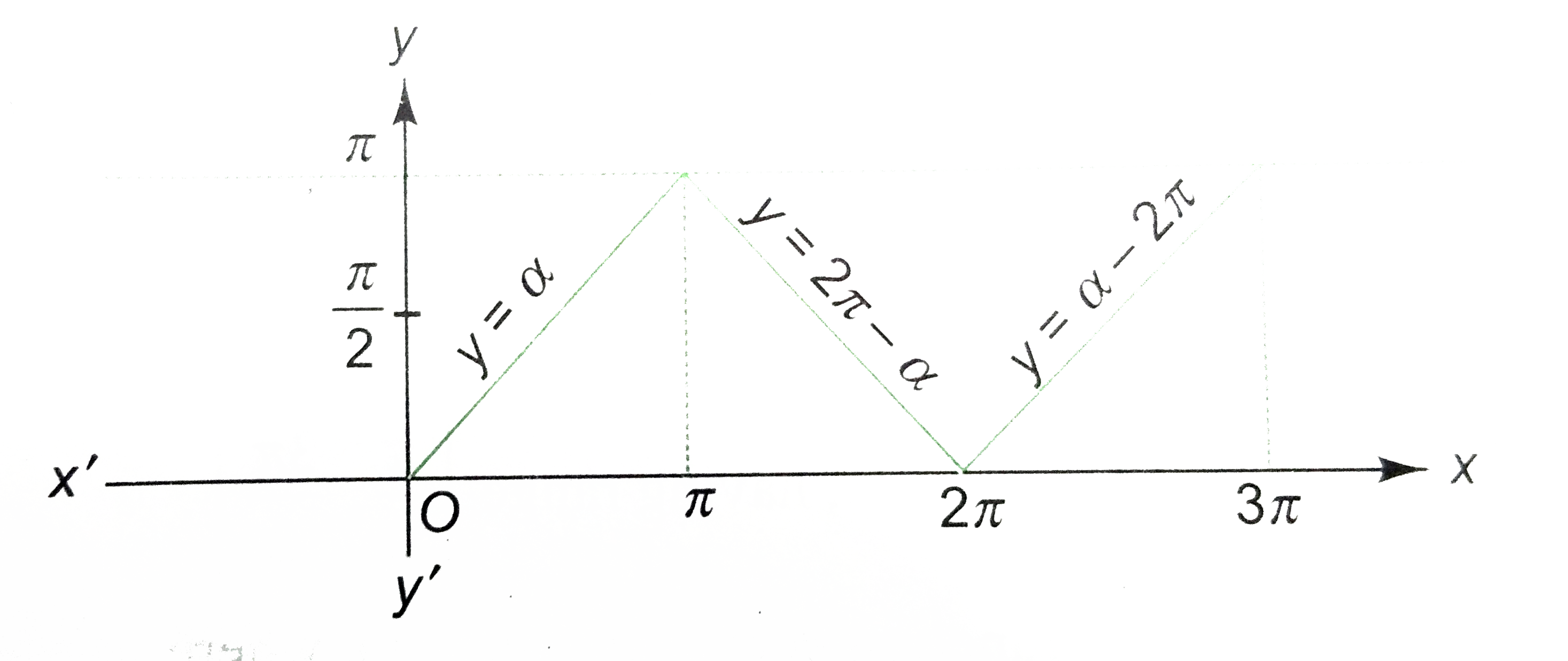
From the graph,
cos−1(4x3−3x)=cos−1(cosα)
=⎧⎪⎨⎪⎩α0≤α<π2π−απ≤α≤2πα−2π2π<α≤3π
=⎧⎪⎨⎪⎩3cos−1x0≤3cos−1x<π2π−3cos−1xπ≤3cos−1x≤2π3cos−1x−2π2π<3cos−1x≤3π
=⎧⎪⎨⎪⎩3cos−1x0≤3cos−1x<π2π−3cos−1x(π≤3)cos−1x≤(2π/3)3cos−1x−2π(2π/3)<cos−1x≤π
=⎧⎪
⎪⎨⎪
⎪⎩3cos−1x(1/2)<x≤12π−3cos−1x(−1/2)≤x≤(1/2)3cos−1x−2π−1≤x<−(−1/2)
=⎧⎪
⎪⎨⎪
⎪⎩3cos−1x−2π−1≤x<(−1/2)2π−3cos−1x(−1/2)≤x≤(1/2)3cos−1x(1/2)<x≤1
For x∈[−1,−12),cos∧(−1)(4x3−3x)=3cos−1x−2π
⇒a=−2πandb=3⇒a+bπ=π
For x∈[−12,12],cos−1(4x3−3x)=2π−3cos−1x
⇒a=2πandb=−3
⇒sin−1(sin.ab)=sin−1(sin.2π−3)=sin−1(−√32)=−π3
For x∈(12,1],cos−1(4x3−3x)=3cos−1x
→a=0andb=3
∴lomy→abcos(y)=limy→03cos(y)=3
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Matrix match type8videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Numerical value type18videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Multiple correct answer type24videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - INTRODUCTION TO VECTORS EXERCISE - MATRIX-MATCH TYPE3videos
INTRODUCTION TO VECTORS
- BOOK - CENGAGE ENGLISHCHAPTER - JEE 2019EXERCISE - Chapter 109videos
JEE 2019
Similar Questions
Let cos−1(4x3−3x)=a+bcos−1x
If x∈[−1,−12), then the value of a+bπ isView SolutionDefine y=cos−1(4x3−3x) in terms of cos−1x
View SolutionLet cos−1(4x3−3x)=a+bcos−1x
Q. If x∈[−12,12], then sin−1(sinab) is :View SolutionIf limx→0x(1+acosx)−bsinxx3=1, then the value of ab is euqal to
View SolutionThe value of limx→∞x2(1−cos.1x) is
View SolutionIf sin−1x+sin−1y=π3 and cos−1x−cos−1y=π6 , find the values of x and y .
View SolutionIf cos−1(x3)+cos−1(y2)=θ2, then the value of 4x2−12xycos(θ2)+9y2 is equal to :
View SolutionLet a=cos−1cos20,b=cos−1cos30andc=sin−1sin(a+b) then
If 5sec−1x+10sin−1y=10(a+b+c) then the value of tan−1x+cos−1(y−1) isView Solutionlimx→0cos2x−1cosx−1
View SolutionLet x+1x=2,y+1y=−2 and sin−1x+cos−1y=mπ, then the value of m is
View Solution

