Maximise Z=5x+3y
Subject to 3x+5y≤15,5x+2y≤10,x≥0,y≥0.
Video Solution
Text Solution
Maximise Z=5x+3y………………1
Subject to 3x+5y≤15 …………..2
5x+2y≤10………………..3
xg30,y≥0………………….4
First we draw the graph of the line 3x+5y=15
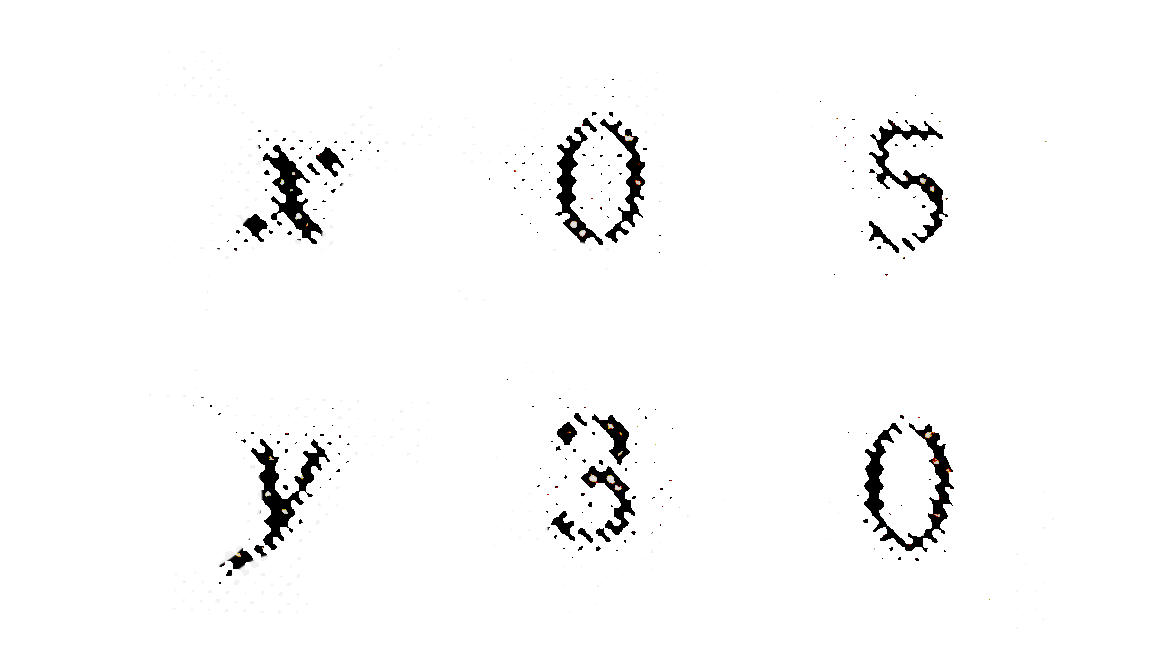
Put (0,0) in the inequation 3x+5y≤15
3×0+5×0≤15⇒0≤15 (True)
Therefore, half plane contains origin.
Since x,y≥0
Therefore feasible region in first quadrant
Now draw the graph of the line 5x+2y=10.

Put (0,0) in the inequation 5x+2y≤10
5×0+2×0≤10⇒0≤10
Therefore half plane contains origin.
From the equation 3x+5y=15
adn 5x+2y=10,
We get x=2019 and y=4519
Therefore, coordinates of point B are (2019,4519)
Therefore, feasible region of OABCO.
Thus, the vertices of the feasible region ar O(0,0),A(2,0),B(2019,4519) and C(0,3). We find the value of Z at these vertices.

Therefore the maximum value of Z is 2359 which is obtained at point B(2019,4519)
|
Topper's Solved these Questions
- LINEAR PROGRAMMINGBook:NAGEEN PRAKASHANChapter:LINEAR PROGRAMMINGExercise:Exercise 12.2
Explore 11 Videos
- LINEAR PROGRAMMINGBook:NAGEEN PRAKASHANChapter:LINEAR PROGRAMMINGExercise:Miscellaneous Exercise
Explore 9 Videos
- LINEAR PROGRAMMINGBook:NAGEEN PRAKASHANChapter:LINEAR PROGRAMMINGExercise:Exercise 12 B
Explore 17 Videos
- INVERES TRIGONOMETRIC FUNCTIONSBook:NAGEEN PRAKASHANChapter:INVERES TRIGONOMETRIC FUNCTIONSExercise:Miscellaneous Exercise (prove That )
Explore 9 Videos
- MATRICESBook:NAGEEN PRAKASHANChapter:MATRICESExercise:Miscellaneous Exerice
Explore 15 Videos
Similar Questions
Knowledge Check
The maximum value of z=15x+30y subject to 3x+y≤12,x+2y≤10,x≥0,y≥0 is
A60B150C160D100
Minimise Z=−3x+4y
Subject to x+2y≤8,3x+2y≤12,x≥0,y≥0.
View SolutionSolve the following linear programming problem graphically:
Maximize : z=5x+3y
Subject to:
x+3y≤5
x+y≤3
x≥0
y≥0
View SolutionMaximise Z=3x+2y
Subject to x+2y≤10,3x+y≤15,x,y≥0
View SolutionMaximise Z=x+y
Subject to x−y≤−1,−x+y≤0,x,y≥0.
View SolutionMinimise and Maximise Z=x+2y
Subject to x+2y≥100,2x−y≤0,2x+y≤200,x,y≥0.
View SolutionSolve the following linear programming problem graphically:
Maximize : z=60x+15y
Subject to : x+y≤50
3x+y≤90
x≥0
y≥0
View SolutionSolve the following linear programming problem graphically:
Maximize :z=x+2y
Subject to: x−y≤2
x+y≤4
x≥0
y≥0
View Solution