Complete Solutions to Comprehension Type of chapter CIRCLES of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | CIRCLES |
| CENGAGE NEW DPP | Comprehension Type |
| Number Of Questions | 8 |
| Academic Year | 2023-24 |
In the diagram as shown, a circle is drawn with centre C(1, 1) and radius I and a line L. The line Lis tangential to the circle at Q. Further L meet the y-axis at R and the x-axis at Pis such a way that the angle OPQ equals `theta` where `0 < theta
View Solution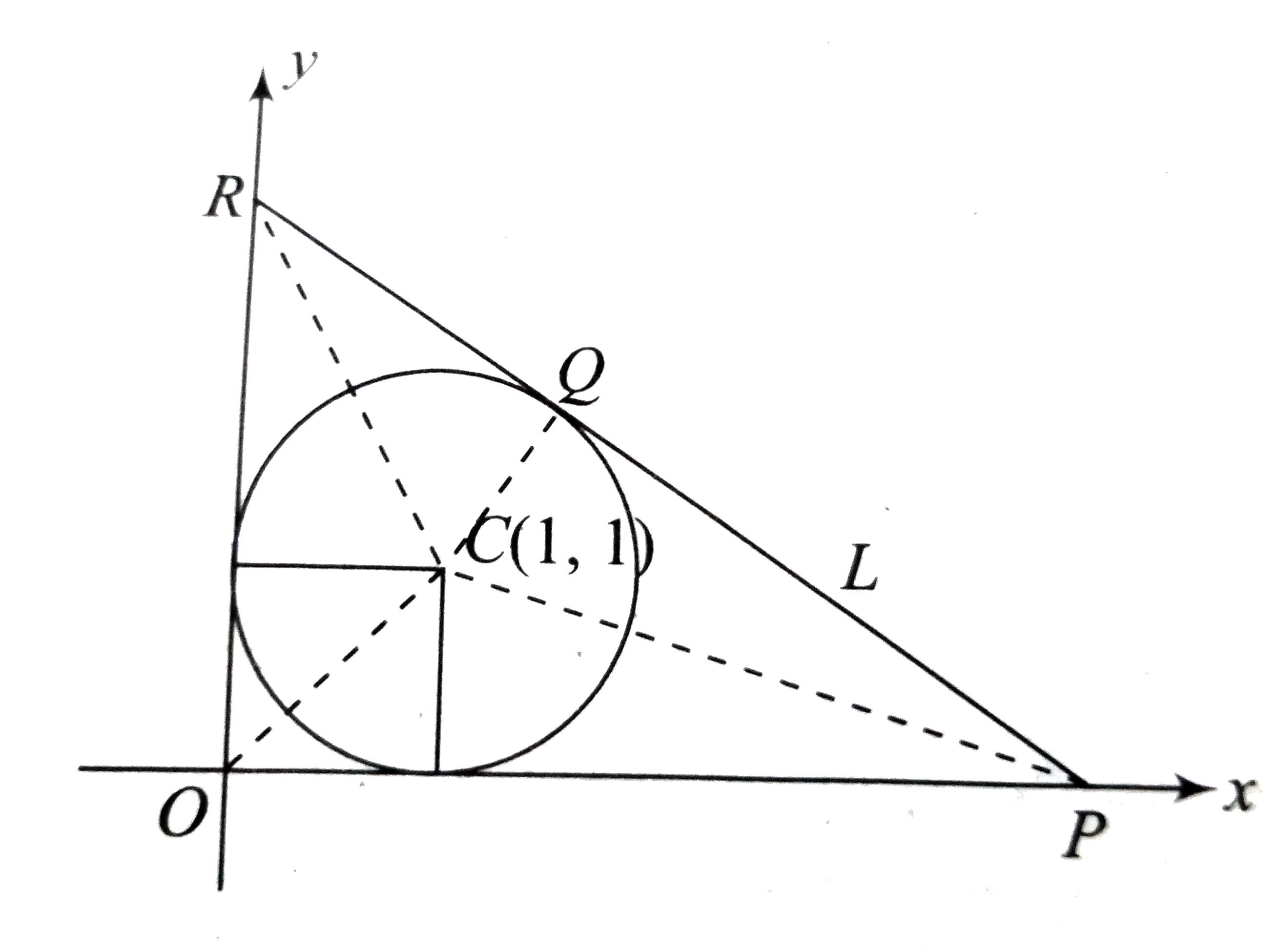
In the diagram as shown, a circle is drawn with centre `C(1,1)` and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals `theta` where `0 lt theta lt (pi)/(2)`.
Equation of the line PR isView Solution
In the diagram as shown, a circle is drawn with centre `C(1,1)` and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals `theta` where `0 lt theta lt (pi)/(2)`.
Area of triangle OPR when `theta = pi//4` isView SolutionLet `P(alpha,beta)` be a point in the first quadrant. Circles are drawn through P touching the coordinate axes.
Radius of one of the circles isView Solution`P` is a point `(a , b)` in the first quadrant. If the two circles which pass through `P` and touch both the coordinates axes cut at right angles, then `a^2-6a b+b^2=0` `a^2+2a b-b^2=0` `a^2-4a b+b^2=0` `a^2-8a b+b^2=0`
02:56View SolutionLet `P(alpha,beta)` be a point in the first quadrant. Circles are drawn through P touching the coordinate axes.
Equation of common chord of two circles isView Solution`P(a,5a)` and `Q(4a,a)` are two points. Two circles are drawn through these points touching the axis of y.
Centre of these circles are atView SolutionTwo circles are drawn through the points `(a,5a) and (4a, a)` to touch the y-axis. Prove that they intersect at angle `tan^-1(40/9).`
View Solution
Comprehension Type questions and complete solutions for chapter CIRCLES of CENGAGE of Class 12
