Complete Solutions to Exercise of chapter GETTING STARTED WITH GRAPHS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | GETTING STARTED WITH GRAPHS |
| CENGAGE NEW | Exercise |
| Number Of Questions | 18 |
| Academic Year | 2023-24 |
Does the following graph pass the vertical or horizontal line test ?
 02:13View Solution
02:13View SolutionConsider the graph of y = f(x) as shown in the following figure.
(i) Find the sum of the roots of the equation f (x) = 0.
(ii) Find the product of the roots of the equation f(x) = 4.
(iii) Find the absolute value of the difference of the roots of the equation f(x) = x+2 .02:54View SolutionWhich of the following functions has (have) y-symmetry or origin symmetry?
(i) ` f(x) = x^(2) sin x" "(ii) f(x) = log ((1-x)/(1+x))`
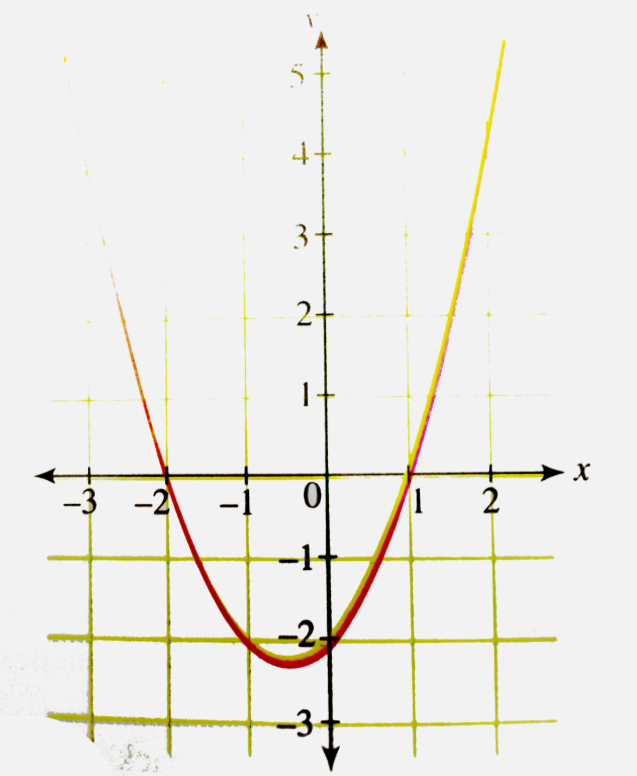
(iii)` f(x) = x/(e^(x)-1)+x/2 + 1`05:03View SolutionThe graph of functions are given in the following fingure. Discuss the symmetry.
 01:59View Solution
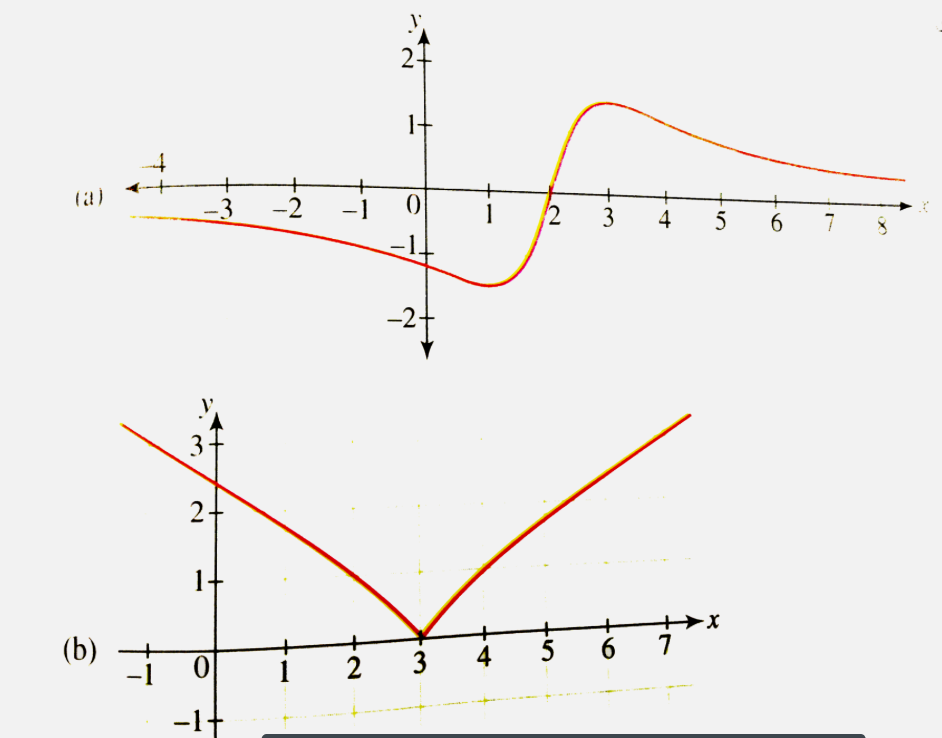
01:59View SolutionCheck weather the following function/functions is/are periodic or not? Find the period in case the function is periodic.
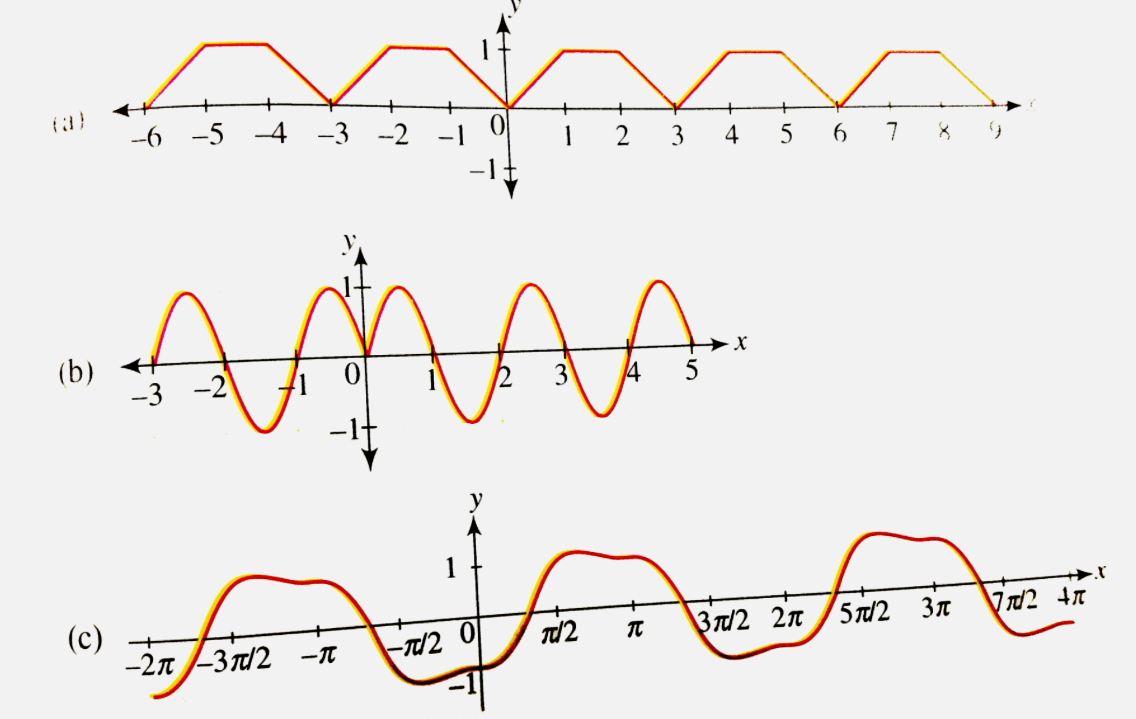 02:57View Solution
02:57View SolutionSuppose that f is even, periodic function with period 2, and that f(x) = x for all x in the interval [0, 1]. Then draw the graph of y = f(x).
02:57View SolutionThe graph of y = f(x) is as shown in the following figure.

Find the following values
(i)` f(-3)" "(ii) f(-2)" "(iii) f(0)`
(iv)` f(2)" "(v) f(3)" "(vi) lim_(x to -3) f(x)`
(vii)` lim_(x to 0) f(x)" "(viii)lim_(x to 2) f(x)" "(ix) lim_(x to 3) f(x)`
`(x) lim_(x to 2^(-)) f(x)" "(xi)lim_(x to -2^(+)) f (x)" "(x ii)lim_(x to 0^(-)) f(x)`
(x iii) `lim_(x to 0^(+)) f(x)`06:19View SolutionThe graph of f is shown. State, with reason, the numbers at which f is not differentiable.
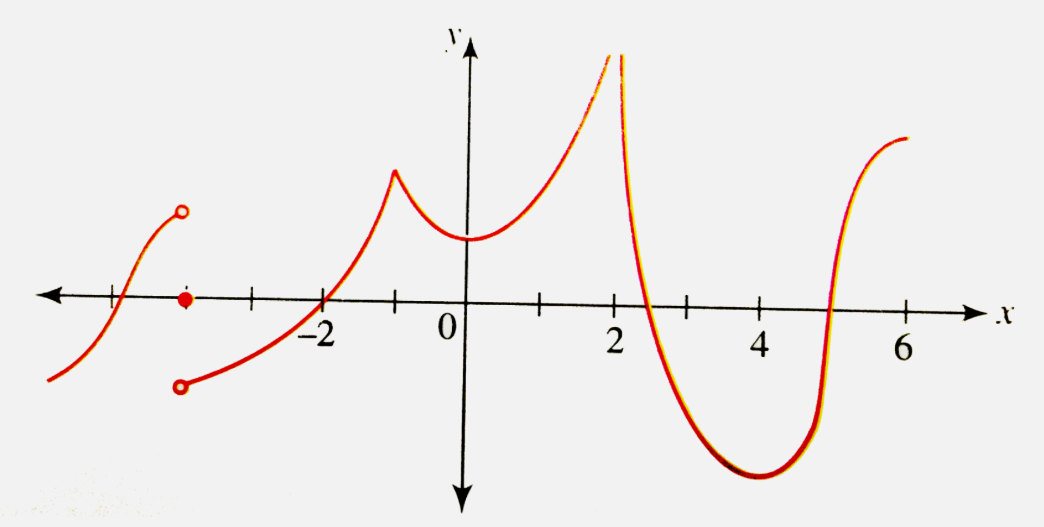 02:25View Solution
02:25View SolutionIf `f:R→R, g:R→R ` are given by `f(x)=(x+1)^2 ` and `g(x)=x^2+1 `, then write the value of `fog(−3)`.
02:08View Solution(a) Can the graph of the function intersect the horizontal asymptote?
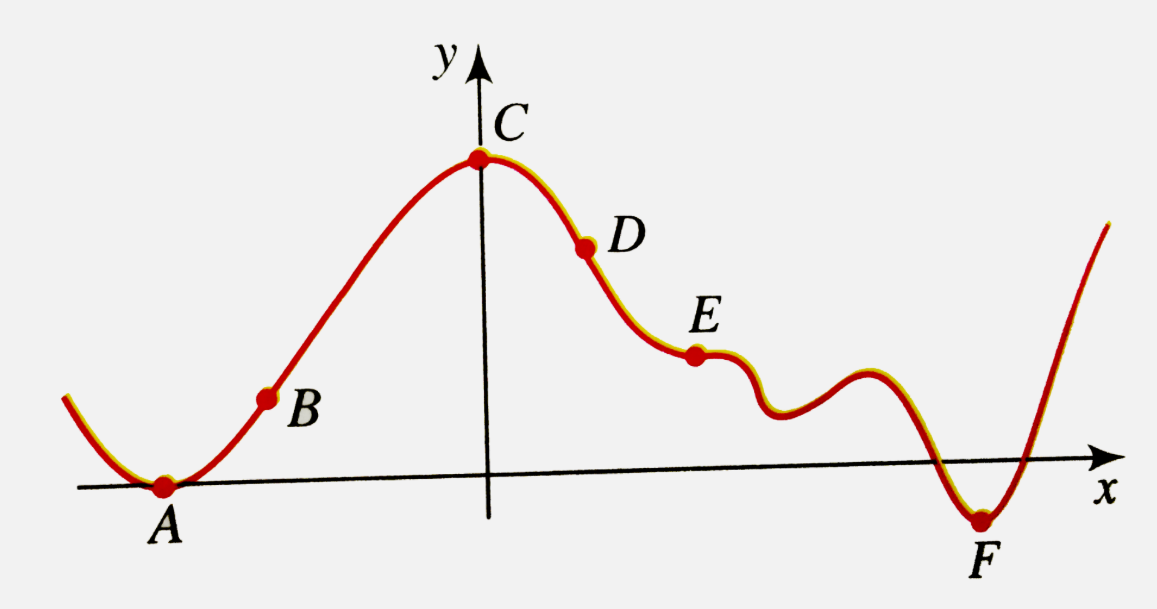
(b) Can the graph of the function intersect the vertical asymptote?03:06View SolutionThe graph of y = f(x) is given with six labelled points. Anser the following questions.
(a) At which point f'(x) has the greatest value?
(b) At which point f(x) and f'(x) both are zero?
(c) At how many point f'(x) is negative ?
(d) Which is the point of infection?
 04:05View Solution
04:05View SolutionGraph of functions are given in the following figure. Check the functions for absolute extremum.
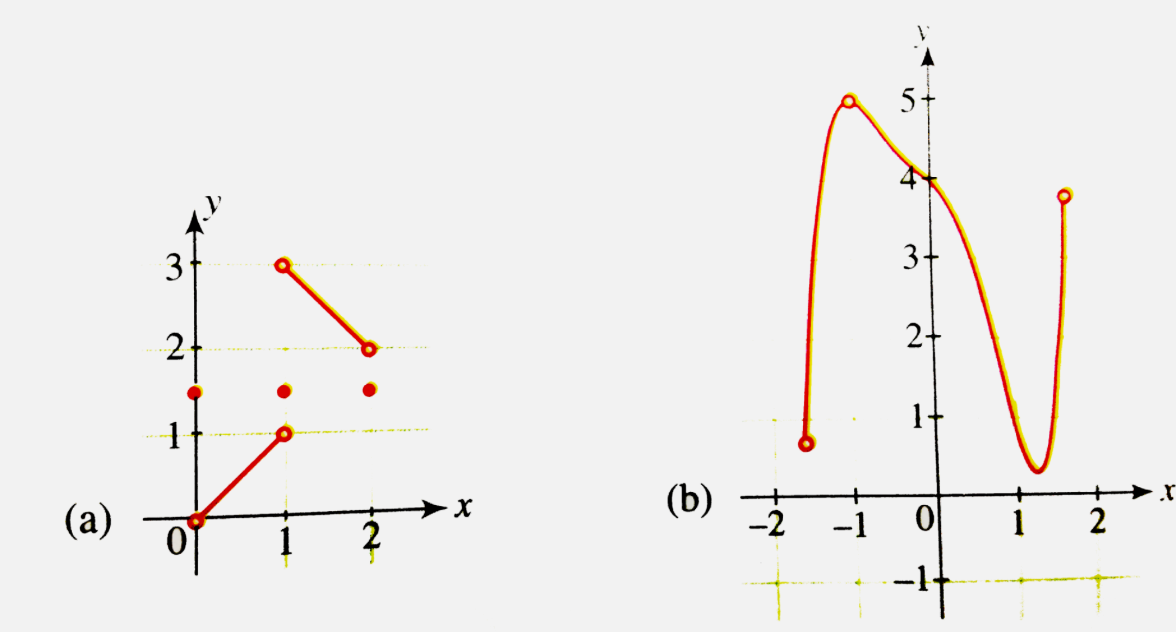
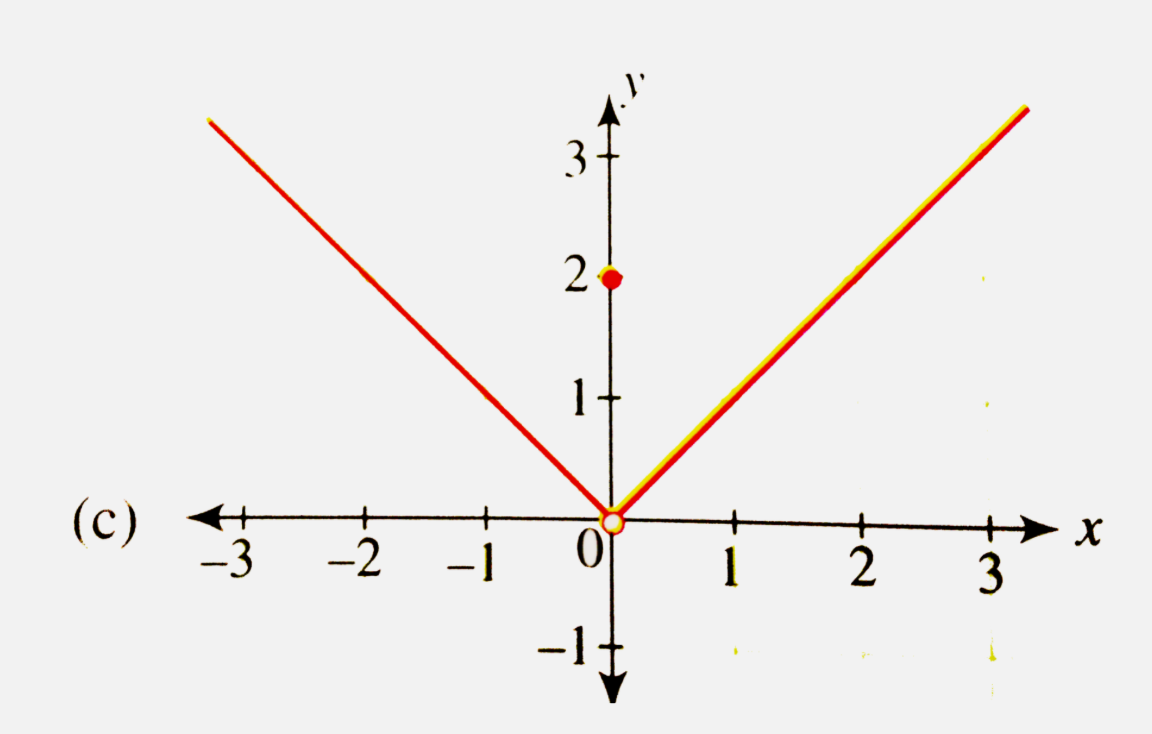 04:04View Solution
04:04View SolutionIn the following graph, state the absolute and local maximum and minimum values of the function.
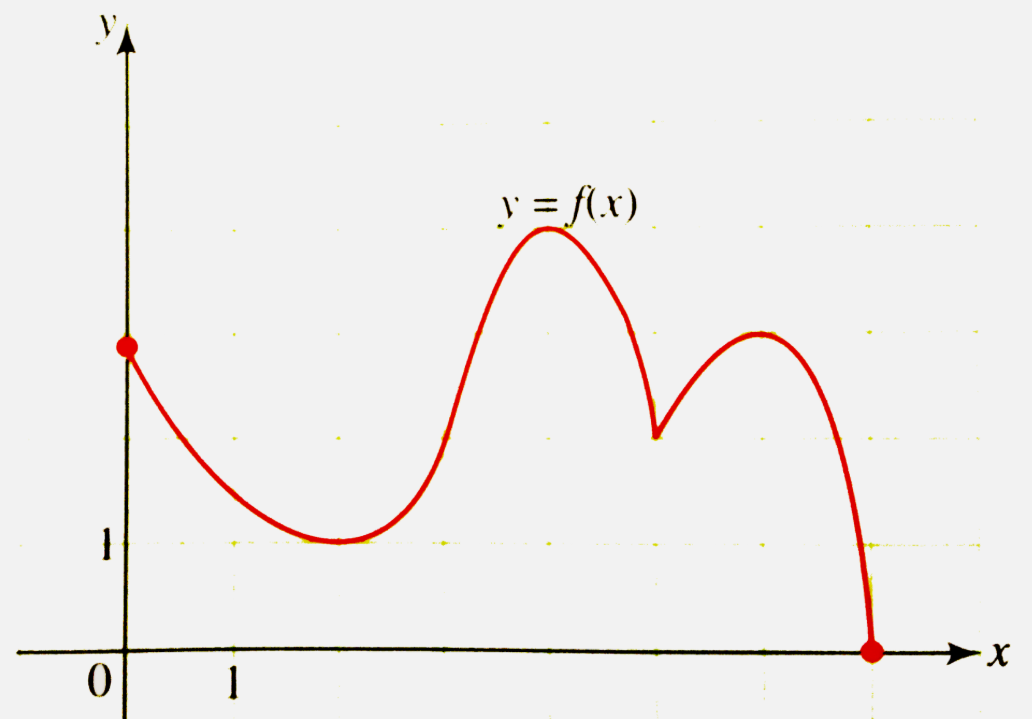 04:10View Solution
04:10View SolutionProve : ` ((1+cot^2 A)/(1+tan^2 A))=((1−cotA)/(1−tanA))^2=tan^2 A`
03:30View SolutionEvaluate `int_(0)^(pi/2)(sinx/(cosx+sinx))dx`
02:53View SolutionThe figure shows the graphs of f, f' and f''. Indentify each curve and explain your choices.
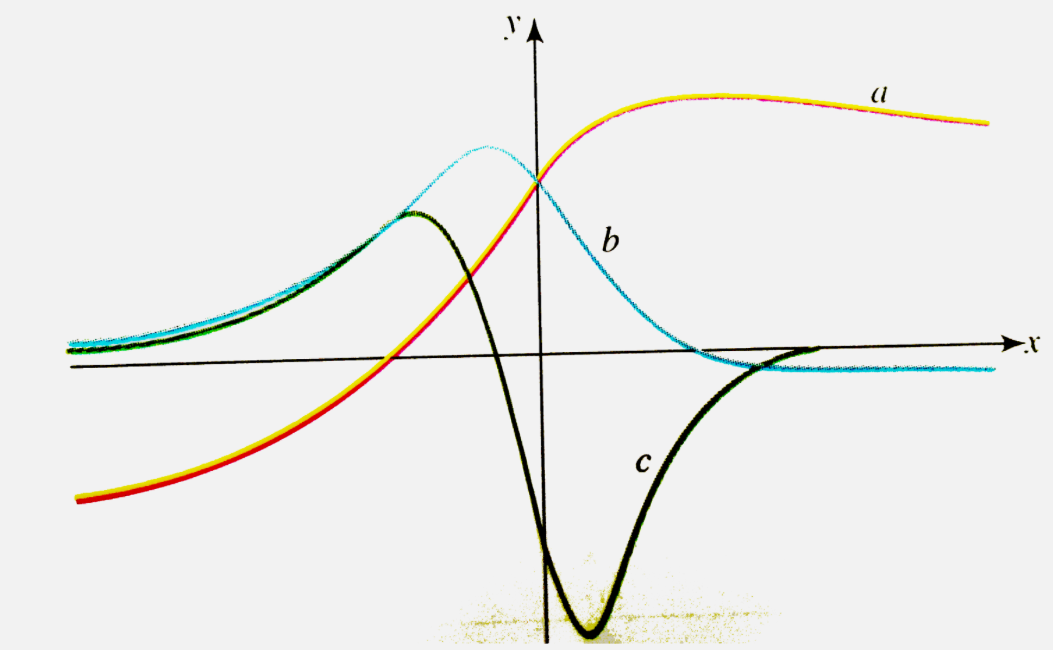 05:06View Solution
05:06View SolutionMatch the graph of y = f(x) in Column I with the corresponding graph of y = f'(x) in Column II.
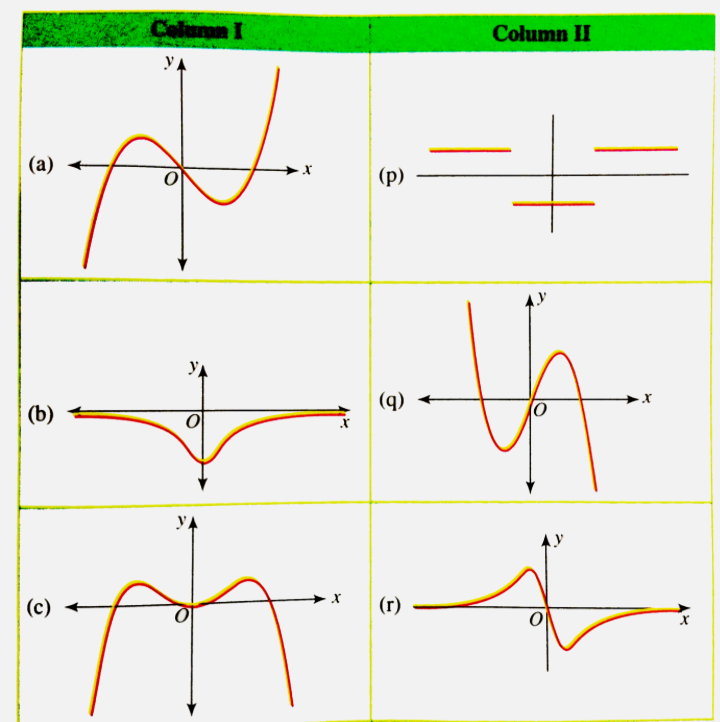
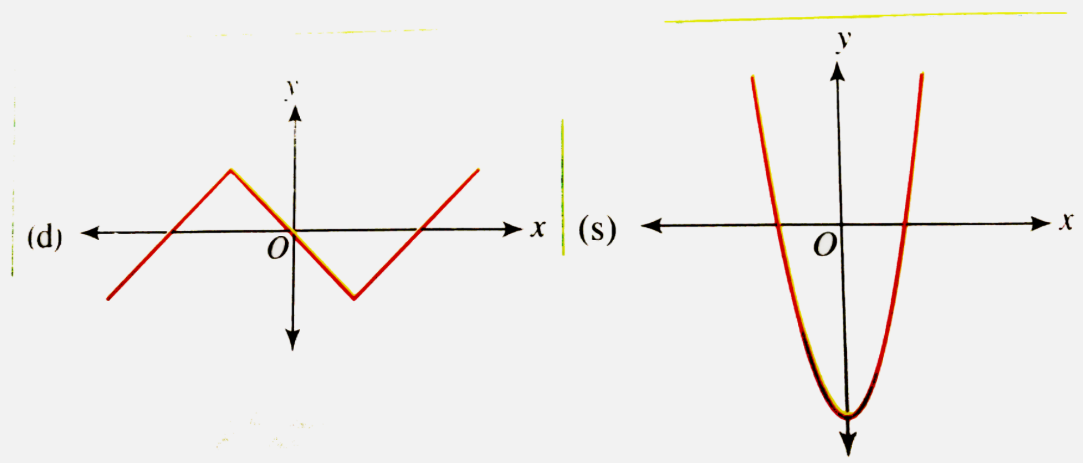 05:06View Solution
05:06View SolutionIf `f(x)` is an even function, then `f'(x)`. If in a `△ABC, atan A+ btan B=(a+b) tan((A+B)/2)` then
05:44View Solution
Exercise questions and complete solutions for chapter GETTING STARTED WITH GRAPHS of CENGAGE of Class 12
