Complete Solutions to Single correct Answer of chapter PERMUTATION AND COMBINATION of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PERMUTATION AND COMBINATION |
| CENGAGE NEW DPP | Single correct Answer |
| Number Of Questions | 61 |
| Academic Year | 2023-24 |
`116` people participated in a knockout tennis tournament. The players are paired up in the first round, the winners of the first round are paired up in the second round, and so on till the final is played between two players. If after any round, there is odd number of players, one player is given a by, i.e. he skips that round and plays the next round with the winners. The total number of matches played in the tournment is
02:25View SolutionThe number of three-digit numbers having only two consecutive digits identical is
02:32View SolutionHow many six-digit numbers are there in which no digit is repeated, even digits appear at even places, odd digits appear at odd places and the number is divisible by `4` ?
04:34View SolutionThe number of ordered pairs `(m,n)` where `m`, `n in {1,2,3,…,50}`, such that `6^(m)+9^(n)` is a multiple of `5` is
06:21View SolutionThere are `10` different books in a shelf. The number of ways in which three books can be selected so that exactly two of them are consecutive is
05:33View SolutionThe number of ways of arranging `6` players to throw the cricket ball so that oldest player may not throw first is
03:27View SolutionNumber of four digit positive integers if the product of their digits is divisible by `3` is.
04:58View SolutionThe number of five-digit numbers which are divisible by `3` that can be formed by using the digits `1,2,3,4,5,6,7,8` and `9`, when repetition of digits is allowed, is
03:05View SolutionIf N is the number of positive integral solutions of `x_1x_2x_3x_4 = 770`, then N =
03:09View SolutionI have tied my square bathroom wall with congruent square tiles. All the tiles are red, except those along the two diagonals, which are all blue. If `I` used `121` blue tiles, then the number of red tiles `I` used are
04:53View SolutionThe number of ordered pairs of positive integers `(m,n)` satisfying `m le 2n le 60`, `n le 2m le 60` is
View SolutionNumber of ways in which `6` distinct objects can be kept into two identical boxes so that no box remains empty is
04:21View SolutionThe number of four-digit numbers that can be formed by using the digits `1,2,3,4,5,6,7,8` and `9` such that the least digit used is `4`, when repetition of digits is allowed is
07:04View SolutionA fair coin is tossed `n` times. Let `a_(n)` denotes the number of cases in which no two heads occur consecutively. Then which of the following is not true ?
View SolutionFive oys and three girls are sitting in a row of `8` seats. Number of ways in which they can be seated so that not all the girls sit side by side is
03:35View SolutionNumber of words that can be made with the letters of the word `"GENIUS"` if each word neither begins with `G` nor ends in `S` is
03:17View SolutionThe number of ways in which the letters of the word PESSIMISTIC can be arranged so that no two S's are together, no of two I's are together and letters `S` and `I` are never together is
04:21View SolutionThe number of different words that can be formed using all the letters of the word 'SHASHANK' such that in any word the vowels are separated by atleast two consonants, is
06:26View SolutionThe number of ways in which six boys and six girls can be seated at a round table so that no two girls sit together and two particular girls do not sit next to a particular boy is
03:49View SolutionSix cards are drawn one by one from a set of unlimited number of cards, each card is marked with numbers `-1, `0` or `1`. Number of different ways in which they can be drawn if the sum of the numbers shown by them vanishes is
04:43View SolutionThe number of positive six-digit integers which are divisible by `9` and four of its digits are `1`, `0`, `0`, `5` is
View SolutionNumber of nine-lettered word that can be formed using all the letters of the word 'MEENANSHU' if alike letters are never adjacent is
06:05View SolutionNumber of ways in which the letters of the word 'ABBCABBC' can be arranged such that the word ABBC does not appear is any word is
06:14View SolutionThe number of `4` digit natural numbers such that the product of their digits is `12` is
03:06View SolutionA class has tree teachers, Mr. `X`, Ms.`Y` and Mrs.`Z` and six students `A`, B`, `C`, `D`, `E`, `F`. Number of ways in which they can be seated in a line of `9` chairs,if between any two teachers there are exactly two students is
03:04View SolutionThe number of words that can be formed using all the letters of the word REGULATIONS such that `G` must come after `R`, `L` must come after `A`, and `S` must come after `N` are
03:16View SolutionThe number of permutation of all the letters of the word `PERMUTATION` such that any two consecutive letters in the arrangement are neither both vowels nor both identical is
05:23View SolutionA guard of `12` men is formed from a group of `n` soldiers. It is found that `2` particular soldiers `A` and `B` are `3` times as often together on guard as `3` particular soldiers `C, D` & `E`. Then `n` is equal to
03:53View SolutionThere are 10 stations on a circular path. A train has to stop at 3 stations such that no two stations are adjacent. The number of such selections must be: (A) `50` (B) `84` (C) `126` (D) `70`
03:06View SolutionFind the number of ways of arranging 15 students `A_1,A_2,........A_15` in a row such that (i) `A_2`, must be seated after `A_1 and A_2`, must come after `A_2` (ii) neither `A_2` nor `A_3` seated brfore `A_1`
03:52View SolutionThere are 15 different apples and 10 different pears. How many ways are apple or a pear and then Jill to pick an apple and a pear?
02:11View SolutionThere are `12` pairs of shoes in a box. Then the possible number of ways of picking `7` shoes so that there are exactly two pairs of shoes are
04:13View SolutionThere are two sets of parallel lines, their equations being `x cos alpha+y sin alpha=p` and `x sin alpha- y cos alpha=p` , `p=1,2,3,….n` and `alpha in (0,pi//2)`. If the number of rectangles formed by these two sets of lines is `225`, then the value of `n` is equals to
View SolutionThe number of rectangles that can be obtained by joining four of the twelves verties of a 12-sides regular polygon is -
01:47View SolutionThe interior angles of a regular polygon measure `150^@` each. The number of diagonals of the polygon is
02:47View SolutionNumber of ways in which 7 green bottles and 8 blue bottles can be arranged in a row if exactly 1 pair of green bottles is side by side, is (Assume all bottles to be alike except for the colour).
05:22View SolutionWhich of the following is noth the number of ways of selecting `n` objects from `2n` objects of which `n` objects are identical
View SolutionFind number of seven-digit number in the form of `a b c d efg(g,f,e ,` tc. Are digits at units, tens hundreds place etc.) `where a<< b<< c<< d>>e>>f>>g dot`
04:28View SolutionNumber of six-digit numbers such that any digit that appears in the number appears at least twice, where the digits of each number are from the set `{1, 2, 3, 4, 5},` is (Example 225252 is valid but 222133 is not valid)
04:35View SolutionAll the five digit numbers in which each successive digit exceeds its predecessor are arranged in the increasing order of their magnitude. The `97^(th)` number in the list does not contain the digit
03:59View SolutionThe number of `n` digit number formed by using digits `{1,2,3}` such that if `1` appears, it appears even number of times, is
View Solution`A,B,C,D` develop `18` items. Five items jointly by `A` and `C`, four items by `A` and `D`, four items by `B` and `C` and five items by `B` and `D`. The number of ways of selecting eight ites out of `18` so that the selected ones belong equally to `A,B,C,D` is
02:49View SolutionThe number of pairs of diagonals of a regular polygon of `10` sides that are parallel are
07:09View SolutionFour letters, two `'a'` and two `'b'` are filled into `16` cells of a matrix as given. It is required that each cell contains atmost one letter and each row or column cannot contain same letters. Then the number of ways the matrix can be filled is
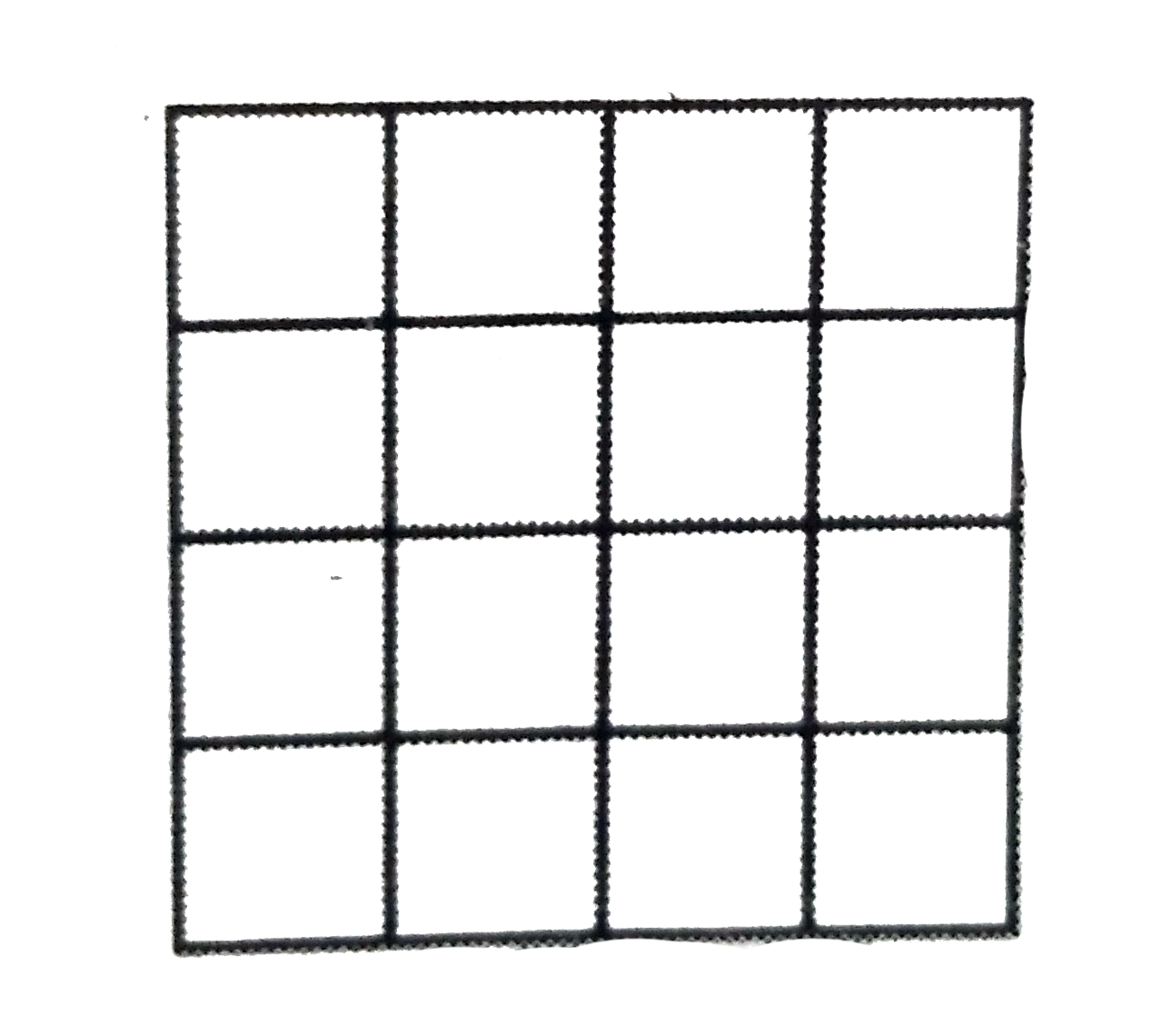 View Solution
View SolutionThe number of increasing function from `f : AtoB` where `A in {a_(1),a_(2),a_(3),a_(4),a_(5),a_(6)}`, `B in {1,2,3,….,9}` such that `a_(i+1) gt a_(i) AA I in N` and `a_(i) ne i` is
04:42View SolutionHow many ordered pairs of (m,n) integers satisfy `(m)/(12)=(12)/(n)`?
01:02View SolutionProduct of all the even divisors of `N = 1000`, is
02:48View SolutionHow many combinations can be made up of `3` hens, `4` ducks and `2` geese so that each combination has hens, ducks and geese ?
03:32View SolutionA positive integer `n` is of the form `n=2^(alpha)3^(beta)`, where `alpha ge 1`, `beta ge 1`. If `n` has `12` positive divisors and `2n` has `15` positive divisors, then the number of positive divisors of `3n ` is
04:03View SolutionNumber of permutations of `1,2,3,4,5,6,7,8`, and `9` taken all at a time are such that digit `1` appearing somewhere to the left of `2` and digit `3` appearing to the left of `4` and digit `5` somewhere to the left of `6`, is
(e.g. `815723946` would be one such permutation)03:04View SolutionThe number of arrangments of all digits of `12345` such that at least `3` digits will not come in its position is
05:50View SolutionSixteen players `S_(1)`, `S_(2)`, `S_(3)`,…,`S_(16)` play in a tournament. Number of ways in which they can be grouped into eight pairs so that `S_(1)` and `S_(2)` are in different groups, is equal to
03:22View SolutionThe number of homogenous products of degree `3` from `4` variables is equal to
01:50View SolutionThe number of ways of distributing `3` identical physics books and `3` identical methematics books among three students such that each student gets at least one books is
04:25View SolutionFour different movies are running in a town. Ten students go to watch these four movies. The number of ways in which every movie is watched by atleast one student, is (Assume each way differs only by number of students watching a movie)
02:38View SolutionTen identical balls are distributed in 5 different boxes kept in a row and labeled `A, B, C, D and E.` The number of ways in which the ball can be distributed in the boxes if no two adjacent boxes remains empty
09:38View Solution`5` different objects are to be distributed among `3` persons such that no two persons get the same number of objects. Number of ways this can be done is,
03:09View SolutionFind number of negative integral solution of equation `x + y + z = - 12`
03:43View SolutionThe number of ways can five people be divided into three groups is
02:07View SolutionThe number of ways of partitioning the set `{a,b,c,d}` into one or more non empty subsets is
02:37View SolutionLet `y` be an element of the set `A={1,2,3,4,5,6,10,15,30}` and `x_(1)`, `x_(2)`, `x_(3)` be integers such that `x_(1)x_(2)x_(3)=y`, then the number of positive integral solutions of `x_(1)x_(2)x_(3)=y` is
02:55View Solution
Single correct Answer questions and complete solutions for chapter PERMUTATION AND COMBINATION of CENGAGE MATHS (HINGLISH) of Class 12
