T is a parallelopiped in which A B C and D are vertices of one face and the face just above it has corresponding vertices A B C D T is now compressed to S with face ABCD remaining same and A B C D shifted to A B C D in S. The volume of parallelopiped S is reduced to 90% of T. Prove that locus of A is a plane.
Let the equation of the plane ABCD be ax + by + cz + d = 0 the point A be α β γ and the height of the parallelopiped ABCD be h... Locus of A is a plane parallel to the plane ABCD.
Related Solutions
T is a parallelepiped in which A,B,C and D are vertices of one face and the face just above it has corresponding vertices A’,B’,C’,D’,T is now compressed to S with face ABCD remaining same and shifted to A”,B”,C”,D”, in S. The volume of parallelepiped S is reduced to 90% of T. Prove that locus of is a plane.
View SolutionT एक समान्तर षट्फलक है, जिसमें A, B, C व D एक फलक के शीर्ष है और उसके ठीक ऊपर वाली फलक के शीर्ष A', B', C' व D' है। T को S तक इस प्रकार दबाया जाता है कि फलक ABCD समान रहती है और A', B', C' व D', S में A'', B'', C'' व D'' में परिवर्तिति हो जाती है। समान्तर षट्फलक S का आयतन, T का 90 % रह जाता है A'' का बिन्दुपथ क्या होगा ?
View SolutionT एक समान्तर षटफलक है जिसमें एक फलक के शीर्ष A, B, C वD है। तथा इससे ठीक ऊपर के फलक के शीर्ष क्रमशः 'A', B, C, D' है। T को S तक इस प्रकार दबाया जाता है कि फलक ABCD समान रहे, तथा A', B, C, D', S क्रमशः A", B", C" व D" में स्थानान्तरित हो जाते है । का आयतन, T के आयतन का 90% कम हो जाता है। सिद्ध कीजिए कि "A" का बिन्दुपथ एक समतल होगा।
View SolutionT is a parallel hexagon, in which A, B, C and D are at the top of a pane and the top of the pane immediately above it is A ', B', C 'and D'. T is pressed to S in such a way that ABCD remains same and converts to A ', B', C 'and D', S to A '', B '', C '' and D ''. . The volume of parallel hexagon S remains 90% of T. What will be the locus of A ''?
View SolutionLet A,B,C,D are (not necessarily square) real matrices such that A^(T) = BCD , B^(T) = CDA, C^(T) = DAB and D^(T) = ABC for the matrix S = ABCD prove that S^(3) = S .
View SolutionLet be (not necessarily ) real matrices such that for the matrix the least value of k such that is
View SolutionLet be (not necessarily ) real matrices such that for the matrix the least value of k such that is
View SolutionLet be (not necessarily ) real matrices such that for the matrix the least value of k such that is
View SolutionLet be (not necessarily ) real matrices such that for the matrix the least value of k such that is
View SolutionA, B, C and D are playing carom C, A and D, B are partners. D is to the right of C, C is facing west. Then, B is facing which direction ?
View SolutionA parallelepiped S has base points A ,B ,Ca n dD and upper face points A^(prime),B^(prime),C^(prime),a n dD ' . The parallelepiped is compressed by upper face A ' B ' C ' D ' to form a new parallepiped T having upper face points A^(prime prime),B^(prime prime),C^(prime prime) and D^(prime prime) . The volume of parallelepiped T is 90 percent of the volume of parallelepiped Sdot Prove that the locus of A^(prime prime) is a plane.
View SolutionA parallelepiped has base points and upper face points . The parallelepiped is compressed by upper face to form a new parallepiped having upper face points and . The volume of parallelepiped is 90 percent of the volume of parallelepiped Prove that the locus of is a plane.
View SolutionA, B,C and D are playing cards. A and C, and B and D are partners. D is to the right of C. The face of C is towards West. Find the direction that D is facing.
View SolutionA parallelepiped has base points and upper face points . The parallelepiped is compressed by upper face to form a new parallepiped having upper face points . The volume of parallelepiped is 90 percent of the volume of parallelepiped Prove that the locus of is a plane.
View SolutionA parallelepiped has base points and upper face points . The parallelepiped is compressed by upper face to form a new parallepiped having upper face points . The volume of parallelepiped is 90 percent of the volume of parallelepiped Prove that the locus of is a plane.
View SolutionA parallelepiped has base points and upper face points . The parallelepiped is compressed by upper face to form a new parallepiped having upper face points . The volume of parallelepiped is 90 percent of the volume of parallelepiped Prove that the locus of is a plane.
View SolutionA, B, C and D are playing a game of carrom. A, C and B, D are partners. C is to the left of D who is facing South. Then A is facing
View SolutionMatch te columns
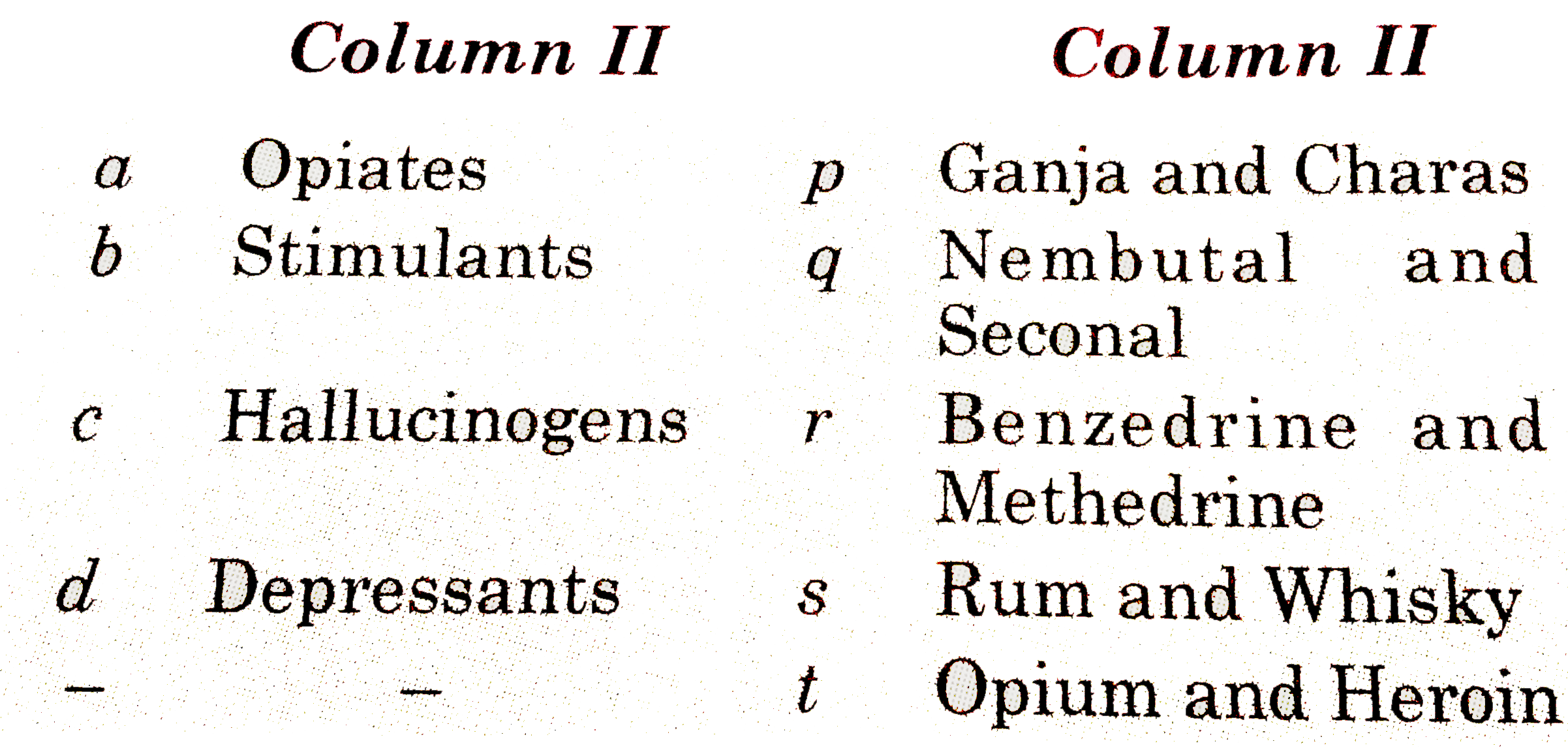
(1) a-s, b-t, c-r, d-p
(2) a-t, b-q, c-r, d-p
(3) a-t, b-r, c-q, d-p
(4) a-t, b-r, c-p, d-qView SolutionMatch the columns

(1) a-s, b-t, c-r, d-p
(2) a-t, b-q, c-r, d-p
(3) a-t, b-r, c-q, d-p
(4) a-t, b-r, c-p, d-qView SolutionLet S be the set of 2xx2 matrices given by S={A=[[a,b],[c,d]],"where" a,b,c,d,in I} , such that A^(T)=A^(-1) Then
View Solution
