If a+b is along the angle bisector of a and b, where , then the number of digits in value of is
The correct Answer is:1
Since, angle bisector of a and b
. . . (i)
Given, a+b is alongg angle bisector
only, when
.
Topper's Solved these Questions
- BOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Subjective Type Questions)8videos
VECTOR ALGEBRA
- BOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)9videos
VECTOR ALGEBRA
- BOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Matching Type Questions)1videos
VECTOR ALGEBRA
- BOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)19videos
TRIGONOMETRIC FUNCTIONS AND IDENTITIES
Similar Questions
A three digit numbers is equal to the sum of the factorial of their digits . If the sum of all such three digit numbers is λ then find the sum of digit of λ.
View SolutionThe sum of all three digit numbers each of which is equal to 11 times the sum of the squares of its digits is λ . Find the sum of digits of λ
View Solution
Knowledge Check
- Question 1 - Select One
The number of rectangles excluding squares from a rectangle of size 11×8 is 48λ, then the value of λ is
A13B23C43D53 - Question 2 - Select One
Find the value of ∠A. If BD is an interior angle bisector of ∠B and DC is an exterior angle bisector of ∠C.
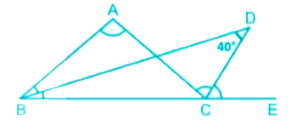 A70∘B60∘C80∘D110∘
A70∘B60∘C80∘D110∘ - Question 3 - Select One
If a,b and c are the sides of a traiangle such that b.c=λ2, then the relation is a,λandA is
Ac≥2λsin(C2)Bb≥2λsin(A2)Ca≥2λsin(A2)DNone of these
The thermal emissivities of two bodies A and B are 0.02 and 0.09, respectively. λA and λB are the wavelengths corresponding to maximum intensity of radiations and λA is shifted from λB by μm . If the surface area and the total power radiated by the bodies are same, then calculate the temperature of B, given temperature of A is 5000 K.
View SolutionA line x=λ intersects the graph of y=log5x and y=log5(x+4).The distance between the point of intersection is 0.5. Given λ=a+√b,where a and b are integers , the value of (a+b) is
View SolutionA radioactive element A of decay constant λA decays into another radioactive element B of decay constant λB. Initially the number of active nuclei of A was N0 and B was absent in the sample. The maximum number of active nuclei of B is found at t=2. In 2/λA. The maximum number of active nuclei of B is
View SolutionRadioactive material 'A' has decay constant '8λ' and material 'B' has decay constant 'lamda'. Initial they have same number of nuclei. After what time, the ratio of number of nuclei of material 'B' to that 'A' will be 1e ?
View SolutionIf a and b are two positive quantities whose sum is λ then the minimum value of √(1+1a)(1+1b) is
View Solution

