$f (x) = | a x - b | + c | x | \forall x \in (- \infty, \infty),$ where $a > 0, b > 0, c > 0 .$ Find the condition if $f (x)$ attains the minimum value only at one point.

Video Solution

Text Solution

Verified by Experts

$f (x) = ⎧ ⎪ ⎪ ⎨ ⎪ ⎪ ⎩ \begin{matrix} b - a x - c x, & x < 0 b - a x + c x, & 0 \leq x < \frac{b}{a} a x - b + c x, & x \geq \frac{b}{a} \end{matrix}$
$= ⎧ ⎪ ⎪ ⎨ ⎪ ⎪ ⎩ \begin{matrix} b - (a + c) x, & x < 0 b + (c - a) x, & 0 \leq x < \frac{b}{a} (a + c) x - b, & x \geq \frac{b}{a} \end{matrix}$
The graphs of the function for different situations are as shown in the following figures.

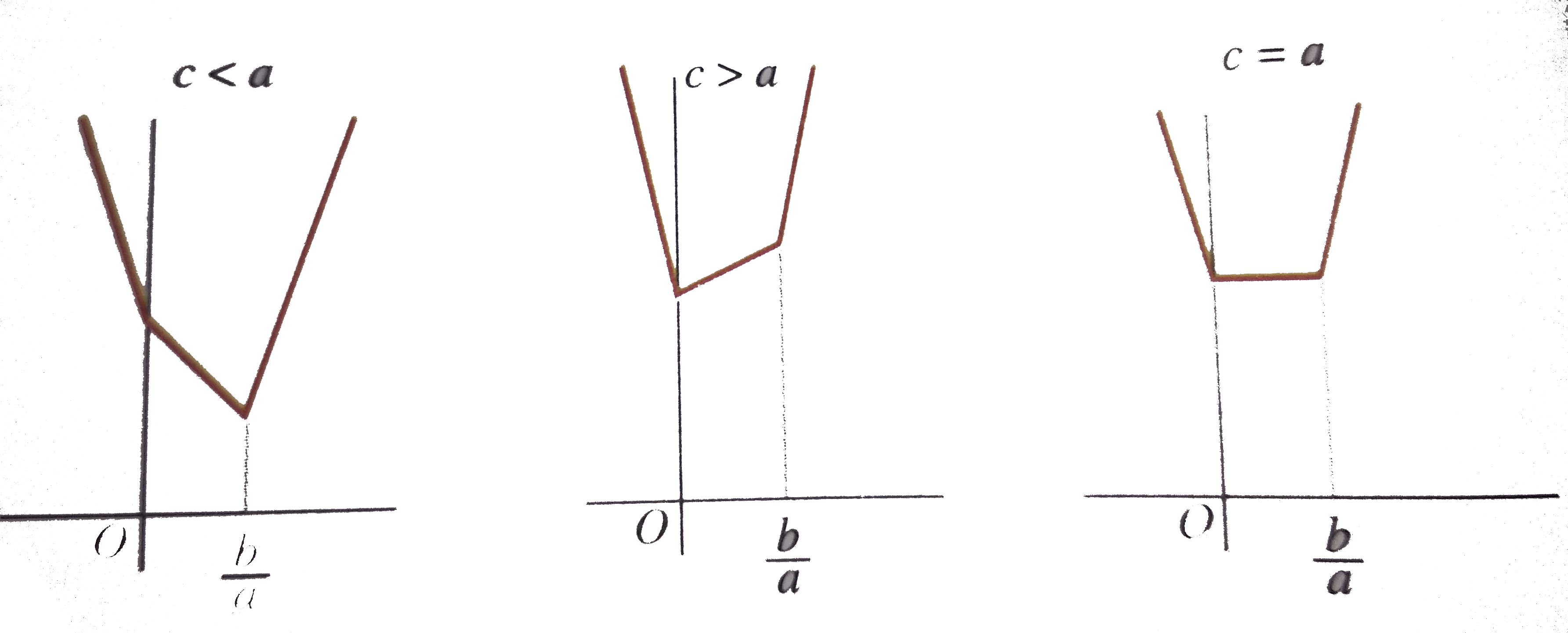
From the figure, we can see that for $c < a and c > a$ , there is only point of minima.
For $c = a$ , there are infinite points of minima.
Thus, for exactly one point of minima, $c \neq a$ .

Updated on:21/07/2023

Let $f (x) < 0 \forall x \in (- \infty, 0) and f (x) > 0 \forall x \in (0, \infty)$ also $f (0) = 0,$ Again $f' (x) < 0 \forall x \in (- \infty, - 1) and f' (x) > \forall x \in (- 1, \infty)$ also $f' (- 1) = 0$ given $lim x \to \infty f (x) = 0 and lim x \to \infty f (x) = \infty$ and function is twice differentiable.
The minimum number of points where $f' (x)$ is zero is:
A1
B2
C3
D4
The value of b for which the function f(x)=sin x-bx+c is decreasing in the interval $(- \infty, \infty)$ is given by
A $b < 1$
B $b \geq 1$
C $b > 1$
D $b \leq 1$