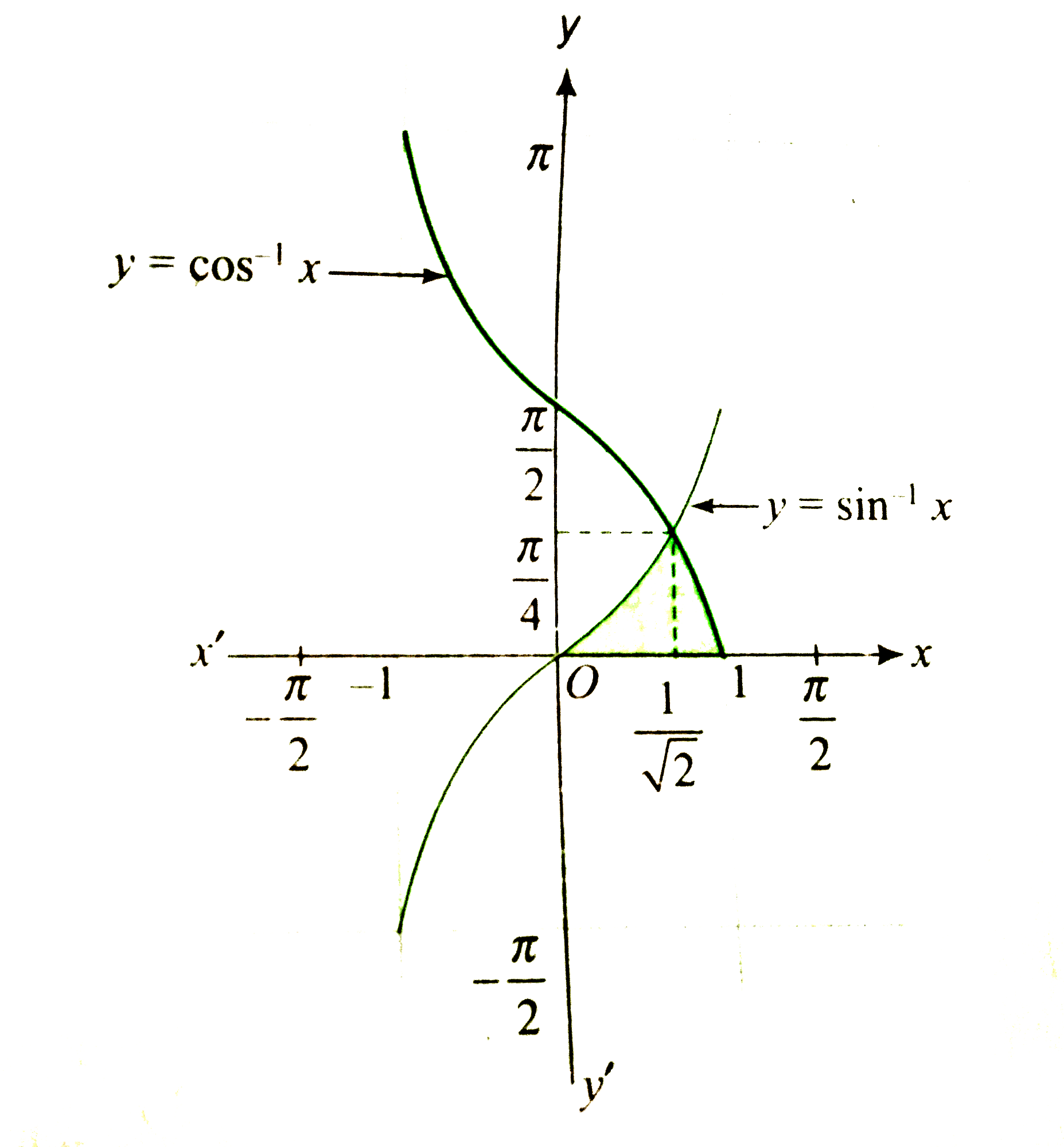
The area bounded by $y = {sin}^{- 1} x, y = {cos}^{- 1} x$ and the x-axis, is given by

Video Solution

Text Solution

Verified by Experts

We have to find the area bounded by $y = {sin}^{- 1} x, y = {cos}^{- 1} x,$ and the x-axis. If integration along x-axis is used then
$A = 1 / \sqrt{2} \int 0 {sin}^{- 1} x d x + 1 \int 1 \sqrt{2} {cos}^{- 1} x d x$
If integration along y-axis is used, then
$A = π / 4 \int 0 (cos y - sin y) d y$
$= {[sin y + cos y]}_{0}^{π / 4}$
$= [\frac{1}{\sqrt{2}} + \frac{1}{\sqrt{2}} - 1]$
$= \sqrt{2} - 1$

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Area bounded by $y = {tan}^{- 1} x, y = {cot}^{- 1} x$ and y-axis is equal to
Aln $\sqrt{2}$ sq. unit
Bln 4 sq. unit
Cln 8 sq. unit
Dln 2 sq. unit
Question 2 - Select One
The area bounded by $y = {sin}^{- 1} x, x = \frac{1}{\sqrt{2}}$ and X-axis is
A $(\frac{1}{\sqrt{2}} + 1)$ sq units
B $(1 - \frac{1}{\sqrt{2}})$ sq units
C $\frac{π}{4 \sqrt{2}}$ sq units
D $(\frac{π}{4 \sqrt{2}} + \frac{1}{\sqrt{2}} - 1)$ sq units
Question 3 - Select One
The area bounded by $y = {sin}^{- 1} x$ , y-axis and $| y | = \frac{π}{2}$ is
A2
B $π$
C $2 π$
D1

CENGAGE-AREA-Comprehension Type

Home
Ask Doubt
Profile