The area common to regions $x^{2} + y^{2} - 2 x \leq 0$ and $y \geq \frac{sin (π x)}{2} .$

Video Solution

Text Solution

Verified by Experts

$For x^{2} + y^{2} - 2 x \leq 0$
points lie on or inside circle ${(x - 1)}^{2} + y^{2} = 1$
$For y \geq sin \frac{π x}{2},$ points lie on or above $y = sin \frac{π x}{2} .$
$y = sin \frac{π x}{2}$ has period 4.
The graphs of curves and the required region is as shown in teh follwoing figure

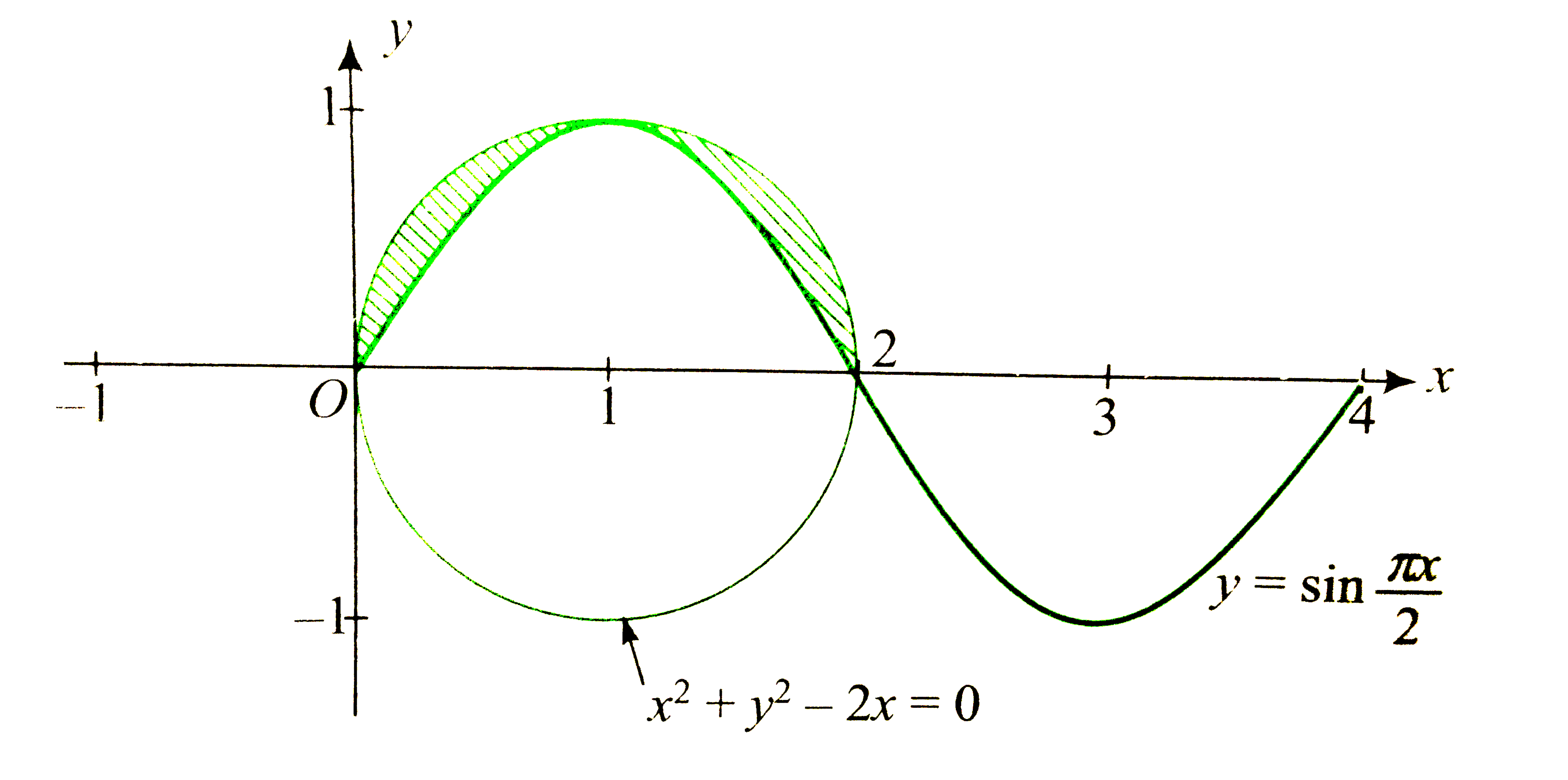
For the figure, required area = area of semicircle $- 2 \int 0 sin \frac{π x}{2} d x$
$= \frac{π}{2} + \frac{2}{π} {[cos \frac{π x}{2}]}_{0}^{2}$
$= \frac{π}{2} - \frac{4}{π}$

Updated on:21/07/2023