Plot the region in the first quadrant in which points are nearer to the origin than to the line $x = 3 .$

Video Solution

Text Solution

Verified by Experts

Point P(x,y) lies in the first quadrant. Therefore, $x, y > 0$

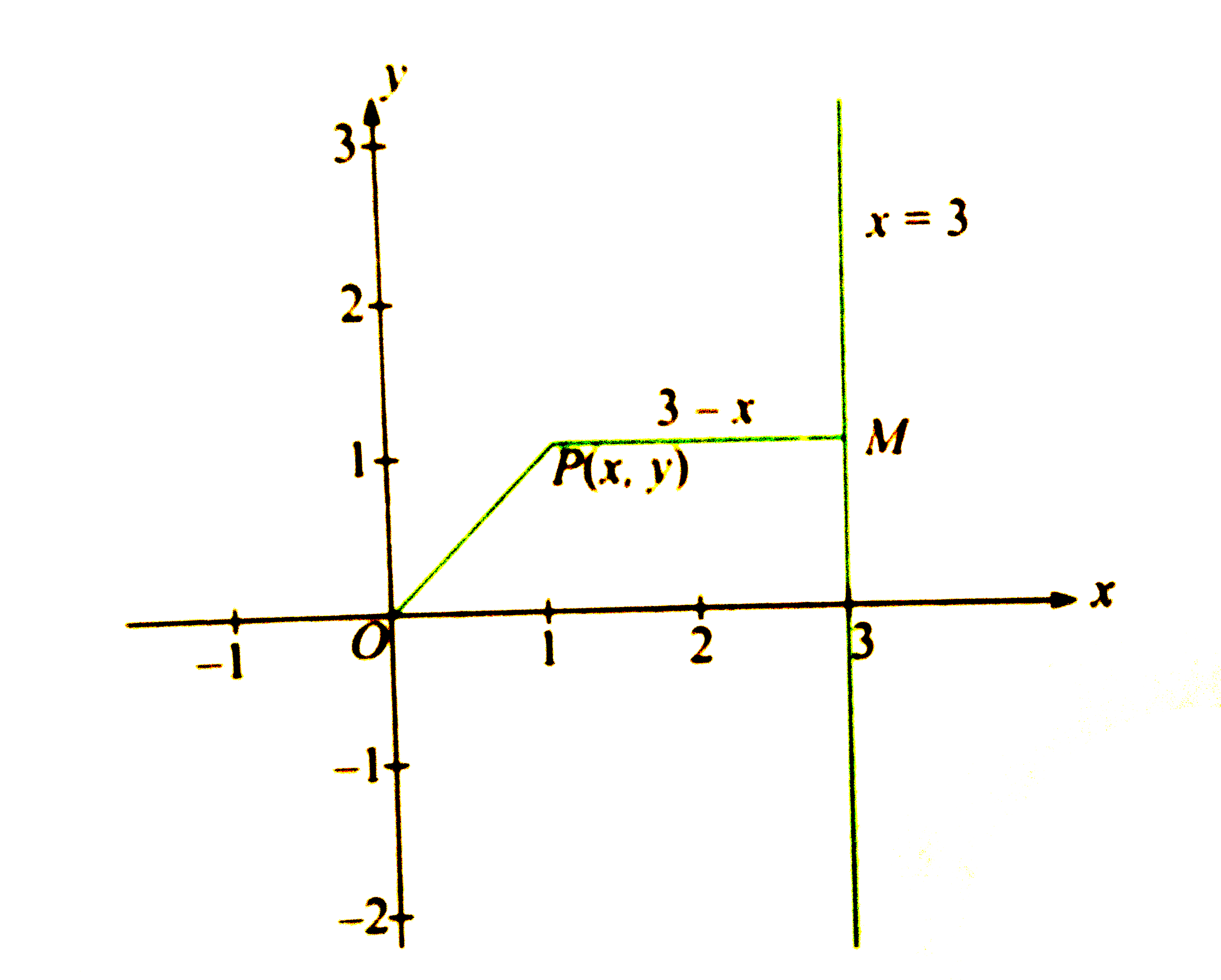
Also point P(x,y) is nearer to the origin than to the line x=3
$Now O P = \sqrt{x^{2} + y^{2}}$
Distance of P from line x-3=0 is PM =3-x
According to the question, $O P < P M$
$\Rightarrow \sqrt{x^{2} + y^{2}} < (3 - x)$
$or x^{2} + y^{2} < x^{2} - 6 x + 9$
$or y^{2} < - 6 x + 9$
$or y^{2} < - 6 (x - \frac{3}{2})$
Points satisfying above inequality lie inside parabola $y^{2} = 9 - 6 x$ in the first quadrant.
$Parabola y^{2} = - 6 (x - \frac{3}{2})$ is concave to the left, having axis as
x-axis and vertex at $(\frac{3}{2}, 0)$ and directrix x=3
The required region as shown in the figure.

$Area of the region = 3 \int 0 \frac{9 - y^{2}}{6} d y = \frac{1}{6} {(9 y - \frac{y^{3}}{3})}_{0}^{3}$
$= \frac{1}{6} (27 - 9) = 3$ sq. units

Updated on:21/07/2023