Two concentric circular coils, one of small radius r1 and the other of large radius r2, such that r1<<r2, are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
 Solution in Gujrati
Solution in Gujrati
Let a current I2 flow through the outer circular coil. The field at the centre of the coil is B2=μ0I2/2r2 . Since the other co-axially placed coil has a very small radius, B2 may be considered constant over its cross-sectional area. Hence,
Φ1=πr21B2
=μ0πr212r2I2
M12I2
Thus,
M12=miu0πr212r2
From Eq. (6.14)
M12=M21=μ0πr212r2
Note that we calculated M12 from an approximate value of Φ1
, assuming the magnetic field B2 to be uniform over the area πr21. However, we can accept this value because r1<<r2.
Topper's Solved these Questions
- BOOK - NCERT GUJARATICHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISES10videos
ELECTROMAGNETIC INDUCTION
- BOOK - NCERT GUJARATICHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ADDITIONAL EXERCISES7videos
ELECTROMAGNETIC INDUCTION
- BOOK - NCERT GUJARATICHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - EXERCISES34videos
ELECTRIC CHARGES AND FIELDS
- BOOK - NCERT GUJARATICHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ADDITIONAL EXERCISES5videos
ELECTROMAGNETIC WAVES
Similar Questions
Predict the direction of induced current in the situations described by the following figures (a) to (d).
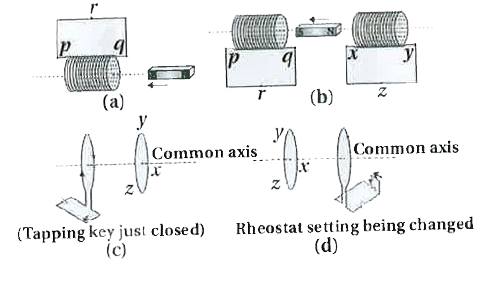 03:01View Solution
03:01View SolutionSuppose the loop is stationary but the current feeding the electromagnet that produces the magnetic field is gradually reduced so that the field decreases from its initial value of 0.3 T at the rate of 0.02 T s−1. If the cut is joined and the loop has a resistance of 1.6 Ω, how much power is dissipated by the loop as heat ? What is the source of this power ?
01:43View SolutionWhat is the force between two small charged speres having charges of 2×10−7 c and 3×10−7 c placed 30 cm apart in air
View SolutionSuppose that the electric field part of an electromagnetic wave in vacuum is →E={(3.1N/C)cos[(1.8rad/m)y+(5.4×106rad/s)]}ˆi
What is the direction of propagation ?02:38View SolutionTwo circular coils, one of smaller radius r1 and the other of very large radius r2 are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
04:02View SolutionTwo concentric circular coil,s one of small radius r1 and the other of large radius r2, such that r1<<r2, are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
02:43View SolutionIn a △ABC if r1<r2<r3, then
07:32View SolutionIf r1andr2 represent radii of two concentric coil (r2>>r1) then give the expression for the coefficient of mutual inductance for the pair of coils.
05:17View SolutionWhen two soap bubbles of radii r_1 and r_2 (r_2 lt r_1) adjoin, the radius of curvature of the common surface is
05:37View SolutionTwo concentric circular coils, one of small radius r1 and the other of large radius r2, such that r1<<r2, are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
01:43View SolutionTwo concentric circular coils, one of small radius r1 and the other of large radius r2, such that r1<<r2, are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
View SolutionTwo circular coils, one of smaller radius r_(1) and the other of very large radius r_(2) are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
View SolutionTwo concentric circular coils, one of small radius r1 and the other of large radius r2, such that r1<<r2, are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
 04:49View Solution
04:49View SolutionTwo concentric circular coil,s one of small radius r1 and the other of large radius r2, such that r1<<r2, are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
View SolutionTwo concentric circular coils, one of small radius r, and the other of large radius r2, such that r1<<r2 , are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
View SolutionTwo concentric spherical shells have masses M1,M2 and radii R1,R2(R1<R2). The force exerted by this system on a particle of mass m. If it is placed at a distance (R1+R22) from the centre.
03:38View Solution

