The P-V curves in two cases are shown as a smaller circle x and a bigger circle y, in the figure, Indicate whether the net work done is positive or negative.
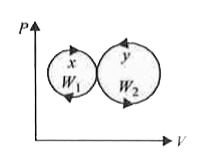
The cyclic process x is clockwise. Hence the work done W1 is positive. The cyclic process y is anticlockwise. Hence the work done W2 is negative.
∵ Area of y> Area of x
∴W2>W1
∴ Net work done will be negative.
Topper's Solved these Questions
- BOOK - MTG GUIDECHAPTER - THERMODYNAMICSEXERCISE - NEET Cafe (Topicwise practice Questions)80videos
THERMODYNAMICS
- BOOK - MTG GUIDECHAPTER - THERMODYNAMICSEXERCISE - NEET (ITALS)25videos
THERMODYNAMICS
- BOOK - MTG GUIDECHAPTER - PROPERTIES OF BULK MATTEREXERCISE - AIPMT/NEET (MCQs)40videos
PROPERTIES OF BULK MATTER
- BOOK - MTG GUIDECHAPTER - WORK, ENERGY AND POWEREXERCISE - AIPMT/NEET (MCQs)34videos
WORK, ENERGY AND POWER
Similar Questions
Knowledge Check
- Question 1 - Select One
In the figure below, the radius of the bigger circle is (√2+1) cm and the radius of all the smaller circles are equal. Each of the smaller circles touches two of the other three smaller circles and the larger circle as shown. Find the area (in cm2) of the shaded portion.
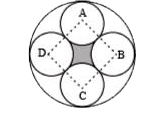 A2−π2B1Cπ/4DCannot to determined
A2−π2B1Cπ/4DCannot to determined - Question 2 - Select One
Two moles of helium gas undergo a cyclic process as shown in figure. Assuming the gas to be ideal. The net work done by the gas is
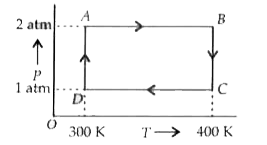 A200 Rln2B100 Rln2C300 Rln2D400 Rln2
A200 Rln2B100 Rln2C300 Rln2D400 Rln2 - Question 3 - Select One
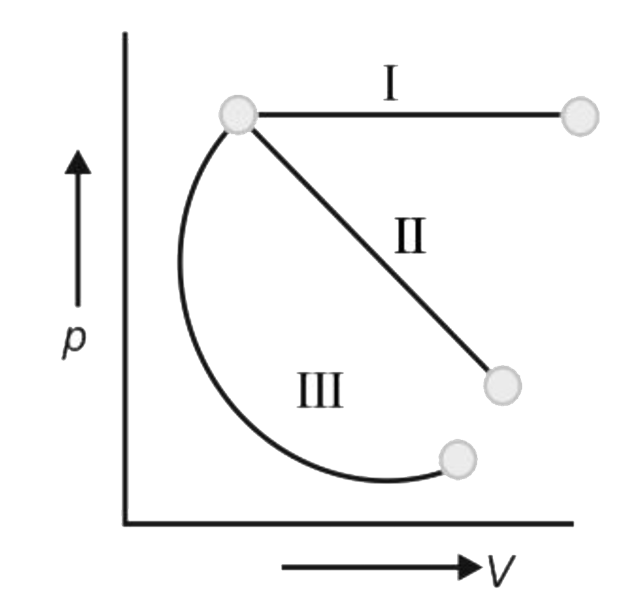
In figure, P−V curve of an ideal gas is given. During the process, the cumulative work done by the gas
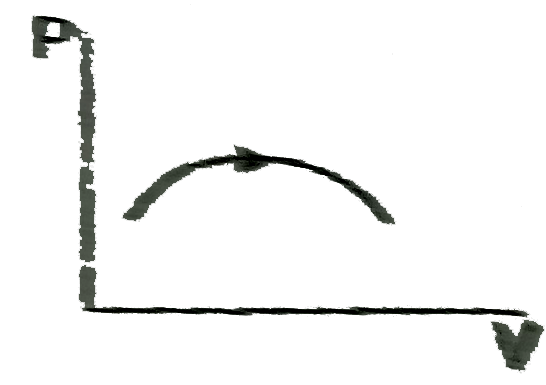 Acontinuously increasesBcontinuously decreasesCfirst increases then decreasesDfirst decreases then increases
Acontinuously increasesBcontinuously decreasesCfirst increases then decreasesDfirst decreases then increases
The figure into which the curve y2=6x divides the circle x2+y2=16 are in the ratio
View SolutionIn a circle with centre O and radius 'r ', another smaller circle is inscribed with centre D and radius half that of the bigger circle as shown in the figure. Tangents to the smaller circle from a point A(A-O-T) on the bigger circle meet at E and F and meet its diameter when produced at B and C. Then, ArΔABC is :
View SolutionIn the figure, two circles intersect each other at points A and B . C is a point on the smaller circle. Secant CA and secant CB of the smaller circle intersect the bigger circle at points D and E respectively then prove : seg DE ∣∣ line CF
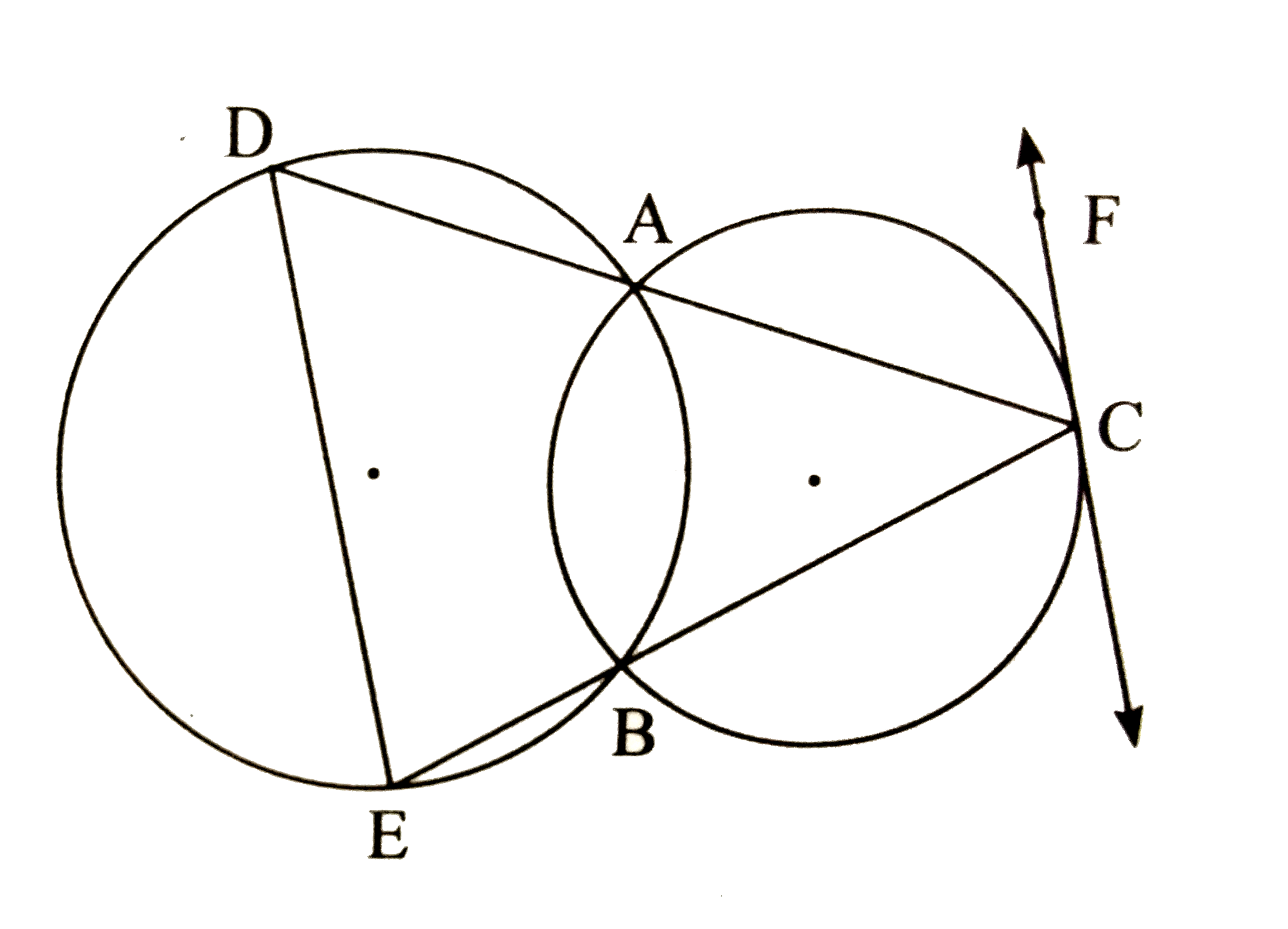 View Solution
View SolutionMake a point A. Taking A as centre draw two circles, one with radius 5 cm and the other with radius 7 cm. Draw line segments AB and AC where AB is a radius of the smaller circle and AC a radius of the bigger circle. Mark points P, Q, R such that point P is inside both the circles, Q is outside the smaller circle but inside the bigger one and point R is outside both the circles.
View SolutionIn figure, P−V curve of an ideal gas is given. During the process, the cumulative work done by the gas
 View Solution
View Solution