A regular hexagon is inscirbed in a circle of radius R . Another circle is inscribed in the hexagon . Now another hexagon is inscribed in the second ( smaller ) circle .
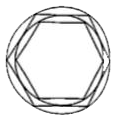
If thre are some more circle and hexagons inscribed in the similar way as given above, then the ratio of each side of outermost hexagon ( largest one ) to that of the fourth ( smaller cone ) hexagon is ( fourth hexagon means the hexagon which is inside the third hexagon from the outside ):
9:3√2
0.67291666666667
8:3√3
none of these
The correct Answer is:C
Step by step video & image solution for A regular hexagon is inscirbed in a circle of radius R . Another circle is inscribed in the hexagon . Now another hexagon is inscribed in the second ( smaller ) circle . If thre are some more circle and hexagons inscribed in the similar way as given above, then the ratio of each side of outermost hexagon ( largest one ) to that of the fourth ( smaller cone ) hexagon is ( fourth hexagon means the hexagon which is inside the third hexagon from the outside ): by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
A regular hexagon is inscirbed in a circle of radius R . Another circle is inscribed in the hexagon . Now another hexagon is inscribed in the second ( smaller ) circle .
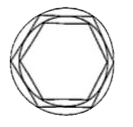
What is the sum of perimeters of both the hexagons ?A(2+√3)RB3(2+√3)RC3(3+√2)RDnone of these - Question 2 - Select One
A regular hexagon is inscirbed in a circle of radius R . Another circle is inscribed in the hexagon . Now another hexagon is inscribed in the second ( smaller ) circle .
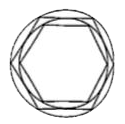
What is the ratio of area of inner circle to the outer circle ?A3:4B9:16C3:8Dnone of these - Question 3 - Select One
If a regular hexagon circumscribes a circle of radius r , then its perimeter is :
Aa)6√3rBb)6rCc)3rDd)12 r
If a regular hexagon circumscribes a circle of radius r , then its perimeter is :
View SolutionIf a regular hexagon circumscribes a circle of radius r , then its perimeter is :
View SolutionIf a regular hexagon circumscribes a circle of radius r , then its perimeter is :
View SolutionArea of regular hexagon with side 'a' is
View SolutionA square is inscribed in circle of radius R, a circle is inscribed in the square, a new square in the circle and so on for n times.
Sum of the areas of all circles isView Solution

