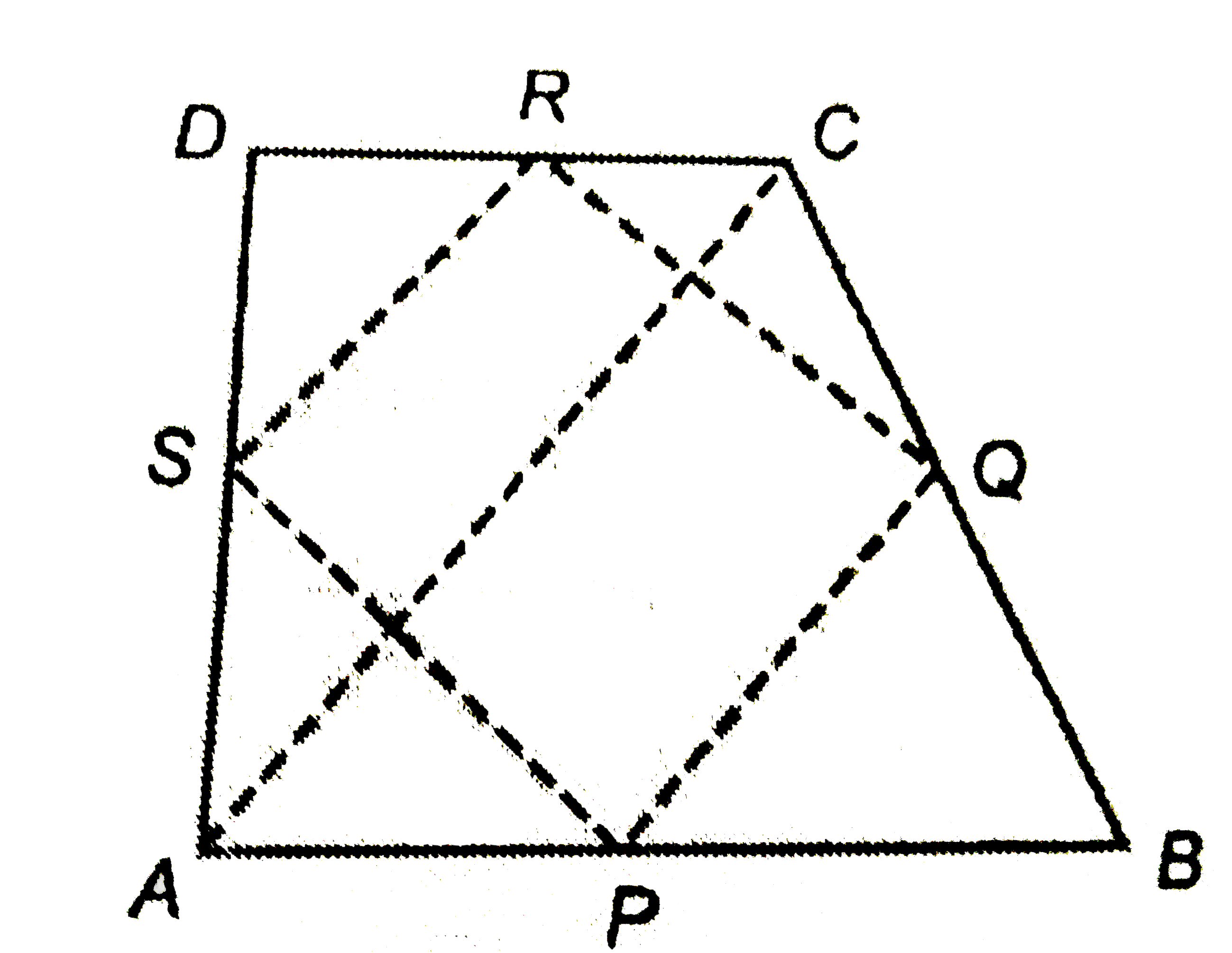
Show that the line segments joining the mid-points of opposite sides of a quadrilateral bisects each other.
Let P, Q R and S are the mid-points of the sides AB, BC, CD and DA respectively of Join AC.,
Since, P and Q are the mid-points of BA and BC respectively.
In
Since, R and S are th mid-points of DC and DA respectively.
From equations (1) and (2)
is a parallelogram
We know that the diagonals of a parallelogram bisect each other.
PR and QS bisect each other.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Problems From NCERT/exemplar11videos
QUADRILATERALS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Exercise 8a29videos
QUADRILATERALS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - PROBABILITYEXERCISE - Revision Exercise (very Short Answer /short Answer Questions)10videos
PROBABILITY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - Revision Exercise12videos
STATISTICS
Similar Questions
Show that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other
View SolutionShow that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other.
View SolutionShow that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other
View SolutionUsing vector method, prove that the line segments joining the mid-points of the adjacent sides of a quadrilateral taken in order form a parallelogram.
View SolutionProve that the lines joining the middle points of the opposite sides of a quadrilateral and the join of the middle points of its diagonals meet in a point and bisect one another
View SolutionProve that the line segments joints joining the mid-points of the adjacent sides of a quadrilateral from a parallelogram.
View SolutionShow that the segment joining the mid-points of a pair of opposite sides of a parallelogram, divides it into two equal parallelograms.
View SolutionProve that the line segments joining the mid-points of the sides of a triangle from four triangles, each of which is similar to the original triangle.
View Solutioni. Show that the lines joining the vertices of a tetrahedron to the centroids of opposite faces are concurrent.
ii. Show that the joins of the midpoints of the opposite edges of a tetrahedron intersect and bisect each other.View SolutionProve that the line segment joining the mid-points of the diagonals of a trapezium is parallel to each of the parallel sides and is equal to half the difference of these sides.
View Solution
