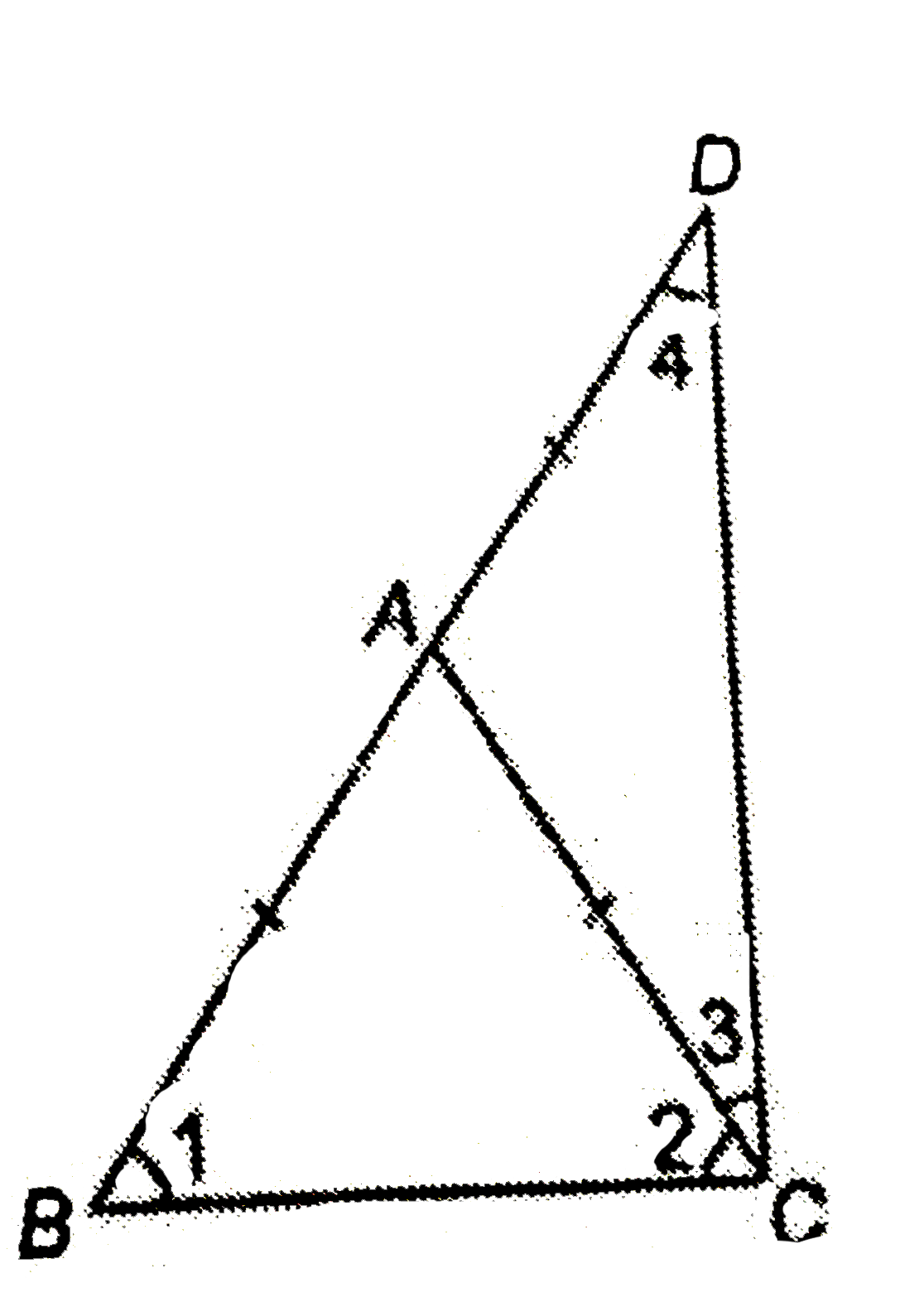
ABC is an isosceles triangle with AB=AC. Side BA is produced to D such that AB=AD. Prove that ∠BCD is a right angle.
Given : ΔABC in which AB=AC. Side BA is produced to D such that AB =AD.
To prove : ∠BCD=90∘ i.e., ∠2+∠3=90∘
Proof : since AB=AC (given)
∴∠2=∠1 ...(1)
(angle opposite to equal sides are equal)
Also, AB=AC=AD (given)
∠3=∠4 (angles opposite to equal sides are equal) ...(2)
Now, in ΔBCD,
∠1+(∠2+∠3)+∠4=180∘ (angle sum property) ...(3)
⇒ ∠2+(∠2+∠3)+∠3=180∘ [from (1), (2) and (3)]
⇒ 2(∠2+∠3)=180∘
⇒ ∠2+∠3=90∘. Hence Proved.
Topper's Solved these Questions
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Problems From NCERT/exemplar
5 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 7a
35 Videos
- SURFACE AREA AND VOLUMEBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)
10 Videos
Similar Questions
ABC is an isosceles triangle in which AB= AC . Side BA is produced to D such that AD = AB (see Fig. 7.34). Show that ∠BCD is a right angle.
View SolutionABC is an isosceles triangle with AB=AC and D is a point on AC such that BC2=ACXCD. Prove that BD=BC.
View SolutionABC is an isosceles triangle with AB=AC and D is a point on AC such that BC2=ACxCD. Prove that BD=BC
View SolutionIn a triangle ABC median AD is produced to X such that AD=DX. Prove that ABXC is a parallelogram.
View SolutionIn a triangle ABC median AD is produced to X such that AD=DX. Prove that ABXC is a parallelogram.
View SolutionIn a triangle ABC median AD is produced to X such that AD=DX. Prove that ABXC is a parallelogram.
View SolutionIn an isosceles triangle ABC with AB=AC , BD is perpendicular from B to the side AC . Prove that BD2−CD2=2 CDAD
View SolutionIn a triangle ABC, if AB=AC and AB is produced to D such that BD=BC, find ∠ACD : ∠ADC
View SolutionIn Figure, ABC is an isosceles triangle with AB=AC, BD and CE are two medians of the triangle. Prove that BD=CE
View SolutionIn an isosceles triangle ABC with AB=AC and BD⊥AC. Prove that BD2−CD2=2CD.AD.
View Solution
