If O is the origin and if the coordinates of any two points Q1andQ2 are (x1,y1)and(x2,y2), respectively, prove that OQ1.OQ2cos∠Q1OQ2=x1x2+y1y2.Solution in Tamil
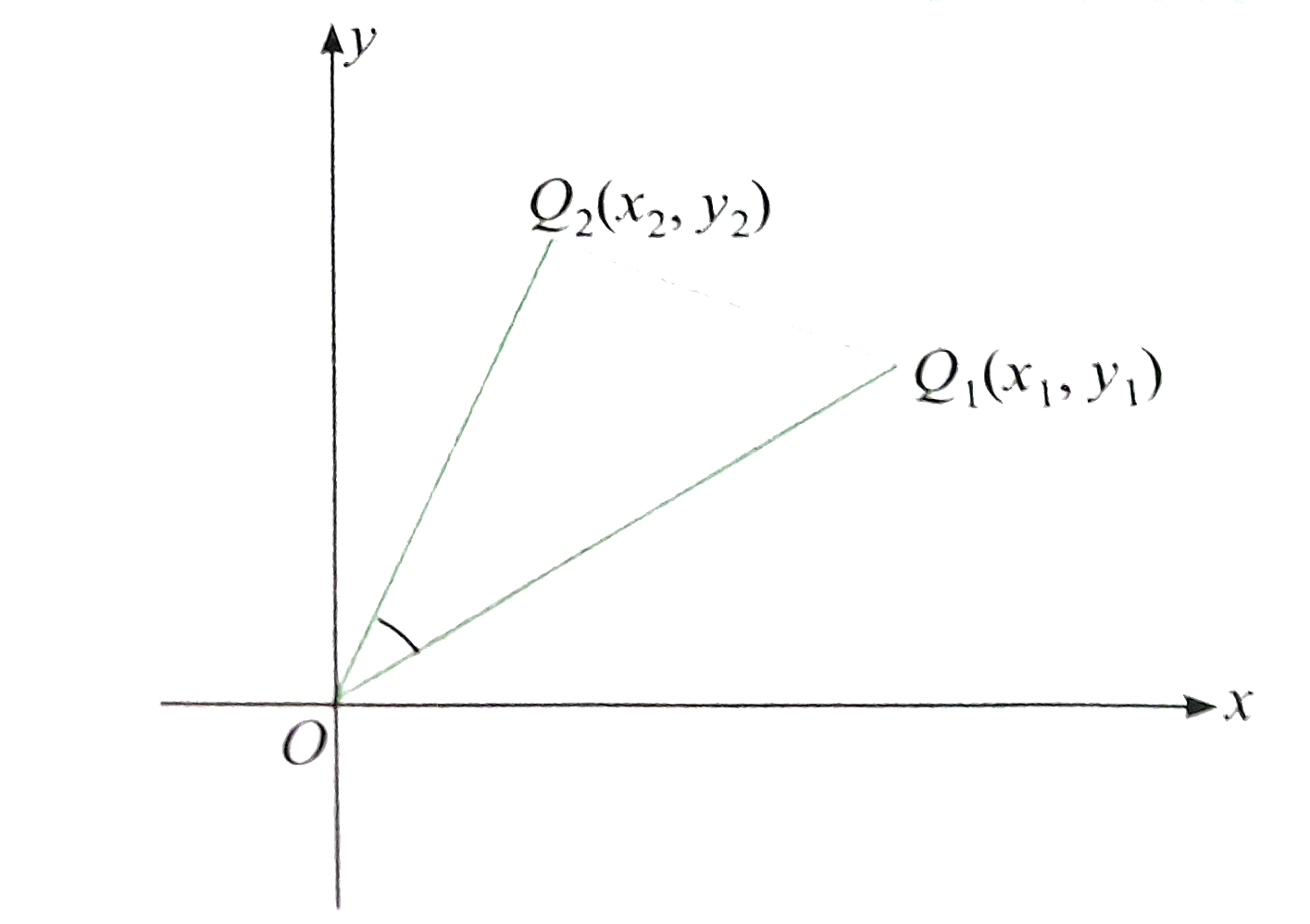
In ΔOQ1Q2, using cosing rule, we get
(Q−1Q2)2=(OQ1)2+(OQ2)2−OQ1.OQ2.cos(∠Q1OQ2)
∴(x2−x1)2+(y2−y1)2
=(x21+y21)+(x22+y22)−2OQ1.OQ2.cos(∠Q1OQ2)
⇒OQ1.OQ2cos(∠Q1OQ2)=x1x2+y1y2.
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.16videos
COORDINATE SYSYEM
- BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.28videos
COORDINATE SYSYEM
- BOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type2videos
COORDINATE SYSTEM
- BOOK - CENGAGECHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.213videos
CROSS PRODUCTS
Similar Questions
What is the minimum area of a triangle with integral vertices ?
03:05View SolutionThe distance between the points (acosα,asinα) and (acosβ,asinβ) is
02:27View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
05:17View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionLet A and B have coordinates (x_1,y_1) and (x_2,y_2) respectively. We define the distance between A and B as d(A,B) =max||x_2-x_1|,|y_2-y_1|| If d(A,O)=1 where O is the origin then locus of A has an area of
03:06View SolutionIf the line segment joining the points P(x1,y1)and Q(x2,y2) subtends an angle α at the origin O, prove that : OP.OQ cosα=x1x2+y1y2.
06:14View SolutionThree points A(x1,y1),B(x2,y2)andC(x,y) are collinear. Prove that: (x−x1)(y2−y1)=(x2−x1)(y−y1).
04:04View SolutionIf the coordinates of any two points Q1andQ2 are (x1,y1) and (x2,y2), respectively, then prove that OQ1×OQ2cos(∠Q1OQ2)=x1x−2+y−1y2, whose O is the origin.
05:32View SolutionIf the point x1+t(x2−x1),y1+t(y2−y1) divides the join of (x1,y1) and (x2,y2) internally then
02:08View Solutionयदि तीन बिंदु (x1,y1),(x2,y2) तथा (x3,y3) संरेख है तो सिद्ध कीजिए की
(x3−x1)(y2−y1)=(x2−x1)(y3−y1)04:00View SolutionIf O is the origin and if the coordinates of any two points Q1 and Q2 are (x1,y1) and (x2,y2), respectively, prove that OQ1.OQ2cos∠Q1OQ2=x1x2+y1y2.
05:42View SolutionIf the points (x1,y1),(x2,y2), and (x3,y3) are collinear show that y2−y3x2x3+y3−y1x3x1+y1−y2x1x2=0
07:06View SolutionIf the coordinates of any two points Q1andQ2 are (x1,y1) and (x2,y2), respectively, then prove that OQ1×OQ2cos(∠Q1OQ2)=x1x−2+y−1y2, whose O is the origin.
01:41View SolutionIf the line segment joining the points P(x1,y1)and Q(x2,y2) subtends an angle α at the origin O, prove that : OP.OQ cosα=x1x2+y1y2.
09:12View Solutionबिंदु (x1,y1),(x2,y2),(x1,y2) तथा (x2,y1) सदैव
03:30View SolutionIf O be the origin and if P(x1,y1)andP2(x2,y2) are two points, the OP1(OP2)sin∠P1OP2, is equal to
02:55View Solution

