Let $A (2, 0)$ and $B (z)$ are two points on the circle $| z | = 2$ . $M (z')$ is the point on $A B$ . If the point $¯ z'$ lies on the median of the triangle $O A B$ where $O$ is origin, then $a r g (z')$ is

A

${tan}^{- 1} (\frac{\sqrt{15}}{5})$

B

${tan}^{- 1} (\sqrt{15})$

C

${tan}^{- 1} (\frac{5}{\sqrt{15}})$

D

$\frac{π}{2}$

Text Solution

Verified by Experts

The correct Answer is:A

$(a)$ $M (z')$ is mid point of $A B$ , so $z' = \frac{z + 2}{2}$

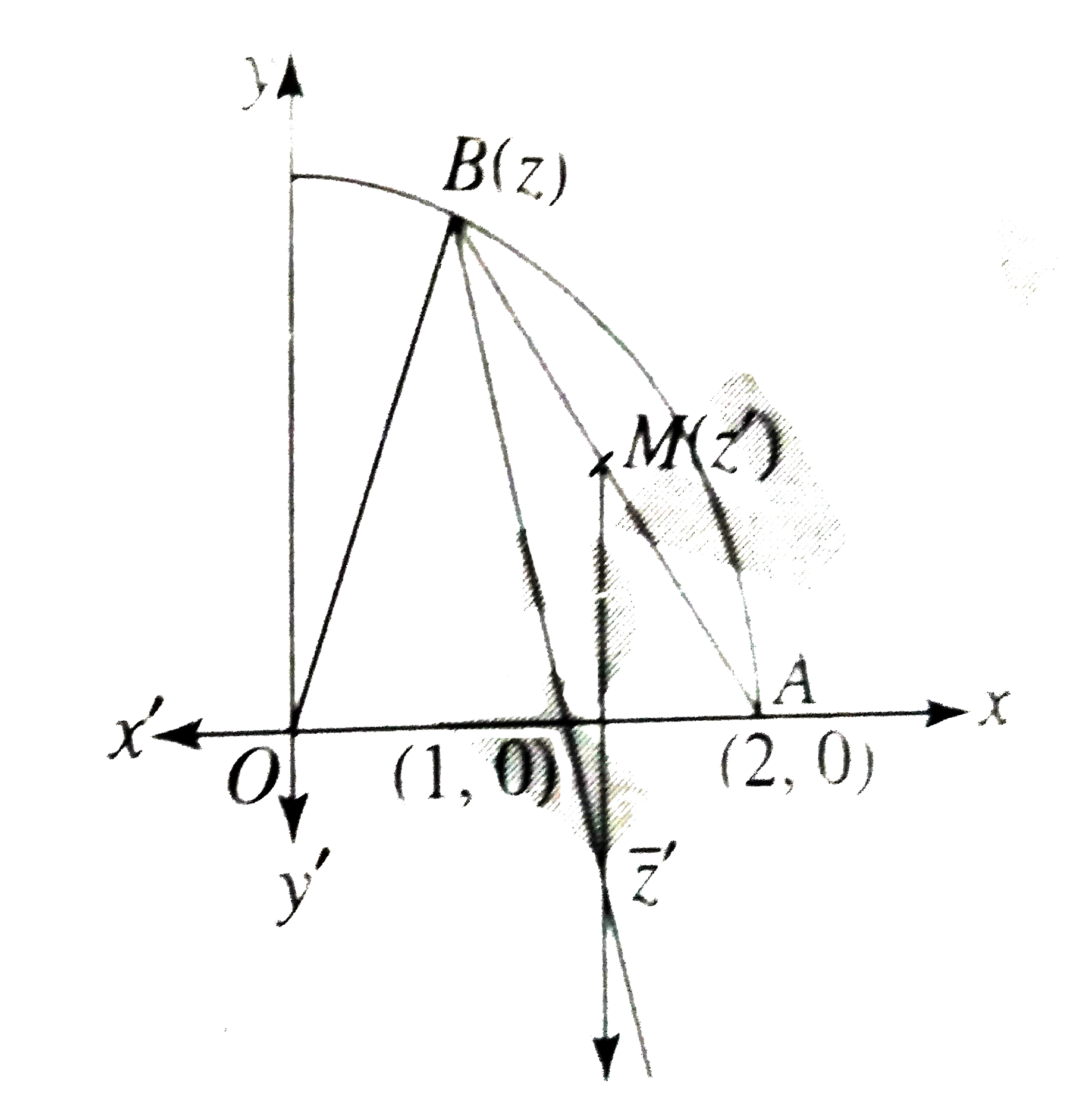
$\Rightarrow ¯ z' = \frac{¯ z + 2}{2}$
Now $z$ , $1$ , $\frac{¯ z}{2} + 1$ are collinear
$\Rightarrow ∣ ∣ ∣ ∣ ∣ \begin{matrix} z & ¯ z & 1 \frac{¯ z}{2} + 1 & \frac{z}{2} + 1 & 1 1 & 1 & 1 \end{matrix} ∣ ∣ ∣ ∣ ∣ = 0$
$\Rightarrow z (\frac{z}{2} + 1 - 1) - ¯ z (\frac{¯ z}{2} + 1 - 1) + 1 (\frac{¯ z}{2} - \frac{z}{2}) = 0$
$\Rightarrow \frac{z^{2}}{2} - \frac{{(¯ z)}^{2}}{2} + \frac{¯ z - z}{2} = 0$
$\Rightarrow (z - ¯ z) (z + ¯ z - 1) = 0$
$\Rightarrow z - ¯ z = 0$ or $(z + ¯ z - 1) = 0$
$\Rightarrow z + ¯ z = 1$ or $R e (z) = \frac{1}{2}$
$| z | = 2 \Rightarrow \frac{1}{4} + {(I m (z))}^{2} = 4$
$\Rightarrow I m (z) = \frac{\sqrt{15}}{2}$
$\Rightarrow z = \frac{1}{2} + \frac{i \sqrt{15}}{2}$
$\Rightarrow a r g (z') = {tan}^{- 1} (\frac{\sqrt{15}}{5})$

|

Updated on:21/07/2023

Class 12 MATHS COMPLEX NUMBERS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
If z is a point on the circle $| z - 1 | = 1,$ then arg z =
A $a r g | z - 1 |$
B $\frac{1}{2} a r g (z - 1)$
C $a r g (z^{2} - z)$
D $\frac{1}{3} a r g (z^{2} - z)$
Question 2 - Select One
If z lies on the circle $| z - 2 i | = 2 \sqrt{2}$ , then the value of arg $[\frac{z - 2}{z + 2}]$ is equal to
A $\frac{π}{3}$
B $\frac{π}{4}$
C $\frac{π}{6}$
D $\frac{π}{2}$
Question 3 - Select One
If 'z, lies on the circle $| z - 2 i | = 2 \sqrt{2}$ , then the value of $a r g (\frac{z - 2}{z + 2})$ is the equal to
A $\frac{π}{8}$
B $\frac{π}{6}$
C $\frac{π}{4}$
D $\frac{π}{3}$

CENGAGE-COMPLEX NUMBERS-JEE Advanced Previous Year

Home
Ask Doubt
Profile