Draw the graph of y=(x−1)(x2−x+1). Solution in Tamil
Video Solution
Text Solution
We have y=f(x)=(x+1)2/3+(x−1)23
Domain of the function is R.
Also the curve never intersects the x-axis.
f(x)=f(−x), so the function is even and hence the graph is symmetrical about the y-axis.
fx=23(x+1)−13+23(x−1)−13
=23(1(x+1)1/3+1(x−1)1/3)
=23(x+1)1/3+(x−1)1/3(x+1)1/3(x−1)1/3
Critical points are x=−1,0,1.
f′(x) does not exist at x=−1 and x=1, hence the function is non-differentiable at these points.
f′(0)=0
Sign scheme of fx is as shown in the following figure.
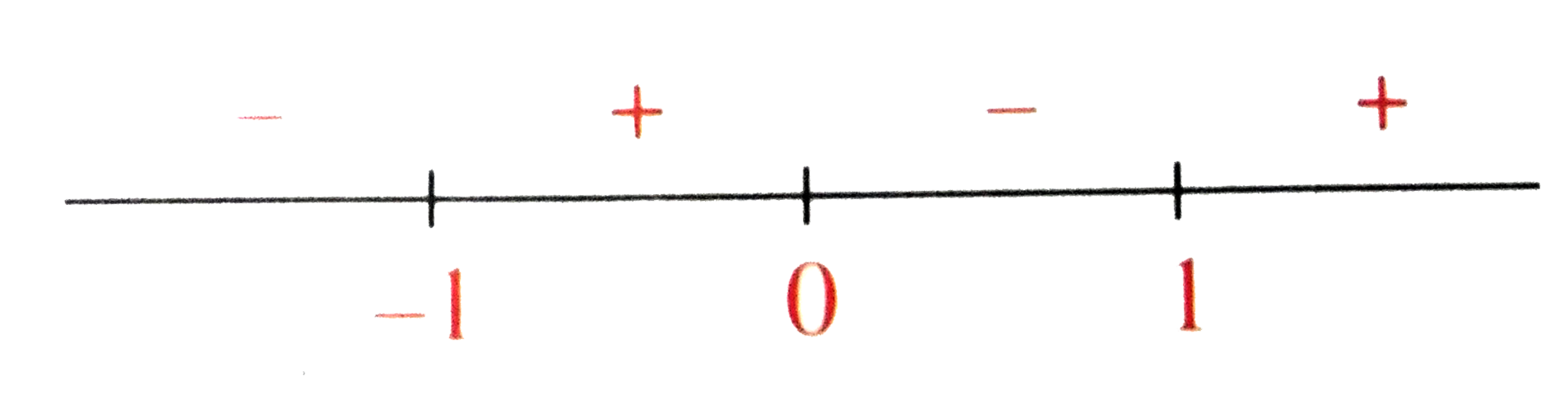
f(1)=f(−1)=22/3
limx→±∞[(x+1)2/3+(x−1)23]=∞
Thus, the graph of the function is as follows:

|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Draw the graph of y=sin2x−cosx
04:14View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
03:12View SolutionSolve: (x+2)3=2x(x2−1)
02:48View SolutionDraw the graph of y=x−1x2 and hence the graph of y=|x−1|x2.
02:16View SolutionDraw the graph of y=f(x)=x+1x2+1
01:18View SolutionDraw the graph of y=(x−1)(x2−x+1).
01:32View SolutionDraw graph of y=1x2−x.
02:16View SolutionDraw the graph of y=x−1x−2.
03:35View SolutionDraw the graph for |y|=(x−1)(x−2).
04:25View SolutionDraw the graph of y=x−1x2 and hence the graph of y=|x−1|x2.
03:14View SolutionDraw the graph of y=f(x)=x+1x2+1
03:03View SolutionDraw the graph of y=(x−1)(x2−x+1).
02:29View SolutionDraw graph of y=1x2−x.
02:52View SolutionDraw the graph of y=x−1x−2.
02:24View SolutionDraw the graph for |y|=(x−1)(x−2).
03:59View SolutionDraw the graph of y=(x−1)(x2−x+1).
01:39View Solution

