Photoelectric emission is observed from a surface for frequencies v1andv2 of the incident radiation (v1>v2) . If maximum kinetic energies of the photo electrons in the two cases are in the ratio 1:K , then the threshold frequency is given by:
v2−v1K−1
Kv1−v2K−1
Kv2−v1K−1
v2−v1K
The correct Answer is:B
To solve the problem, we will use the principles of the photoelectric effect. The key points to remember are:
1. The energy of the incident photon is given by E=hν, where h is Planck's constant and ν is the frequency of the incident radiation.
2. The maximum kinetic energy (KE) of the emitted photoelectrons can be expressed as:
KE=hν−ϕ
where ϕ is the work function of the material, which is related to the threshold frequency ν0 by ϕ=hν0.
1. Write the equations for the maximum kinetic energies:
For the two frequencies ν1 and ν2:
KE1=hν1−hν0(1)
KE2=hν2−hν0(2)
2. Express the kinetic energies in terms of the threshold frequency:
From equations (1) and (2), we can express the kinetic energies as:
KE1=h(ν1−ν0)
KE2=h(ν2−ν0)
3. Set up the ratio of the kinetic energies:
According to the problem, the maximum kinetic energies are in the ratio 1:K:
KE1KE2=1K
Substituting the expressions for KE1 and KE2:
h(ν1−ν0)h(ν2−ν0)=1K
4. Simplify the equation:
The h cancels out:
ν1−ν0ν2−ν0=1K
Cross-multiplying gives:
K(ν1−ν0)=ν2−ν0
5. Rearranging for the threshold frequency ν0:
Rearranging the equation:
Kν1−Kν0=ν2−ν0
Kν1−ν2=Kν0−ν0
Kν1−ν2=(K−1)ν0
Finally, solving for ν0:
ν0=Kν1−ν2K−1
Final Answer:
The threshold frequency ν0 is given by:
ν0=Kν1−ν2K−1
2. The maximum kinetic energy (KE) of the emitted photoelectrons can be expressed as:
KE=hν−ϕ
where ϕ is the work function of the material, which is related to the threshold frequency ν0 by ϕ=hν0.
For the two frequencies ν1 and ν2:
KE1=hν1−hν0(1)
KE2=hν2−hν0(2)
From equations (1) and (2), we can express the kinetic energies as:
KE1=h(ν1−ν0)
KE2=h(ν2−ν0)
According to the problem, the maximum kinetic energies are in the ratio 1:K:
KE1KE2=1K
Substituting the expressions for KE1 and KE2:
h(ν1−ν0)h(ν2−ν0)=1K
The h cancels out:
ν1−ν0ν2−ν0=1K
Cross-multiplying gives:
K(ν1−ν0)=ν2−ν0
Rearranging the equation:
Kν1−Kν0=ν2−ν0
Kν1−ν2=Kν0−ν0
Kν1−ν2=(K−1)ν0
Finally, solving for ν0:
ν0=Kν1−ν2K−1
The threshold frequency ν0 is given by:
ν0=Kν1−ν2K−1
Similar Questions
Photoelectric emission is observed from a surface for frequencies v1andv2 of incident radiations
(v1>v2). If the maximum kinetic energy of photoelectrons in the two cases are in the ratio of 2:1 then threshold frequency v0 is given by.View SolutionPhotoelectric emission is observed from a metallic surface for frequencies v1 and v2 of the incident light rays (v1>v2). If the maximum values of kinetic energy of the photoelectrons emitted in the two cases are in the ratio of 1:k , then the threshold frequency of the metallic surface is
View SolutionPhotoelectric emission is observed from a metallic surface for frequencies v1 and v2 of the incident light rays (v1>v2). If the maximum values of kinetic energy of the photoelectrons emitted in the two cases are in the ratio of 1:k , then the threshold frequency of the metallic surface is
View SolutionPhotoelectric emmision is observed from a surface when lights of frequency n1and n2 incident.If the ratio of maximum kinetic energy in two cases is K:1 then ( Assume n1>n2) threshod frequency is
View SolutionA If the wavelength of the incident radiation changes from λ1 to λ2 then the maximum kinetic energy of the emitted photo electrons changes from K to K,, then the work function of the emitter surface is
View SolutionMaximum kinetic energy (Ek) of a photoelectron varies with the frequency (v) of the incident radiation as
View SolutionThe photoelectric threshold frequency of a metal is v. When light of frequency 4v is incident on the metal . The maximum kinetic energy of the emitted photoelectrons is
View SolutionGiven in fig. is the graph between frequency v of the incident light and maximum kinetic energy (Ek) of the emitted photoelectrons. Find the values of (i) threshold frequency and (ii) work function form the graph.
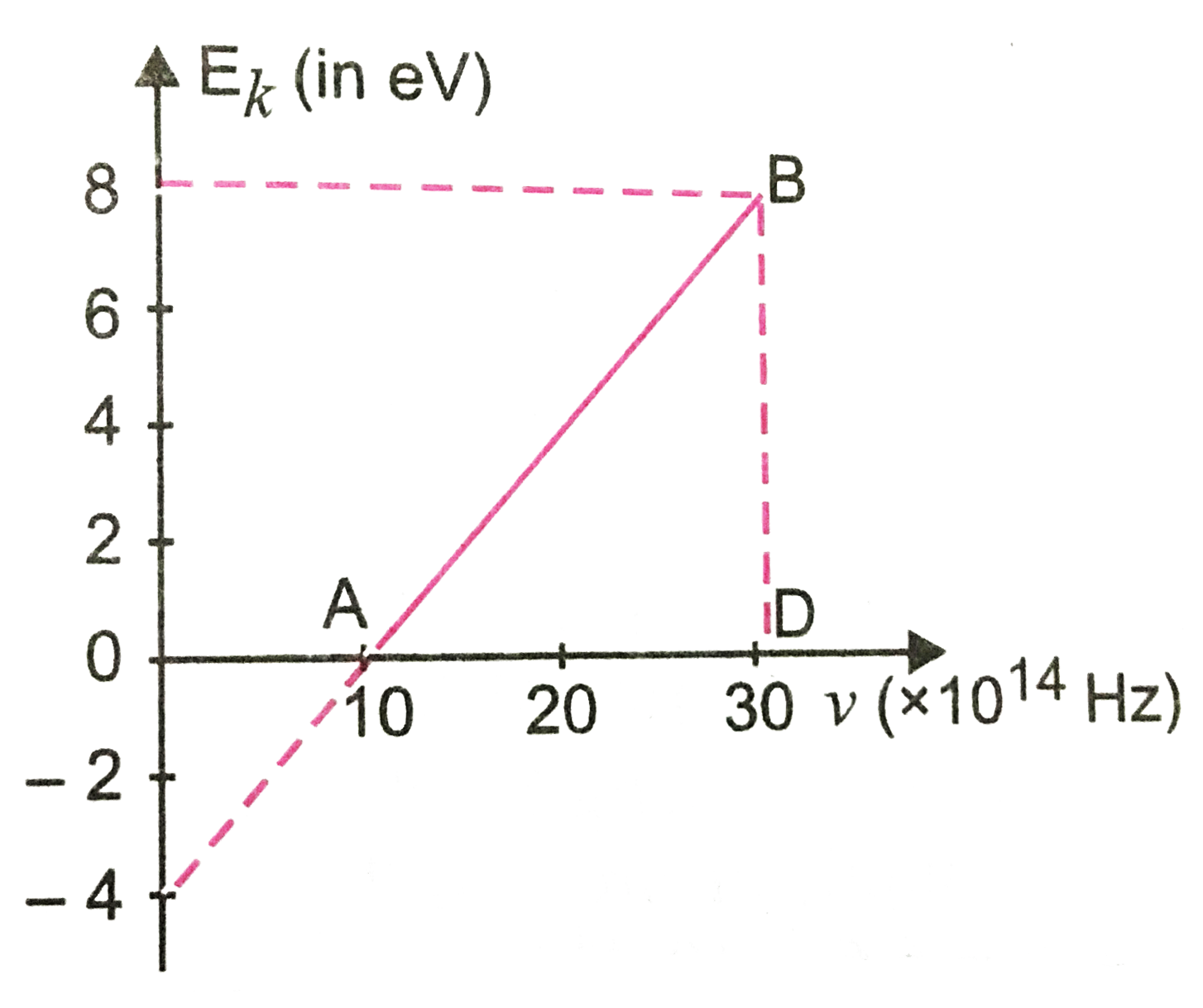 View Solution
View SolutionTwo masses, m1 and m2 , are moving with velocities v1 and v2. Find their total kinetic energy in the reference frame of centre of mass.
View SolutionThe work functions of metals A and B are in the ratio 1:2. If light of frequencies f and 2f are incident on the surfaces of A and B respectively , the ratio of the maximum kinetic energy of photoelectrons emitted is ( f is greater than threshold frequency of A,2f is greater than threshold frequency of B)
View Solution

