A circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumferences of the discs coincide. The centre of mass of the new disc is αR from the centre of the bigger disc. The value of α is :
1/4
1/3
1/2
1/6
The correct Answer is:B
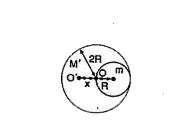
(M.+m)=M=π(2R)2.σ, when σ= mass per unit area,m=πR2.σ
M.=3πR2.σ,3πR2.σ.x+πR2σ.RM=0
Because for the full disc, the centre of mass is at the centre O. or x=−R3=αR
∴|α|=∣∣∣−13∣∣∣=13
The centre of mass is at R3 to the left on the diameter of the original disc.
Similar Questions
A circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumferences of the discs coincide. The center of mass of new disc is αR from the center of the bigger disc. The value of α is
View SolutionA circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumferences of the discs coincide. The center of mass of new disc is αR from the center of the bigger disc. The value of α is
View Solution
Knowledge Check
- Question 1 - Select One
A circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumference of the discs coincoid . The centre of mass of the new disc is αR from the centre of the bigger disc . the value of α is
A1/3B1/2C1/6D1/4 - Question 2 - Select One
A circular disc of radius R is removed from a bigger circular dise of radius such that the circumferences of the discs coincide. The centre of mass of the new disc is αR from the centre of the bigger disc. The value of a is:
A12B16C14D13 - Question 3 - Select One
A circular disc of radius R is removed from a bigger circular disc of radius 2R such that the cirucmferences of the discs coincide. The centre of mass of the new disc is αR from the center of the bigger disc. The value of α is
A14B13C12D16
A circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumference of the discs coincoid . The centre of mass of the new disc is αR from the centre of the bigger disc . the value of α is
View SolutionA solid toy is in the form of a hemisphere surmounted by a right circular cone of same radius. The height of the cone is 10 Cm and the radius of the base is 7 cm. Determine the volume of the toy. Also find the area of the coloured sheet required to cover the toy. (Use π=22/7 and √149=12.2)
View SolutionA circular disc of radius R is removed from a bigger circular disc of radius 2R, such that the circumference of the discs coincide. The centre of the bigger disc. The value of α is
View SolutionA circular disc of radius R is removed from a bigger circular disc of radius 2R, such that the circumference of the discs coincide. The centre of the bigger disc. The value of α is
View SolutionA circular disc of radius R is removed from a bigger of the discs coincide. The centre of mass of the new disc is ar from the centre of the bigger disc. The value of α is
View Solution

