Calculate the magnetic flux coming out from the surface containing magnetic dipole (say, a bar magnet) as shown in figure.
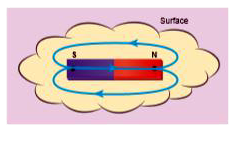
Magnetic dipole is kept, the total flux emanating from the closed surface S is zero. So,
ΦB=∮→B.d→A=0
Here the integral is taken over closed surface. Since no isolated magnetic pole (called magnetic monopole) exists, this integral is always zero,
∮→B.d→A=0
This is similar to Gauss’s law in electrostatics.
Topper's Solved these Questions
- BOOK - NCERT TAMILCHAPTER - MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENTEXERCISE - EVALUATION (I Multiple choice questions)15videos
MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENT
- BOOK - NCERT TAMILCHAPTER - MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENTEXERCISE - EVALUATION (IV. Numerical problems)10videos
MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENT
- BOOK - NCERT TAMILCHAPTER - ELECTROSTATICSEXERCISE - Exercises23videos
ELECTROSTATICS
- BOOK - NCERT TAMILCHAPTER - MAGNETISM AND MATTEREXERCISE - Additional Exercises19videos
MAGNETISM AND MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One
The net magnetic flux through any closed surface, kept in a magnetic field is
AzeroBμ04πC4πμ0D4μ0π - Question 2 - Select One
The net magnetic flux through any closed surface, kept in a magnetic field is
AzeroBμ04πC4πμ0D4μ0π - Question 3 - Select One
The net magnetic flux through any closed surface, kept in a magnetic field is
AzeroBμ04πC4πμ0D4μ0π
A long copper wire carries a current of 10 ampere . Calculate the magnetic flux per metre of the wire for a plane surface S inside the wire as shown in the figure.
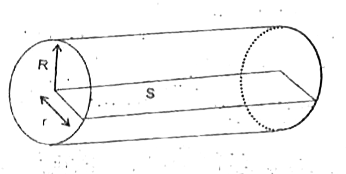 View Solution
View SolutionA long copper wire carries a current of 10 ampere . Calculate the magnetic flux per metre of the wire for a plane surface S inside the wire as shown in the figure.
 View Solution
View SolutionCalculate potential energy of a magnetic dipole in a magnetic field.
View SolutionThe magnetic field lines due to a bar magnet are correctly shown in figure.
View SolutionAssertion : The magnetic flux through a closed surface containing a bar magnet is zero.
Reason : Gauss's law applies in the case of electric flux only.View Solution
