Compound 'A' and B react according to the following chemical equation.
A(g)+2B(g)→2C(g)
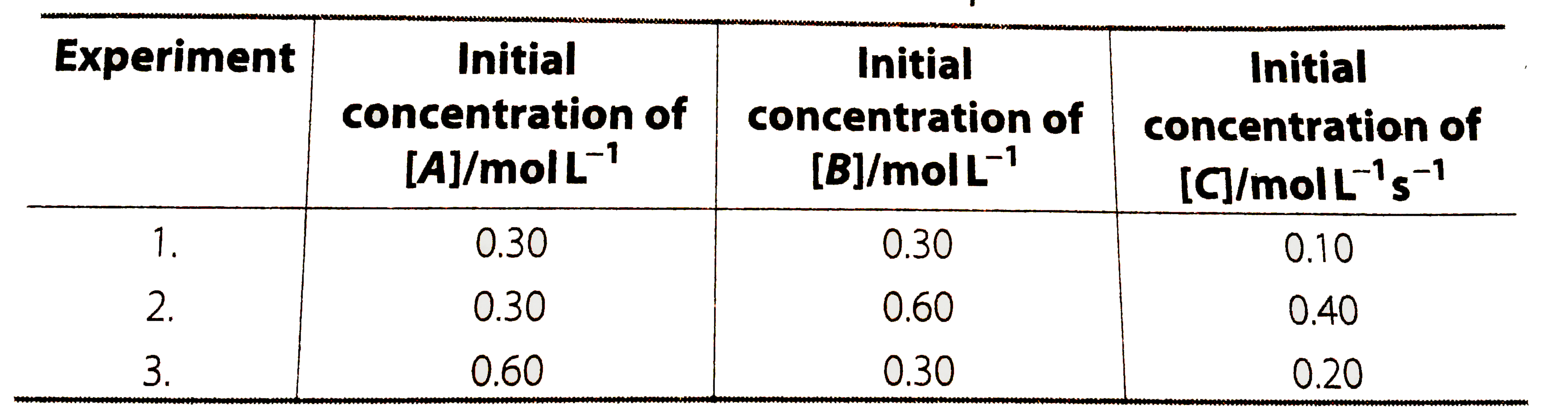
Concentration of either A or B were changed keeping the concentrations of one of the reactants constant and rates were mesured as a function of initial concentration. Following results were obtained. Choose the correct option for the rate equations for this reaction.
ExperimentInitial concentration of[A]/molL−1Initial concentration of[B]molL−1Initial rate of formation of[c]/molL−1s−110.300.300.1020.300.600.4030.600.300.20
Rate =k[A]2[B]
Rate =k[A][B]2
Rate =k[A][B]
Rate = k[A]2[B]0
The correct Answer is:B
To determine the rate equation for the reaction A(g)+2B(g)→2C(g) based on the given experimental data, we will analyze the initial concentrations of reactants and the corresponding initial rates of formation of product C.
Step 1: Write the general rate law expression
The rate of the reaction can be expressed as:
Rate=k(A)x(B)y
where k is the rate constant, x is the order of the reaction with respect to A, and y is the order of the reaction with respect to B.
Step 2: Analyze the data from the experiments
We have the following data from the experiments:
| Experiment | (A) (mol L−1) | (B) (mol L−1) | Rate (mol L−1 s−1) |
|------------|-----------------------|-----------------------|-----------------------------------|
| 1 | 0.30 | 0.30 | 0.10 |
| 2 | 0.30 | 0.60 | 0.40 |
| 3 | 0.60 | 0.30 | 0.20 |
Step 3: Determine the order with respect to B using Experiments 1 and 2
In Experiment 1 and Experiment 2, the concentration of A is constant, while the concentration of B changes.
- For Experiment 1:
Rate1=k(0.30)x(0.30)y=0.10
- For Experiment 2:
Rate2=k(0.30)x(0.60)y=0.40
Dividing the second equation by the first:
0.400.10=k(0.30)x(0.60)yk(0.30)x(0.30)y
This simplifies to:
4=(0.60)y(0.30)y
4=(0.600.30)y=2y
Thus, y=2.
Step 4: Determine the order with respect to A using Experiments 1 and 3
Now, we will compare Experiment 1 and Experiment 3, where the concentration of B is constant, and the concentration of A changes.
- For Experiment 1:
Rate1=k(0.30)x(0.30)2=0.10
- For Experiment 3:
Rate3=k(0.60)x(0.30)2=0.20
Dividing the third equation by the first:
0.200.10=k(0.60)x(0.30)2k(0.30)x(0.30)2
This simplifies to:
2=(0.60)x(0.30)x
2=(0.600.30)x=2x
Thus, x=1.
Step 5: Write the final rate equation
Now that we have determined x=1 and y=2, we can write the rate equation as:
Rate=k(A)1(B)2
Conclusion
The correct rate equation for the reaction is:
Rate=k(A)(B)2
The rate of the reaction can be expressed as:
Rate=k(A)x(B)y
where k is the rate constant, x is the order of the reaction with respect to A, and y is the order of the reaction with respect to B.
We have the following data from the experiments:
|------------|-----------------------|-----------------------|-----------------------------------|
| 1 | 0.30 | 0.30 | 0.10 |
| 2 | 0.30 | 0.60 | 0.40 |
| 3 | 0.60 | 0.30 | 0.20 |
In Experiment 1 and Experiment 2, the concentration of A is constant, while the concentration of B changes.
Rate1=k(0.30)x(0.30)y=0.10
- For Experiment 2:
Rate2=k(0.30)x(0.60)y=0.40
0.400.10=k(0.30)x(0.60)yk(0.30)x(0.30)y
This simplifies to:
4=(0.60)y(0.30)y
4=(0.600.30)y=2y
Thus, y=2.
Now, we will compare Experiment 1 and Experiment 3, where the concentration of B is constant, and the concentration of A changes.
Rate1=k(0.30)x(0.30)2=0.10
- For Experiment 3:
Rate3=k(0.60)x(0.30)2=0.20
0.200.10=k(0.60)x(0.30)2k(0.30)x(0.30)2
This simplifies to:
2=(0.60)x(0.30)x
2=(0.600.30)x=2x
Thus, x=1.
Now that we have determined x=1 and y=2, we can write the rate equation as:
Rate=k(A)1(B)2
The correct rate equation for the reaction is:
Rate=k(A)(B)2
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - ASSERTION & REASON
15 Videos
- CHEMICAL KINETICSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Rate Of A Chemical Reaction
17 Videos
- CHEMICAL KINETICSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Higher Order Thinking Skills
9 Videos
- BIOMOLECULESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - BIOMOLECULESEXERCISE - Assertion And Reason
15 Videos
- CHEMISTRY IN EVERYDAY LIFE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - CHEMISTRY IN EVERYDAY LIFE EXERCISE - NCERT Exemplar
15 Videos
Similar Questions
Compounds 'A' and 'B' react according to the following chemical equation.
A(g)→2B(g)→2C(g)
Concentration of either 'A' or 'B' were changed Keeping the concentration of one of the reactants constant and rates were measured as a function of initial concentration. Following result were obtained.
Choose the correct option for the rate equations for this reaction.
View SolutionFor the reaction A+B→C+D, doubling the concentration of both the reactants increases the reaction rate by 8 times and doubling the initial concentration of only B ismply doubles the reaction rate. What is the rate law for the reaction ?
View Solution
Knowledge Check
Compounds 'A' and 'B' react according to the following chemical equation.
A(g)→2B(g)→2C(g)
Concentration of either 'A' or 'B' were changed Keeping the concentration of one of the reactants constant and rates were measured as a function of initial concentration. Following result were obtained.
Choose the correct option for the rate equations for this reaction.
 ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘
ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘Compounds 'A' and 'B' react according to the following chemical equation.
A(g)→2B(g)→2C(g)
Concentration of either 'A' or 'B' were changed Keeping the concentration of one of the reactants constant and rates were measured as a function of initial concentration. Following result were obtained.
Choose the correct option for the rate equations for this reaction.
 ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘
ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘Compounds 'A' and 'B' react according to the following chemical equation.
A(g)→2B(g)→2C(g)
Concentration of either 'A' or 'B' were changed Keeping the concentration of one of the reactants constant and rates were measured as a function of initial concentration. Following result were obtained.
Choose the correct option for the rate equations for this reaction.
 ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘
ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘
In a reaction A + B → Products
(i) If the initial concentration of A is doubled and B is kept constant, the rate of the reaction is doubled
(ii) If the initial concentration both A and B are doubled the rate of reaction becomes eight times
The rate law of the reaction is :
View SolutionFor a reaction 2A+B→ Products, doubling the initial concentration of both the reactants increases the rate by a factor of 8, and doubling the concentration of B alone doubles the rate. The rate law for the reaction is:
View SolutionRate law for the reaction A+2B→Cis found to be Rate =k[A][B] Concentration of reactant 'B' is doubled. Keeping the concentration of 'A' constant, the value of rate constant will be:
View SolutionRate law for the reaction A+2B→Cis found to be Rate =k[A][B] Concentration of reactant 'B' is doubled. Keeping the concentration of 'A' constant, the value of rate constant will be:
View SolutionRate law for the reaction A+2B→Cis found to be Rate =k[A][B] Concentration of reactant 'B' is doubled. Keeping the concentration of 'A' constant, the value of rate constant will be:
View Solution
