Consider the following transformation:
Cr(CO)x→Cr(CO)y(NO)x
If both reactant and product follow EAN rule, then calculate value of x+z−y (where x,y and z are natural numbers).
The correct Answer is:5
To solve the problem, we need to analyze the transformation of the coordination compound from Cr(CO)_(x) to Cr(CO)_(y)(NO)_(z) and ensure both follow the Effective Atomic Number (EAN) rule.
Step 1: Identify the EAN for Cr(CO)_(x)
The atomic number of chromium (Cr) is 24. Each carbonyl (CO) ligand donates 2 electrons. Therefore, the EAN for Cr(CO)_(x) can be calculated as follows:
EAN=Atomic Number of Cr+2×x
EAN=24+2x
Step 2: Determine the EAN for Cr(CO)_(y)(NO)_(z)
In the product Cr(CO)_(y)(NO)_(z), carbonyl (CO) still donates 2 electrons, while nitrosyl (NO) donates 3 electrons. The EAN can be expressed as:
EAN=Atomic Number of Cr+2×y+3×z
EAN=24+2y+3z
Step 3: Apply the EAN Rule
According to the problem, both reactant and product must satisfy the EAN rule, which means:
24+2x=24+2y+3z
Step 4: Simplify the Equation
By simplifying the equation, we can eliminate the common terms:
2x=2y+3z
Step 5: Rearrange the Equation
Rearranging gives us:
2x−2y=3z
2(x−y)=3z
Step 6: Solve for Natural Numbers
Since x, y, and z are natural numbers, we can express z in terms of x and y:
z=2(x−y)3
To ensure z is a natural number, 2(x−y) must be divisible by 3. This implies that x−y must be a multiple of 3.
Step 7: Assign Values to x, y, and z
To find suitable values for x, y, and z, we can try different values for x and y.
Let’s assume:
- If x=6 (which corresponds to 3 CO ligands),
- Then y=3 (which corresponds to 1 CO ligand),
- We can substitute these values into the equation to find z:
z=2(6−3)3=2×33=2
Step 8: Calculate x + z - y
Now we can calculate x+z−y:
x+z−y=6+2−3=5
Final Answer
Thus, the value of x+z−y is:
5
The atomic number of chromium (Cr) is 24. Each carbonyl (CO) ligand donates 2 electrons. Therefore, the EAN for Cr(CO)_(x) can be calculated as follows:
EAN=24+2x
In the product Cr(CO)_(y)(NO)_(z), carbonyl (CO) still donates 2 electrons, while nitrosyl (NO) donates 3 electrons. The EAN can be expressed as:
EAN=24+2y+3z
According to the problem, both reactant and product must satisfy the EAN rule, which means:
By simplifying the equation, we can eliminate the common terms:
Rearranging gives us:
2(x−y)=3z
Since x, y, and z are natural numbers, we can express z in terms of x and y:
To find suitable values for x, y, and z, we can try different values for x and y.
- If x=6 (which corresponds to 3 CO ligands),
- Then y=3 (which corresponds to 1 CO ligand),
- We can substitute these values into the equation to find z:
Now we can calculate x+z−y:
Thus, the value of x+z−y is:
Topper's Solved these Questions
- CO-ORDINATION COMPOUNDSBOOK - VK JAISWAL ENGLISHCHAPTER - CO-ORDINATION COMPOUNDSEXERCISE - LEVEL 2
144 Videos
- CO-ORDINATION COMPOUNDSBOOK - VK JAISWAL ENGLISHCHAPTER - CO-ORDINATION COMPOUNDSEXERCISE - ASSERTION-REASON TYPE QUESTIONS
26 Videos
- CHEMICAL BONDING (BASIC)BOOK - VK JAISWAL ENGLISHCHAPTER - CHEMICAL BONDING (BASIC)EXERCISE - SUBJECTIVE PROBLEMS
54 Videos
- d-BLOCK ELEMENTSBOOK - VK JAISWAL ENGLISHCHAPTER - d-BLOCK ELEMENTSEXERCISE - ASSERTION-REASON TYPE QUESTIONS
32 Videos
Similar Questions
M(CO)x(NO)y+NO−−−→−COM(NO)z
Where EAN of metal (M) in both produce and reactant is samme and it is 54. then calculate value of (x+y=-z). (where x,y and z are netural numbers and M belong to 6th group according to long form of periodic table)
View SolutionConsider the following carbonyl complex compounds.
(i) Mo(CO)x
(ii) Hy[Cr(CO)5] and (iii) Co2(CO)z
Then calculate value of |x+y−z|.
View SolutionConsider the following carbonyl complex compounds.
(i) Mo(CO)x
(ii) Hy[Cr(CO)5] and (iii) Ru3(CO)s
Then calculate value of |x+y−x|.
View SolutionConsider the following parallelograms. Find the values of the unknowns x, y, z.
View SolutionFactorize each of the following expressions : x(x+z)−y(y+z)
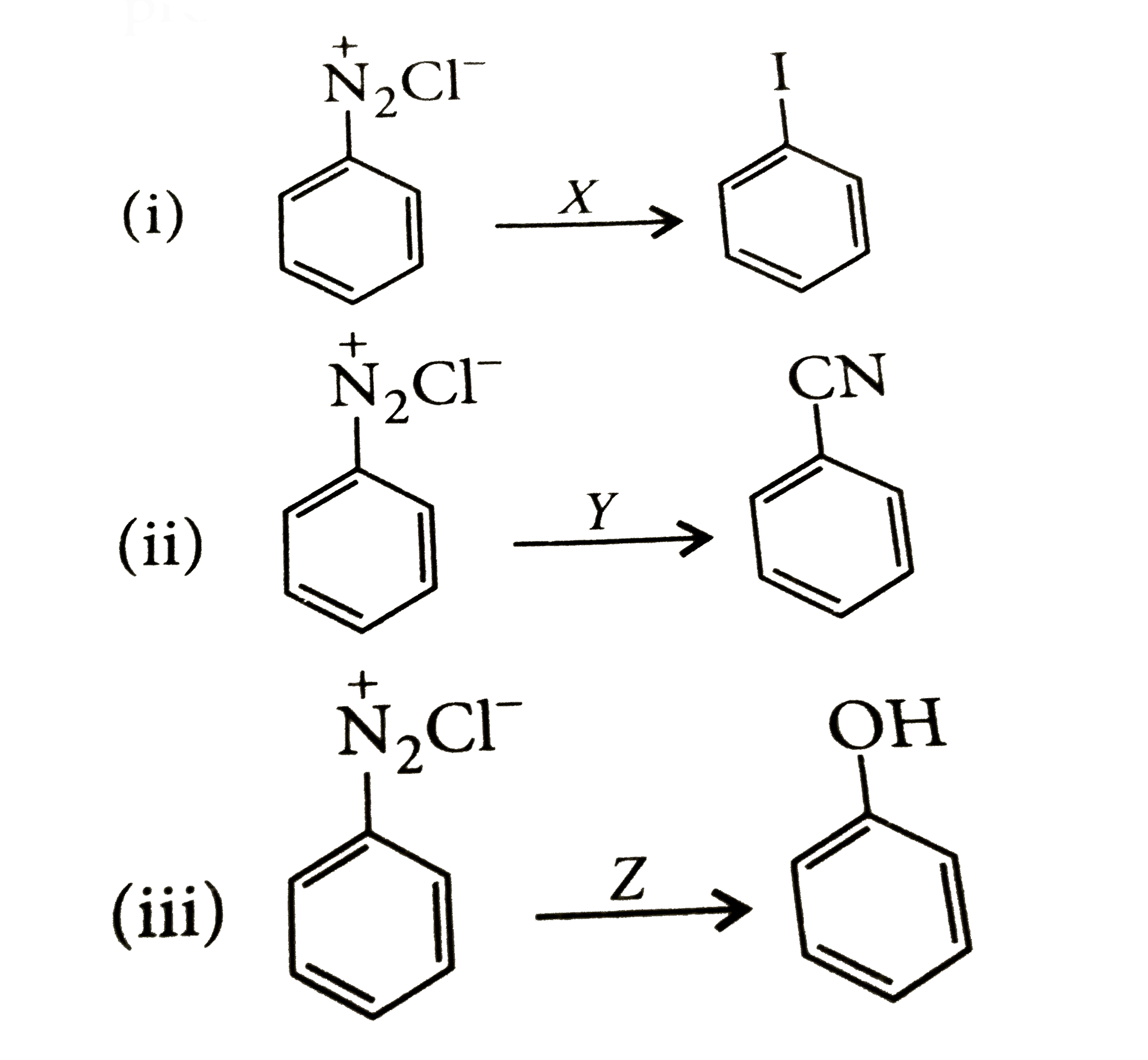
View SolutionIdentify the reagents X,Y and Z for the following products.
View SolutionIf cosx=tany,cosy=tanzandcosz=tanx, then sinx=2sinθ where θ is (where, x,y,z,θ are acuate angles)
View SolutionFollowing sidwick.s rule of EAN, Co(CO)x will be:
View SolutionIf x,y,z are three real numbers of the same sign, then the value of (xy+yz+zx) lies in the interval _____
View SolutionFor oxyacid HClOx, if x=y=z (x,y and z are natural numbers), then calculate the value of |x+y+z|. Where x=number of 'O' atoms
y=total number of lone pairs at central atom
z=total number of pi(π) electrons in the oxyacid.
View Solution
