In the study of inversion of sucrose in presence of acid, if r0,r1 and r∞ represent the polarimetric readings at times 0, t and ∞ respectively, then at the 50% inversion, which of the following relationship will hold good ?
rt=r0+r∞
rt=12(r0+r∞)
rt=r0−r∞
rt=12(r0−r∞)
The correct Answer is:B
To solve the problem regarding the inversion of sucrose in the presence of acid and the polarimetric readings at different times, we can follow these steps:
1. Define the Polarimetric Readings:
- Let R0 be the polarimetric reading at time t=0.
- Let Rt be the polarimetric reading at time t.
- Let R∞ be the polarimetric reading at time t=∞.
2. Understand the Concept of Inversion:
- The inversion of sucrose refers to the conversion of sucrose into glucose and fructose, which can be monitored by changes in optical rotation (polarimetric readings).
- At 50% inversion, half of the sucrose has been converted.
3. Relate Polarimetric Readings to Concentration:
- The change in polarimetric readings can be related to the concentration of sucrose remaining.
- The relationship can be expressed as:
R0−Rt∝x
where x is the amount of sucrose that has been inverted.
4. Determine x at 50% Inversion:
- At 50% inversion, the amount of sucrose inverted x is equal to half of the initial concentration A:
x=A2
5. Express the Change in Polarimetric Readings:
- The change in readings can also be expressed as:
R0−R∞∝A
- Therefore, at 50% inversion:
R0−Rt=A2
6. Substituting the Proportional Relationships:
- From the above relationships, we can write:
R0−Rt=12(R0−R∞)
7. Rearranging the Equation:
- Rearranging gives:
2(R0−Rt)=R0−R∞
- Expanding this leads to:
2R0−2Rt=R0−R∞
8. Solving for Rt:
- Rearranging the terms, we get:
2Rt=R0+R∞
- Thus, we find:
Rt=12(R0+R∞)
Final Result:
At 50% inversion, the relationship that holds good is:
Rt=12(R0+R∞)
- Let R0 be the polarimetric reading at time t=0.
- Let Rt be the polarimetric reading at time t.
- Let R∞ be the polarimetric reading at time t=∞.
- The inversion of sucrose refers to the conversion of sucrose into glucose and fructose, which can be monitored by changes in optical rotation (polarimetric readings).
- At 50% inversion, half of the sucrose has been converted.
- The change in polarimetric readings can be related to the concentration of sucrose remaining.
- The relationship can be expressed as:
R0−Rt∝x
where x is the amount of sucrose that has been inverted.
- At 50% inversion, the amount of sucrose inverted x is equal to half of the initial concentration A:
x=A2
- The change in readings can also be expressed as:
R0−R∞∝A
- Therefore, at 50% inversion:
R0−Rt=A2
- From the above relationships, we can write:
R0−Rt=12(R0−R∞)
- Rearranging gives:
2(R0−Rt)=R0−R∞
- Expanding this leads to:
2R0−2Rt=R0−R∞
- Rearranging the terms, we get:
2Rt=R0+R∞
- Thus, we find:
Rt=12(R0+R∞)
At 50% inversion, the relationship that holds good is:
Rt=12(R0+R∞)
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - PRADEEPCHAPTER - CHEMICAL KINETICSEXERCISE - COMPETITION FOCUS (JEE(Main and Advanced)/Medical Entrance) (II. Multiple Choice Questions)
1 Videos
- CHEMICAL KINETICSBOOK - PRADEEPCHAPTER - CHEMICAL KINETICSEXERCISE - COMPETITION FOCUS (JEE(Main and Advanced)/Medical Entrance) (I. Multiple Choice Questions) With one or more than one correct answers
7 Videos
- CHEMICAL KINETICSBOOK - PRADEEPCHAPTER - CHEMICAL KINETICSEXERCISE - VALUE BASED QUESTIONS (WITH ANSWERS)
2 Videos
- BIOMOLECULES BOOK - PRADEEPCHAPTER - BIOMOLECULES EXERCISE - IMPORTANT QUESTIONS (FOR BOARD EXAMINATION)
25 Videos
- CHEMISTRY IN EVERYDAY LIFE BOOK - PRADEEPCHAPTER - CHEMISTRY IN EVERYDAY LIFE EXERCISE - IMPORTANT QUESTION FOR BOARD EXAMINATION
30 Videos
Similar Questions
If sinθ and cosθ are the roots of the quadratic equation px2+qx+r=0(p≠0), then which of the following relation holds good?
View SolutionInverison of sucrose is studied by measuring the angle of rotation at any time t.
C12H22O11(Sucrose)+H2O(Glucose)H⊕−−→C6H12(Fructose)O6+C6H12O6
It is found that
(r∞−r0)∝a and (r∞−rt)∝,(a−x), where r0,rt, and r∞ are the angle of rotation at the start, at the time t, and at the end of the reaction, respectively. Form the following values calculate the rate constant and the time at which the solution is optically inactive.
∣∣∣Time (min)0.046.0∞Rotation of polarized light (degree)24.110.0−10.7∣∣∣
View Solution
Knowledge Check
Let f : R → R be a function such that limx→∞f(x)=M>0. Then which of the following is false?
Alimx→∞xsin(1/x)f(x)=MBlimx→∞sin(f(x))=sinMClimx→∞xsin(e−x)f(x)=MDlimx→∞sinxxf(x)=0Let f(x)=∫x0dt√1+t2 and g(x) be the inverse of f(x), then which one of the following holds good ?
A2g=g2Bg''(x)=g(x)C3g=2g2D3g=g2Inversion of sucrose (C12H22O11) is first-order reaction and is studied by measuring angle of rotation at different instants of time
C12H22O11Sucrosed+H2OH+−−→C6H12O6Glucosed+C6H12O6Fructosel
If (r∞−r0)=a and (r∞−rt)=(a−x) (where r0,rt and r∞) are the angle of rotation at the start, at the time t and at the end of the reaction respectively, then there is 50% inversion when :Ar0=2rt−r∞Br0−rt−r∞Cr0=rt−2r∞Dr0=rt+r∞
Show that – a is the inverse of a for the addition operation '+' on R and 1a is the inverse of a≠0for the multiplication operation X on R.
View SolutionTwo satellite A and B are travelling in the same plane and same sense in circular orbit around the earth at an atitude rA and rB from the centre of the earth respectively. If at time t=0 the satellite are aligned as shown in the figure, knowing that the radius of the earth R. Determine the time at which again the satellite will be aligned as they were at t=0
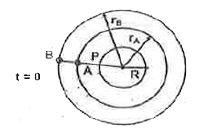
View SolutionIf nPr−1a=nPrb=nPr+1c," then which of the following holds good
View SolutionThe inverison of a sugar follows first order rate equation which can be followed by noting the change in the rotation of the plane of polarization of light in the polarimeter. If r∞,rf and r0 are the rotations at t=∞,t=t, and t=0, then the first order reaction can be written as
View SolutionThe concentric, thin metallic spheres of radii r1 and r2(r1>r2) carry charges q1 and q2 respectively. Then the electric potential at distance r(r2<r<r1) will be 14πε0 times
View Solution
