The reaction of A2 and B2 follows the equation
A2(g)+B2(g)→2AB(g)
The following data were observed
[A2]0[B2]0Initial rate of appearance of AB(g)(inMs−10.100.102.5×10−40.200.105×10−40.200.2010×10−4
The value of rate constatnt for the above reaction is :Solution in Telugu
2.5×10−4
2.5×10−2
1.25×10−2
None of these
The correct Answer is:C
Step by step video & image solution for The reaction of A_(2) and B_(2) follows the equation A_(2)(g)+B_(2)(g)to2AB(g) The following data were observed {:(,[A_(2)]_(0),[B_(2)]_(0),"Initial rate of appearance of AB(g)(in" Ms^(-1)),(,0.10,0.10,2.5xx10^(-4)),(,0.20,0.10,5xx10^(-4)),(,0.20,0.20,10xx10^(-4)):} The value of rate constatnt for the above reaction is : by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CHEMICAL KINETIC & NUCLEAR CHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - CHEMICAL KINETIC & NUCLEAR CHEMISTRYEXERCISE - Level 1 (Q.3 To Q.32)
2 Videos
- CHEMICAL KINETIC & NUCLEAR CHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - CHEMICAL KINETIC & NUCLEAR CHEMISTRYEXERCISE - Level 1 (Q.33 To Q.62)
3 Videos
- SURFACE CHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - SURFACE CHEMISTRYEXERCISE - Level 3 - Assertion - Reason Type Questions
1 Videos
Similar Questions
The reaction of A2 and B2 follows the equation
A2(g)+B2(g)→2AB(g)
The following data were observed
[A2]0[B2]0Initial rate of appearance of AB(g)(inMs−10.100.102.5×10−40.200.105×10−40.200.2010×10−4
The value of rate constatnt for the above reaction is :
View SolutionHalf-life (t1/2) and completion time (T) of the reaction (A→2B) are if initial concentration A is 1M and k = 0.001M/ min :
View Solutioneach question constain STATEMENT-1(Assertion ) and STATEMENT - 2 (reason). examine the statement carefully and work the correct answer accoridng to the instructions given below :
STATEMENT-1: Dispersed phase particles of colloidal solution cannot pass through ultra -filter paper.
STATEMENT-2: The size of colloidal particles are larger than the size of true solution particles.
View SolutionThe equilibrium constant for the reaction A_(2)(g)+B_(2)(g) hArr 2AB(g) is 20 at 500K . The equilibrium constant for the reaction 2AB(g) hArr A_(2)(g)+B_(2)(g) would be
View SolutionA_(2)+B_(2)to2AB , R.O.R.= k[A_(2)]^(a)[B_(2)]^(b) {:(,"Initial"[A_(2)],"Initial"[B_(2)],R.O.R.(r) Ms^(-1)),(,0.2,0.2,0.04),(,0.1,0.4,0.04),(,0.2,0.4,0.08):} Order of reaction with respect to A_( 2) and B_(2) are respectively :
View SolutionThe reaction of A_(2) and B_(2) follows the equation A_(2)(g)+B_(2)(g)to2AB(g) The following data were observed {:(,[A_(2)]_(0),[B_(2)]_(0),"Initial rate of appearance of AB(g)(in" Ms^(-1)),(,0.10,0.10,2.5xx10^(-4)),(,0.20,0.10,5xx10^(-4)),(,0.20,0.20,10xx10^(-4)):} The value of rate constatnt for the above reaction is :
View Solutionthe value of the determinant
∣∣ ∣ ∣ ∣ ∣∣(a1−b1)2(a1−b2)2(a1−b3)2(a1−b4)2(a2−b1)2(a2−b2)2(a2−b3)2(a3−b4)2(a3−b1)2(a3−b2)2(a3−b3)2(a3−b4)2(a4−b1)2(a4−b2)2(a4−b3)2(a4−b4)2∣∣ ∣ ∣ ∣ ∣∣ is
View SolutionConsider the reaction:
(i) A2(s)+B2(g)→A2(g)+B2(g),ΔH=−x kJ
(ii) A2(g)+B2(g)→2AB(g),ΔH=−y kJ
What is the value of the change in enthalpy for the following reaction A2(s)+B2(g)→2AB(g) ?
View SolutionThe equilibrium constant (Kc) of the reaction A2(g)+B2(g)→2AB(g) is 50. If 1 mol of A2 and 2 mol of B2 are mixed, the amount of AB at equilibrium would be
View SolutionFor the Chemical reaction A2(g)+B2(g)⇔ 2 AB(g) the amount of AB at equilibrium is affected by
View Solutionthe value of the determinant
∣∣ ∣ ∣ ∣ ∣∣(a1−b1)2(a1−b2)2(a1−b3)2(a1−b4)2(a2−b1)2(a2−b2)2(a2−b3)2(a2−b4)2(a3−b1)2(a3−b2)2(a3−b3)2(a3−b4)2(a4−b1)2(a4−b2)2(a4−b3)2(a4−b4)2∣∣ ∣ ∣ ∣ ∣∣ is
View SolutionThe reaction of A2 and B2 follows the equation
A2(g)+B2(g)→2AB(g)
The following data were observed
[A2]0[B2]0Initial rate of appearance of AB(g)(inMs−10.100.102.5×10−40.200.105×10−40.200.2010×10−4
The value of rate constatnt for the above reaction is :
View SolutionAt constant temperature 80% AB dissociates into A_(2) and B_(2) then the equilibrium constant for 2AB_((g)) harr A_(2(g)) +B_(2(g))
View Solution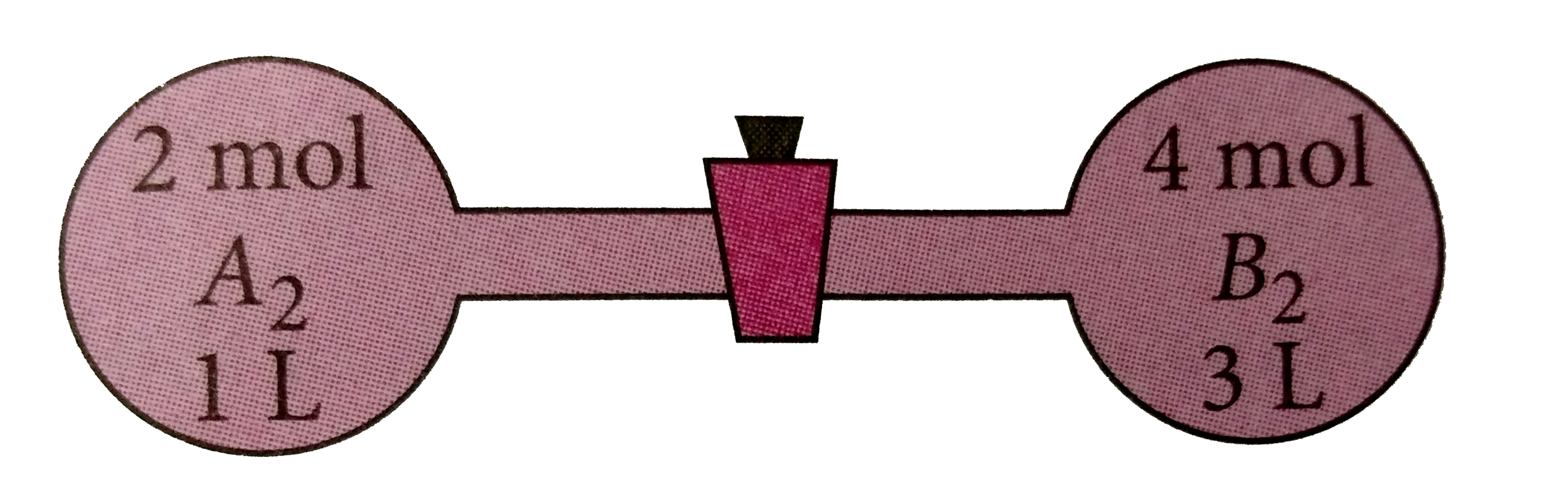
When A2andB2 are allowed to react, the equilibrium constant of the reaction at 27∘C is found (Kc=4).
A2(g)+B2(g)⇔2AB(g)
what will be the equilibrium concentration of AB?
View SolutionThe equilibrium constant for the reaction A_(2)(g)+B_(2)(g) hArr 2AB(g) is 20 at 500K . The equilibrium constant for the reaction 2AB(g) hArr A_(2)(g)+B_(2)(g) would be
View SolutionThe equilibrium constant (K_(c)) of the reaction A_(2)(g) + B_(2)(g) rarr 2AB(g) is 50. If 1 mol of A_(2) and 2 mol of B_(2) are mixed, the amount of AB at equilibrium would be
View Solution
