A block of mass m is kept on the edge of a horizontal turn table of radius R which is rotating with constant angular verticle ω (along with the block ) about its axis . If coefficient of friction is μ, find the friction force between block and table.
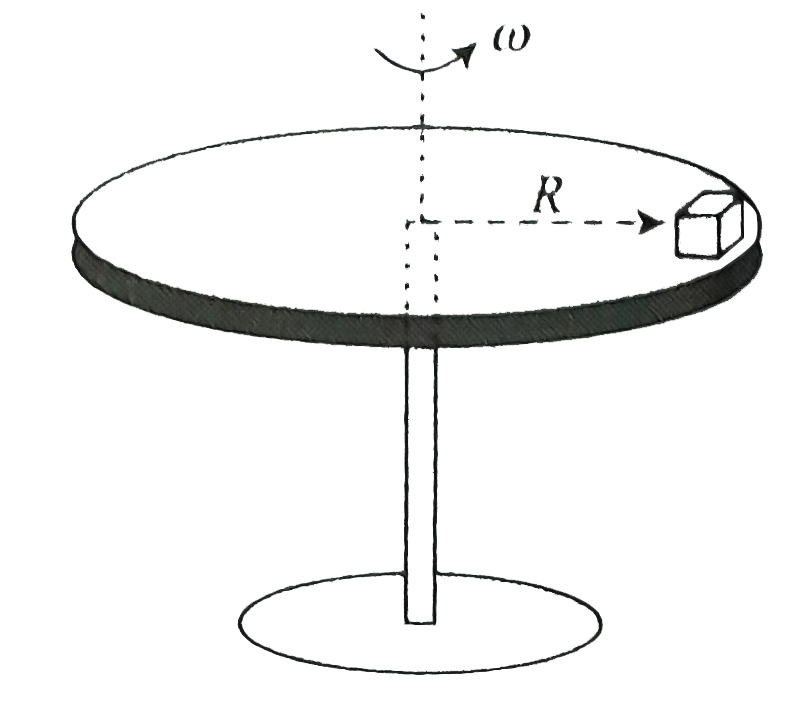
The block in Fig does not accelerate vertically. Therefore , we model it as a particle in equilibrum in the verticle direction if centripetal acceleration in the horizontal direction in this case centriprted is proveded by friction.
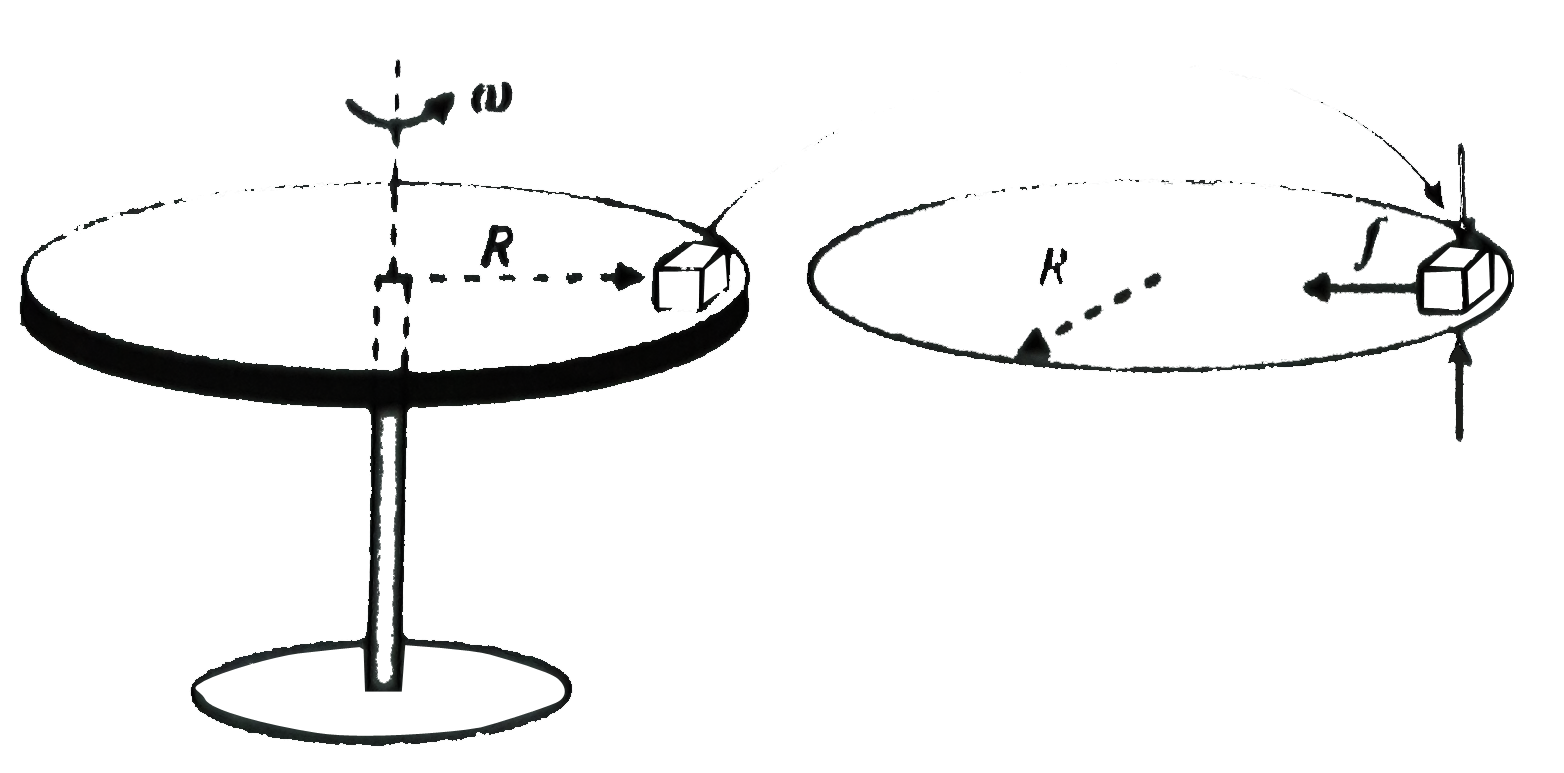
∑Fy=N−mg=0...(i)
N=mg....(ii)
∑Fx=f=mac=mω2R
Friction force = centripental force =mω2R
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Solved Examples12videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.125videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer5videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
Similar Questions
A block of mass m is kept on a horizontal table. If the static friction coefficient is μ., find the frictional force acting on the block.
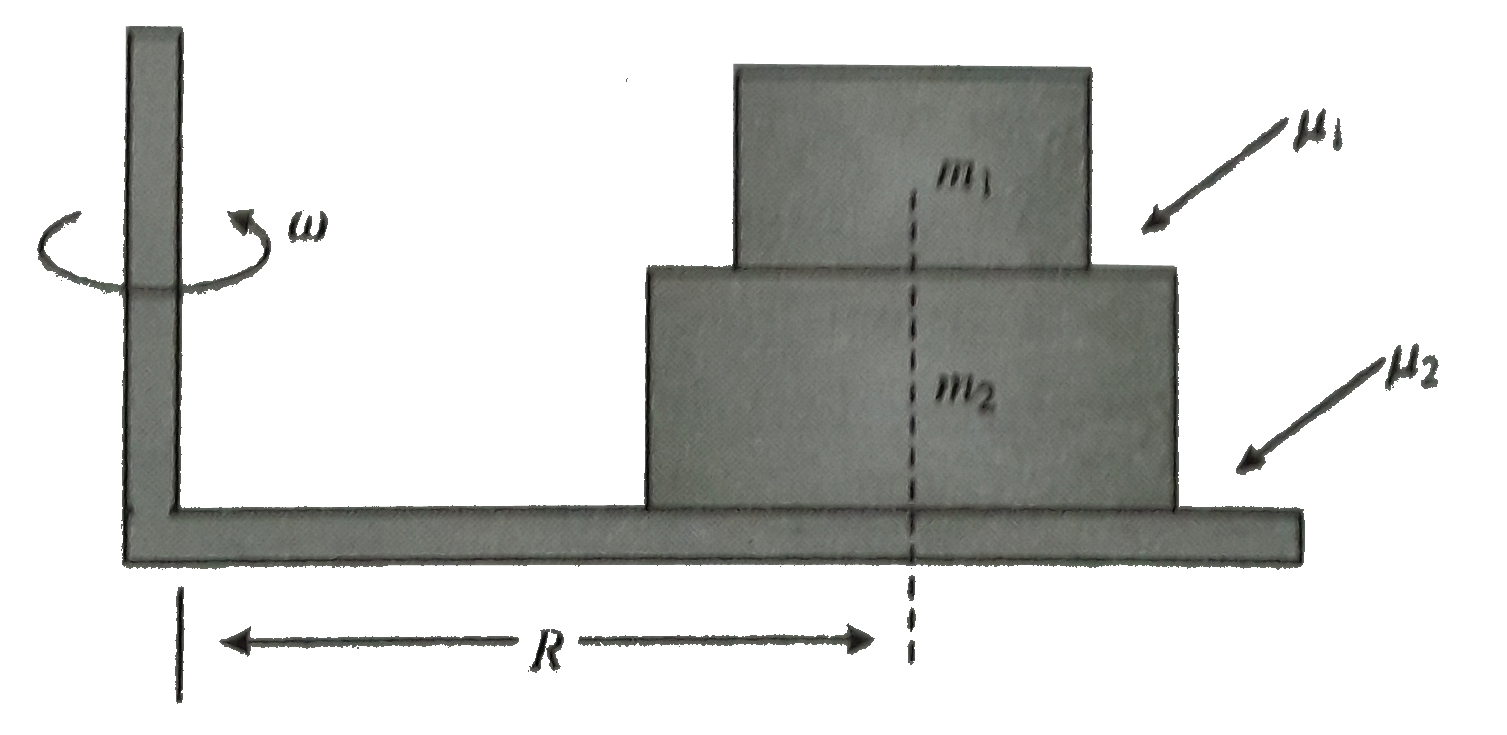
View SolutionThere are two blocks of masses m1 and m2,m1 is placed on m2 on a table which is rotating with an angular velocity ω about the vertical axis . The coefficent of friction between the block is μ1 and between m2 and table is μ2(μ1<μ2) if block are placed at distance R from the axis of ratation , for relative sliding between the surface in contact , find the
a. friction force at the contacting surface
b. maximum angular speed ω
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
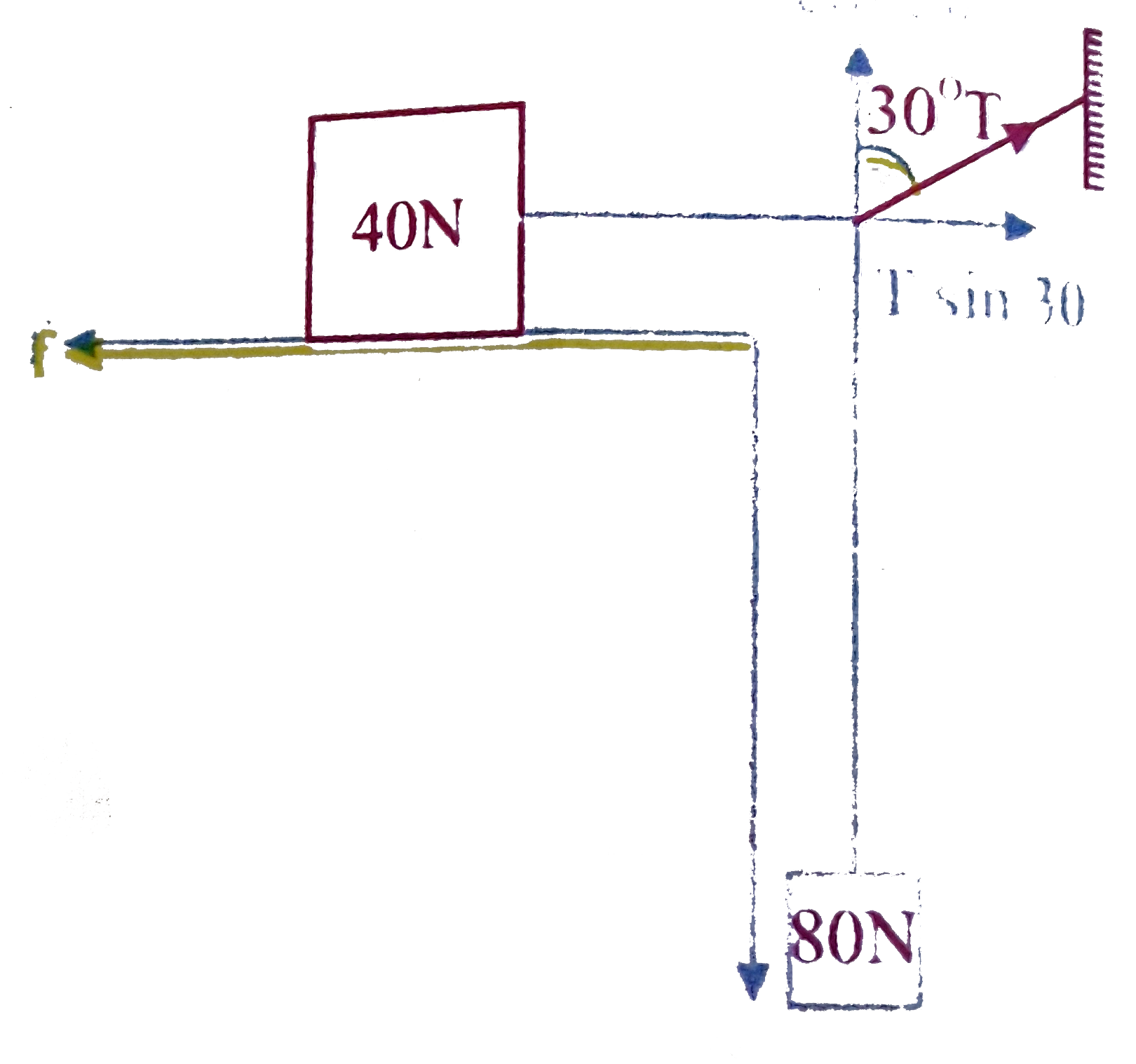
If coefficient of friction between both the block is μ−12 . Find friction force acting between both block.
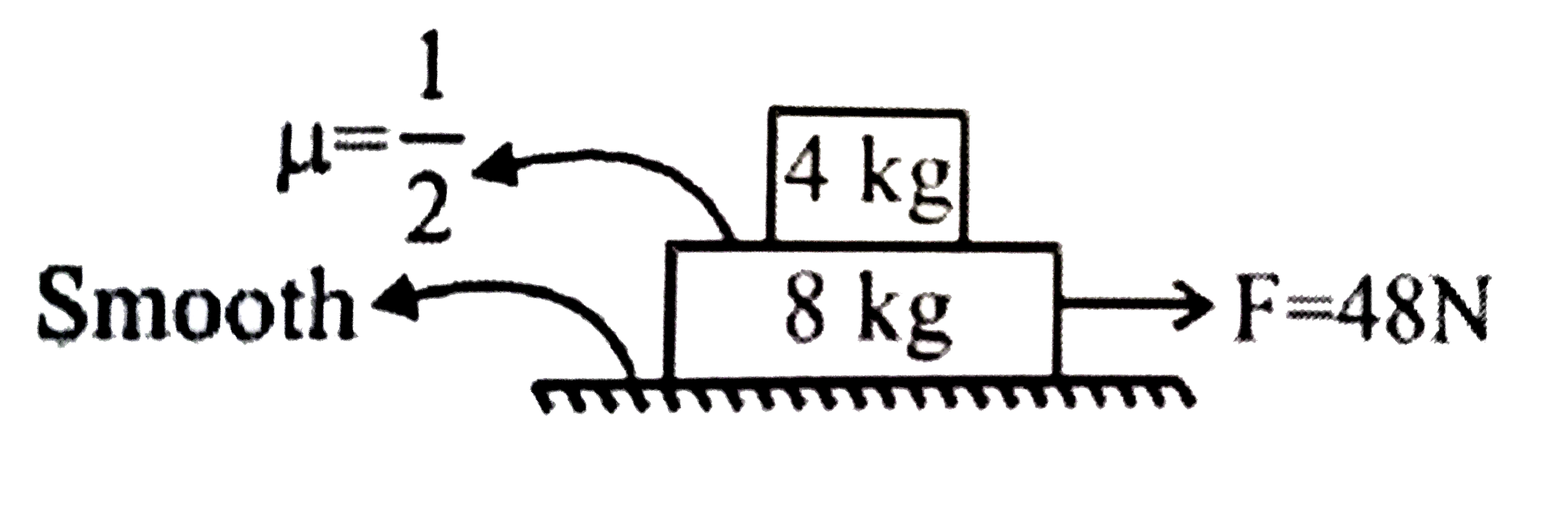 A16NB20NC8ND10N
A16NB20NC8ND10N - Question 2 - Select One
A ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
Aω0μkR2gBω0g2μkRC2ω0RμkgDω0R2μkg - Question 3 - Select One
A block A of mass m1 rests on a horizontal table. A light string connected to it passes over a frictionless pully at the edge of the table and from its other end another block B of mass m2 is suspended. The coefficient of kinetic friction between the block and the table is H. When the block A is sliding on the table, the tension in the string is
Am1m2(1+μk)g(m1+m2)Bm1m2(1−μk)g(m1)+m2C(m2+μkm1)g(m1+m2)D(m2−μkm1)g(m1+m2)
A particle of mass m is placed over a horizontal circular table rotating with an angular velocity ω about a vertical axis passing through its centre. The distance of the object from the axis is r. Find the force of friction f between the particle and the table.
View SolutionA block on table shown in is just on the edge of slipping Find the coefficient of static friction between the blocks and table
 .View Solution
.View SolutionA block 'A' of mass 2m is placed on another block 'B' of mass 4m which in turn is placed on a fixed table. The two blocks have a same length 4d and they are placed as shown in fig The coefficient of friction (both static and kinetic) between the block 'B' and table is μ. There is no friction between the two blocks. A small object of mass m moving horizontally along a line passing through the centre of mass (cm.) of the block B and perpendicular to its face with a speed v collides elastically with the block B at a height d above the table.
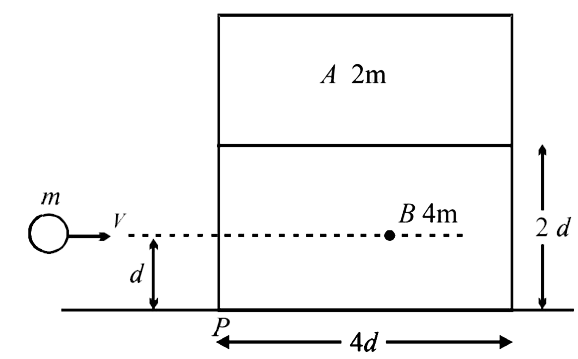
(a) What is the minimum value of v(call it v0) required to make the block A topple?
(b) If v=2v0,find the distance (from the point P in the figure ) at which the mass m falls on the table after collision. (Ignore the role of friction during the collision).View SolutionA horizontal force of 20 N i sapplied to a block of mass 4 kg resting on a rough horiozontal table. If the blcok does not move on the table, how much fricrtional force the table is applying on the blockgt What can be said about the coefficient of static friction between the block and the table? Takeg=ms2.
View SolutionA block of mass m is pulled along a horizontal surface by applying a force at an angle θ with the horizontal. If the block travels with a uniform velocity and has a displacement d and the coefficient of friction is μ, then the work done by the applied force is
View Solution

