A bob A of a simple pendulum is released when the string makes an angle of 45∘ with the vertical. It hits another bob B of the same material and same mass kept at rest on the table. If the collision is perfectly elastic then

B will rise to a height equal to A and A comes to rest
B moves first and A follows it with half of its initial velocity
both A and B come to rest at B
both A and B move with the same velocity of A
The correct Answer is:A
The masses of the three parts will be 1kg, 1 kg and 3kg respectively.
The two parts with masses 1 kg and 1kg move in perpendicular directions.
∴ Resultant momentum .
=√(1×30)2+(1×30)2=√1800=30√2
and the moemntum of the bigger part =3×v
By the principle of conservation of linear momentum, momentum before collison = 0 .
and Momentum after collision =30√2+3v
∴30√2+3v=0 ∴3v=−30√2
∴v=−10√2m/s
∴ The magnitude of the velocity =10√2m/s
Thus the third part moves in a direction opposite to the direction of the resultant of the two perpendicular momenta.
Topper's Solved these Questions
Similar Questions
The bob A of a simple pendulum is released when the string makes an angle of 45∘ with the vertical. It hits another bob B of the same material and same mass kept at rest on the table. If the collision is elastic, then
 View Solution
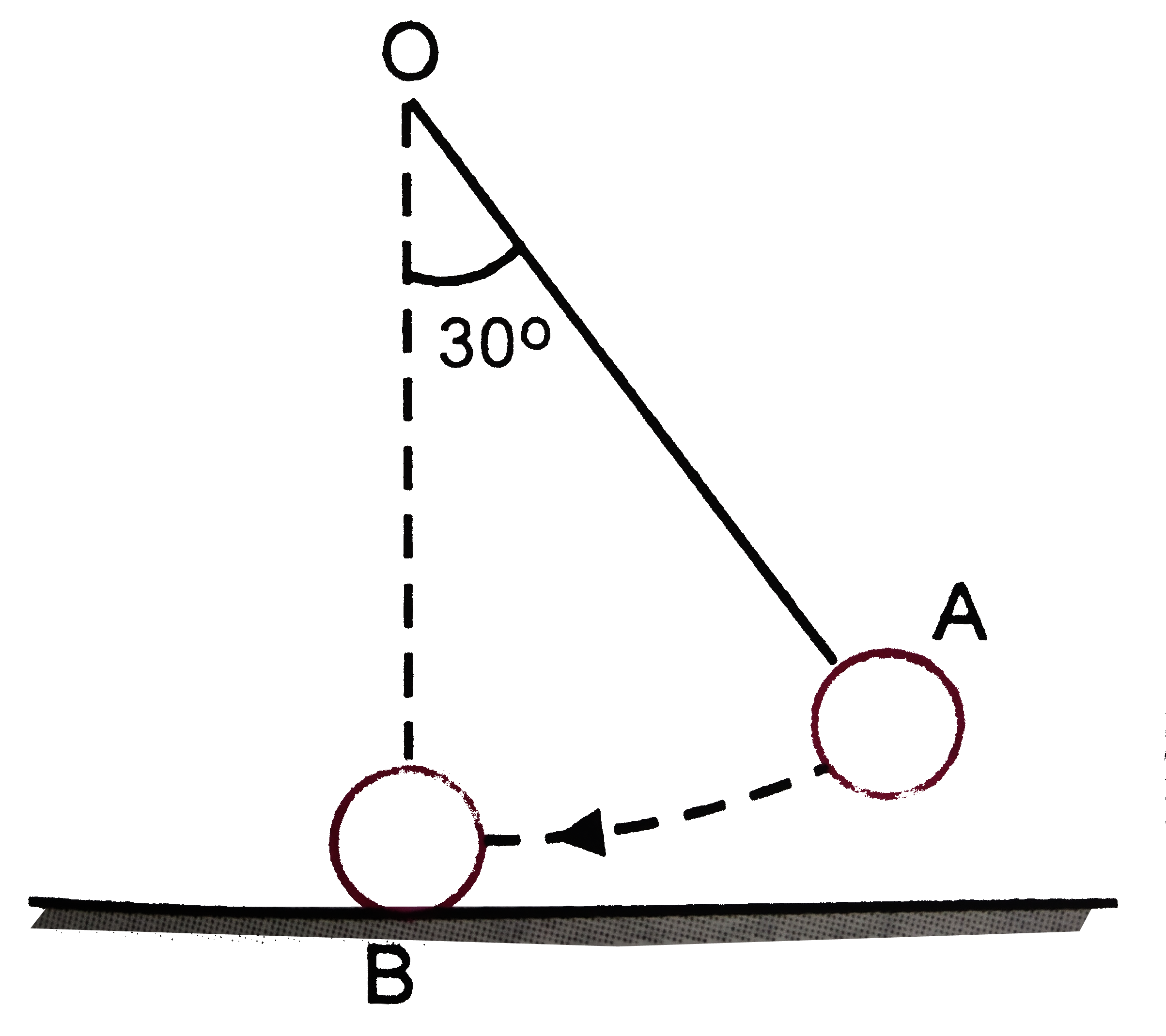
View SolutionThe bob A of a simple pendulum released from 30∘ to the vertical hits another bobo B of the same mass at rest on a table as shown in figure. How high does the bob A rise after the collision ? Neglect the size of the bobs and assume the collision to be elastic.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The bob A of a simple pendulum is released when the string makes an angle of 45∘ with the vertical. It hits another bob B of the same meterial and same mass kept at rest on the table. If the collision is elastic, then
 ABoth AandB rise to the same heightBBoth AandB comes to rest at BCBoth AandB move with the same velocity of ADA comes to rest and B move with the same velocity of A
ABoth AandB rise to the same heightBBoth AandB comes to rest at BCBoth AandB move with the same velocity of ADA comes to rest and B move with the same velocity of A - Question 2 - Select One
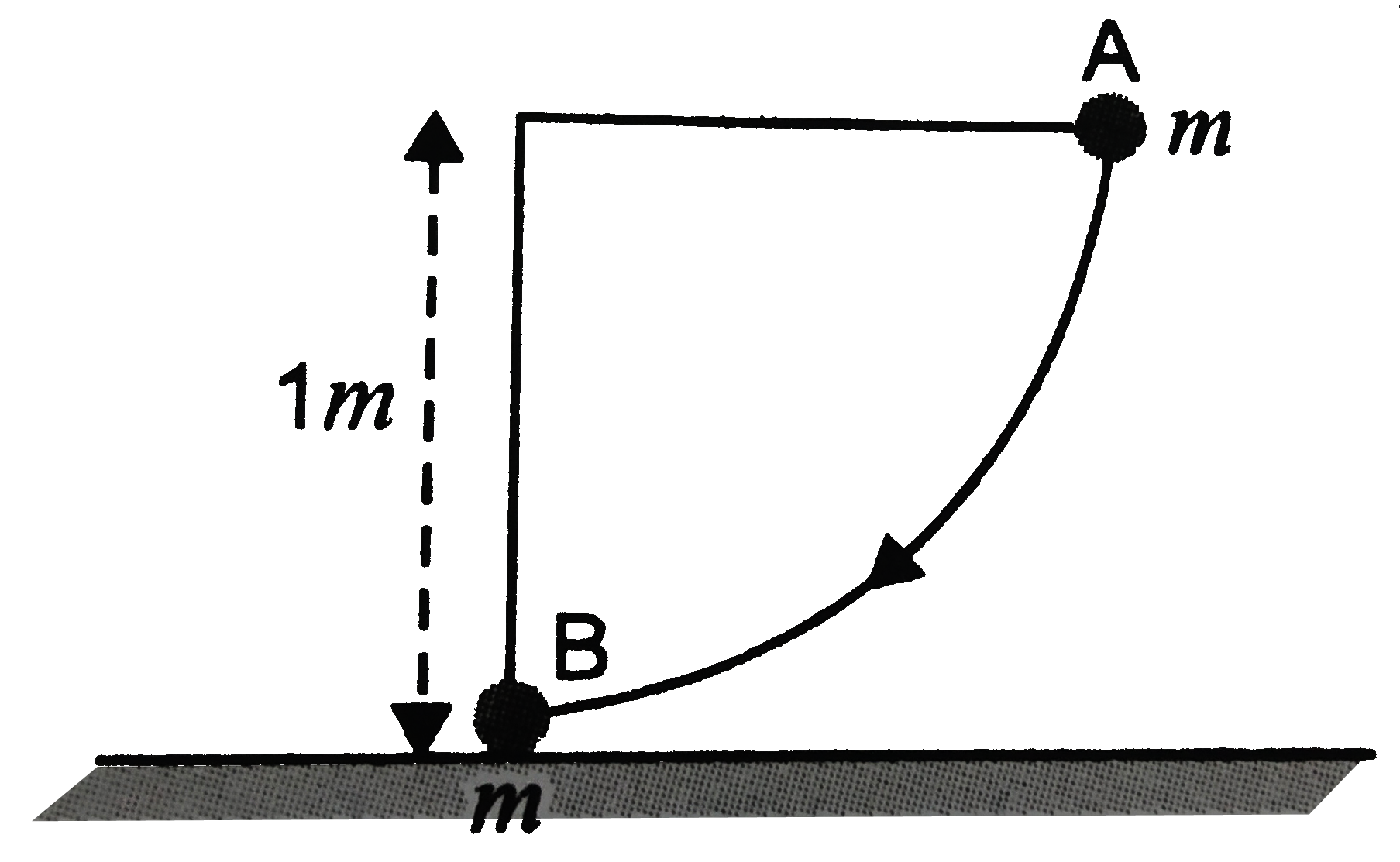
The bob A of a pendulum released from horizontal to the vertical hits another bob B of the same mass at rest on a table as shown in figure.
If the length of the pendulum is 1m, calculate
(a) the height to which bob A will rise after collision.
(b) the speed with which bob B starts moving.
Neglect the size of the bobs and assume the collision to be elastic.
 A4.47ms−1B5.47ms−1C6.47ms−1D3.47ms−1
A4.47ms−1B5.47ms−1C6.47ms−1D3.47ms−1
The bob A of a simple pendulum released from 30∘ to the vertical hits another bobo B of the same mass at rest on a table as shown in figure. How high does the bob A rise after the collision ? Neglect the size of the bobs and assume the collision to be elastic.
 View Solution
View SolutionA nail is located at a certain distance vertically below the point of suspension of a simple pendulum. The pendulum bob is released from a position where the string makes an angle of 60∘ with the vertical. Calculate the distance of nail from the point of suspension such that the bob will just perform revolutions with the nail as centre. Assume the length of the pendulum to be one meter.
View SolutionThe bob of a pendulum of length 980 cm is released from rest with its string making an angle 60∘ with the vertical. It collides with a ball of mass 20 gm resting on a smooth horizontal surface just at the position of rest of the pendulum. With what velocity will the bob move immediately after the collision? What will be the maximum angular displacement of the bob after them collision ? The mass of the bob is 10 gm. '
View SolutionThe bob A of a pendulum released from horizontal to the vertical hits another bob B of the same mass at rest on a table as shown in figure.
If the length of the pendulum is 1m, calculate
(a) the height to which bob A will rise after collision.
(b) the speed with which bob B starts moving.
Neglect the size of the bobs and assume the collision to be elastic.
 View Solution
View SolutionThe bob A of a pendulum released from horizontal to the vertical hits another bob B of the same mass at rest on a table as shown in figure.
If the length of the pendulum is 1m, calculate
(a) the height to which bob A will rise after collision.
(b) the speed with which bob B starts moving.
Neglect the size of the bobs and assume the collision to be elastic.
 View Solution
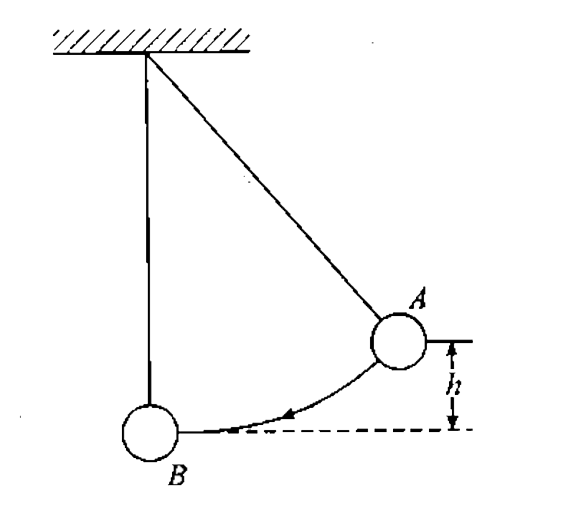
View SolutionThe bob A of a pendulum released from a height h hits head-on another bob B of the same mass of an identical pendulum initially at rest. What is the result of this collision? Assume the collisio to be elastic (see figure).
 View Solution
View Solution

