A boby is moving in a room with a velocity of 20m/s perpendicular to the two walls separated by 5 meters .There is no friction and the collisionnn with the walls are elastic.
Not periodic
Periodic but not smple harmonic
Periodic and simple harmonic
Periodic with variable time period
The correct Answer is:B
To solve the problem, we need to analyze the motion of the body as it collides with the walls in the room. Here’s a step-by-step solution:
Step 1: Understand the Setup
- The body is moving with a velocity of 20m/s perpendicular to two walls that are 5m apart.
- The collisions with the walls are elastic, meaning the body will bounce back with the same speed after hitting the walls.
Step 2: Calculate the Time Taken for One Round Trip
- The body travels 5m to the first wall and then 5m back to the starting point after colliding with the second wall.
- The total distance for one complete round trip is:
Total Distance=5m+5m=10m
- The time taken to travel this distance at a speed of 20m/s can be calculated using the formula:
Time=DistanceVelocity=10m20m/s=0.5s
Step 3: Determine the Nature of Motion
- Since the body returns to its starting position after 0.5s, this indicates that the motion is periodic.
- However, for the motion to be classified as Simple Harmonic Motion (SHM), it must satisfy the condition that the acceleration is directly proportional to the displacement from the mean position and directed towards it.
Step 4: Analyze if the Motion is SHM
- In this case, while the motion is periodic (the body keeps bouncing between the walls), it does not have a restoring force that is proportional to the displacement from a mean position (as required for SHM).
- Therefore, the motion is periodic but not SHM.
Conclusion
- The correct classification of the motion is that it is periodic but not simple harmonic motion (SHM).
Final Answer
The motion of the body is periodic but not SHM.
---
- The body is moving with a velocity of 20m/s perpendicular to two walls that are 5m apart.
- The collisions with the walls are elastic, meaning the body will bounce back with the same speed after hitting the walls.
- The body travels 5m to the first wall and then 5m back to the starting point after colliding with the second wall.
- The total distance for one complete round trip is:
Total Distance=5m+5m=10m
- The time taken to travel this distance at a speed of 20m/s can be calculated using the formula:
Time=DistanceVelocity=10m20m/s=0.5s
- Since the body returns to its starting position after 0.5s, this indicates that the motion is periodic.
- However, for the motion to be classified as Simple Harmonic Motion (SHM), it must satisfy the condition that the acceleration is directly proportional to the displacement from the mean position and directed towards it.
- In this case, while the motion is periodic (the body keeps bouncing between the walls), it does not have a restoring force that is proportional to the displacement from a mean position (as required for SHM).
- Therefore, the motion is periodic but not SHM.
- The correct classification of the motion is that it is periodic but not simple harmonic motion (SHM).
The motion of the body is periodic but not SHM.
Topper's Solved these Questions
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Multiple Correct Answer Type
5 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Multiple Correct Answer Type
9 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Subjective type
2 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
Similar Questions
A block slides on ice with a velocity of 5m/s comes to rest after moving through a distance of 13.5m . Find the coefficient of friction ?
View SolutionA bead of mass m and diameter d is sliding back and forth with velocity v on a wire held between two right walls of length L. Assume that th ecollisions with the wall are perfectly elastic and ther is no friction. The average force that the bouncing bead exerts on the one of the walls is :_
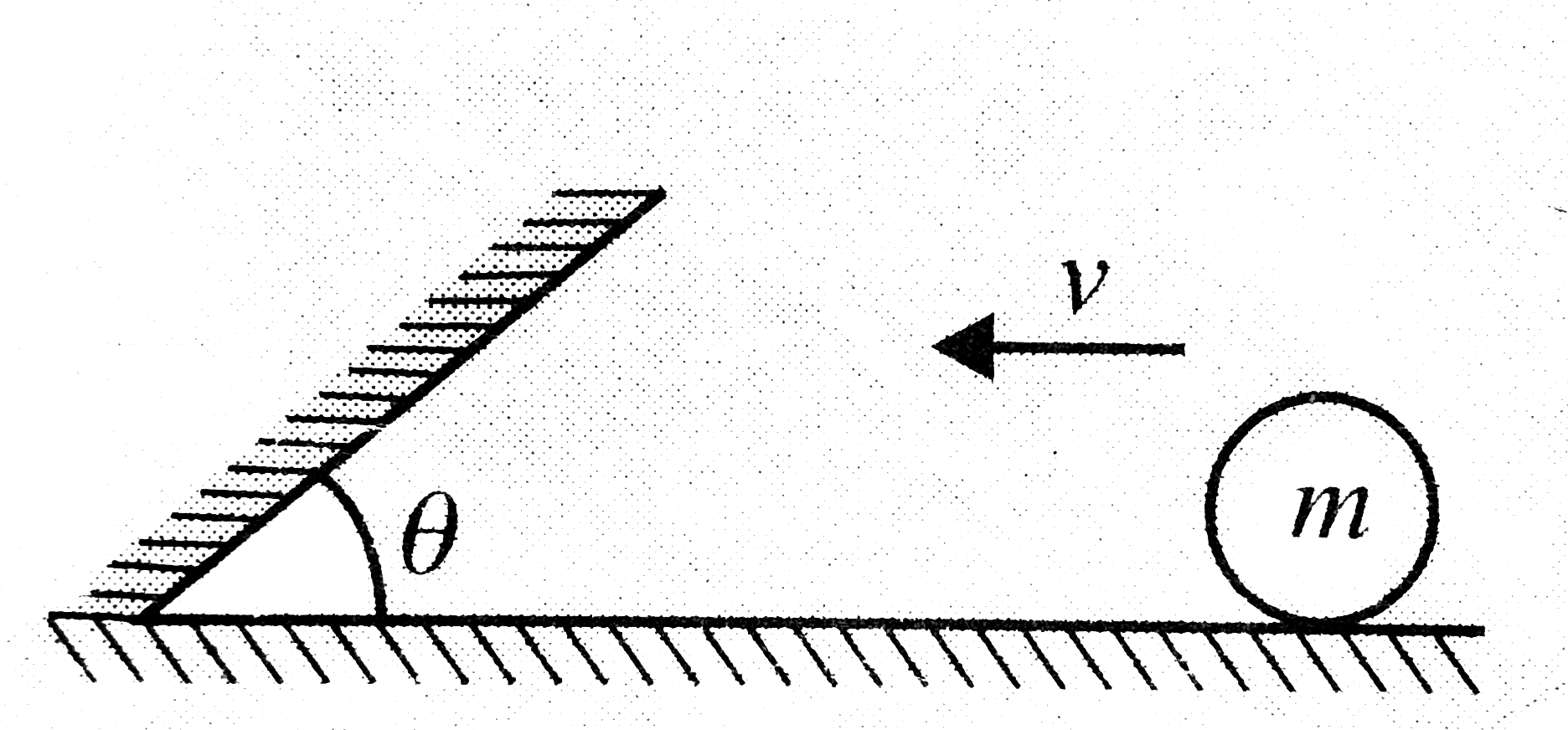
View SolutionA sphere of mass m slides with velocity v on as frictionless surface towards a smooth inclined wall as shown in figure. If the collision with the wall is perfectly elastic find a. the impulse imparted by the wall on the sphere b the impulse imparted by the floor on the sphere.
View SolutionA parallel beam of particles each of mass m moving with velocity v impinges on a wall at an angle θ to its normal. The number of particles per unit volume in the beam is n. If the collision of particles with the wall is elastic, then the pressure exerted by this beam on the wall is
View SolutionA cart is moving with a velocity 20m/s. Sand is being dropped into the cart at the rate of 50 kg/min. The force required to move the cart with constant velocity will be :-
View SolutionA ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
View SolutionA ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
View Solution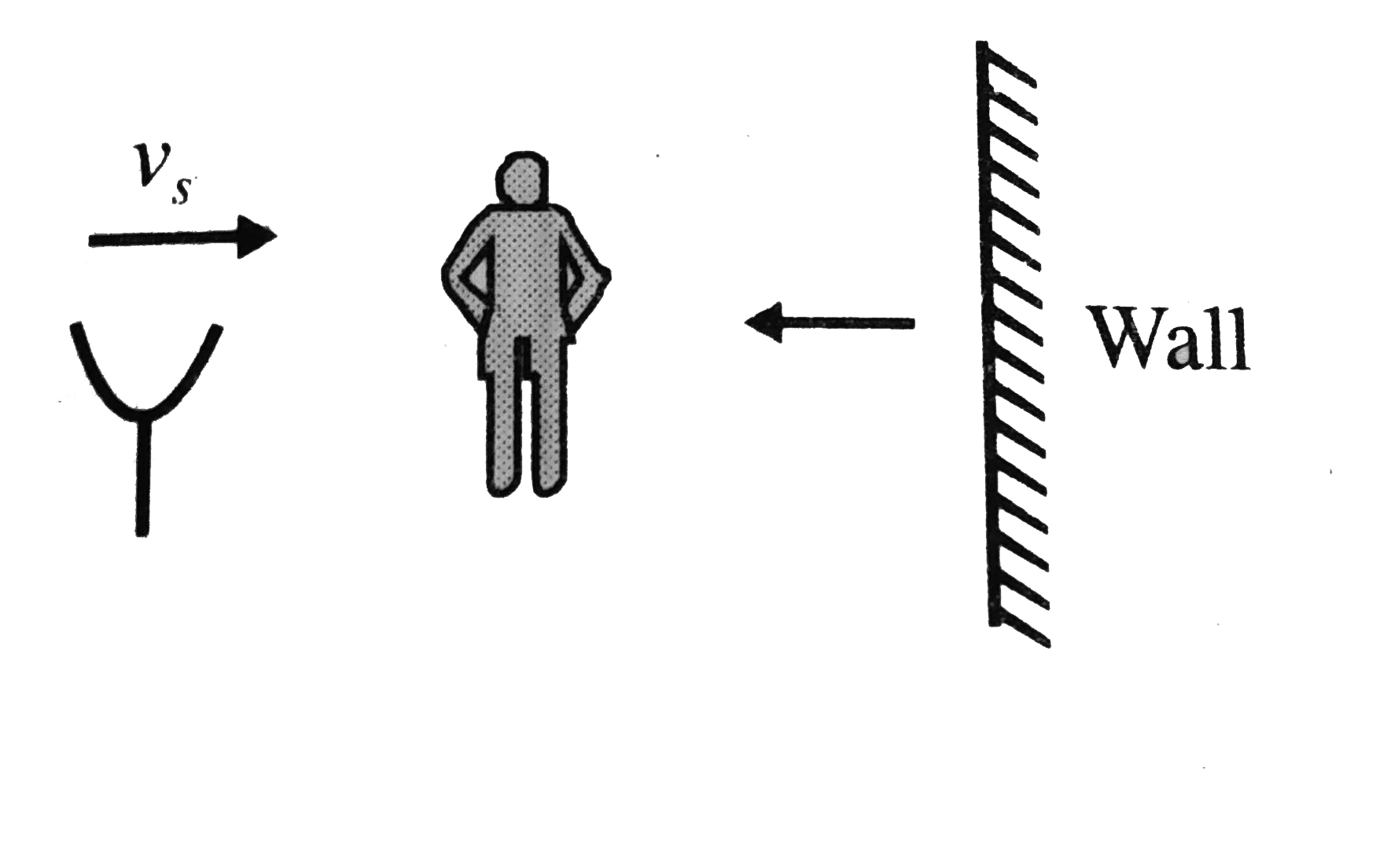
A tuning fork of frequency 380 Hz is moving towards a wall with a velocity of 4ms Then the number of beats heard by a stationary listener between direct and reflected sound will be (velocity of sound in air is 340ms)
View SolutionA train moving with a velocity of 20 m s−1' is brought to rest by applying brakes in 5 s. Calculate the retardation.
View SolutionA molecule of mass m moving at a velocity v impinges elastically on the wall at an angle α with the wall. Then
View Solution
