A body of mass 10 gm and having charge 2C is attached to a spring which is suspended from the ceiling. It vibrates with a time period 1 sec. If an electric field of intensity 100 N/C is now applied in the downward direction, find the time period.
The correct Answer is:1 sec
To solve the problem, we need to determine the time period of a mass-spring system when an electric field is applied. The key points to consider are the mass of the body, the charge, the initial time period, and the effect of the electric field.
1. Identify the Given Values:
- Mass of the body, m=10g=10×10−3kg=0.01kg
- Charge of the body, q=2C
- Initial time period, Ti=1s
- Electric field intensity, E=100N/C
2. Understand the Time Period Formula:
The time period T of a mass-spring system is given by the formula:
T=2π√mk
where k is the spring constant.
3. Consider the Effect of the Electric Field:
When an electric field is applied, it exerts a force on the charged body given by:
Fe=qE
This force acts in the downward direction, adding to the gravitational force Fg=mg.
4. Calculate the Forces Acting on the Mass:
The total effective force acting on the mass when the electric field is applied is:
Ftotal=mg+qE
However, this does not affect the time period directly since the time period depends only on the mass and the spring constant.
5. Determine the Time Period After Applying the Electric Field:
The time period is independent of the forces acting on the mass (as long as the spring remains linear). Therefore, the time period remains the same:
Tf=Ti=1s
Final Answer:
The time period of the mass-spring system after applying the electric field remains Tf=1s.
---
- Mass of the body, m=10g=10×10−3kg=0.01kg
- Charge of the body, q=2C
- Initial time period, Ti=1s
- Electric field intensity, E=100N/C
The time period T of a mass-spring system is given by the formula:
T=2π√mk
where k is the spring constant.
When an electric field is applied, it exerts a force on the charged body given by:
Fe=qE
This force acts in the downward direction, adding to the gravitational force Fg=mg.
The total effective force acting on the mass when the electric field is applied is:
Ftotal=mg+qE
However, this does not affect the time period directly since the time period depends only on the mass and the spring constant.
The time period is independent of the forces acting on the mass (as long as the spring remains linear). Therefore, the time period remains the same:
Tf=Ti=1s
The time period of the mass-spring system after applying the electric field remains Tf=1s.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-I)13videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Exercise57videos
ELECTRIC CHARGES AND FIELDS
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Additional Exercise11videos
ELECTROMAGNETIC INDUCTION
Similar Questions
A bob of a simple pendulum of mass 40 mg with a positive charge 4×10−6C is oscilliating with time period T1. An electric field of intensity 3.6×104 N/c is applied vertically upwards now time period is T2. The value of T2T1 is (g=10ms2)
View SolutionA body of mass m attached to a spring which is oscillating with time period 4 s. If the mass of the body is increased by 4 kg, its time period increases by 2 s. Determine value of initial mass m.
View SolutionA particle of mass 'm' and charge 'q' is suspended from the ceiling with the help of an insulating wire of length 'l'. It is placed in an uniform electric field as shown in figure. Then the time period of oscillation is
_2019_CBT6_E01_041_Q01.png) View Solution
View SolutionA water particle of mass 10.0 mg and having a charge of 1.50×10−6C stays suspended in a room. What is the magnitude of electric field in the room ? What is its direction?
View SolutionA block of mass m is suspended vertically with a spring of spring constant k. The block is made to oscillate in a gravitation field. Its time period is fould to be T. Now the space between the plates is made gravity free, and an electric field E is produced in the downward direction. Now the block is given a charge q. the new time period of oscillation is
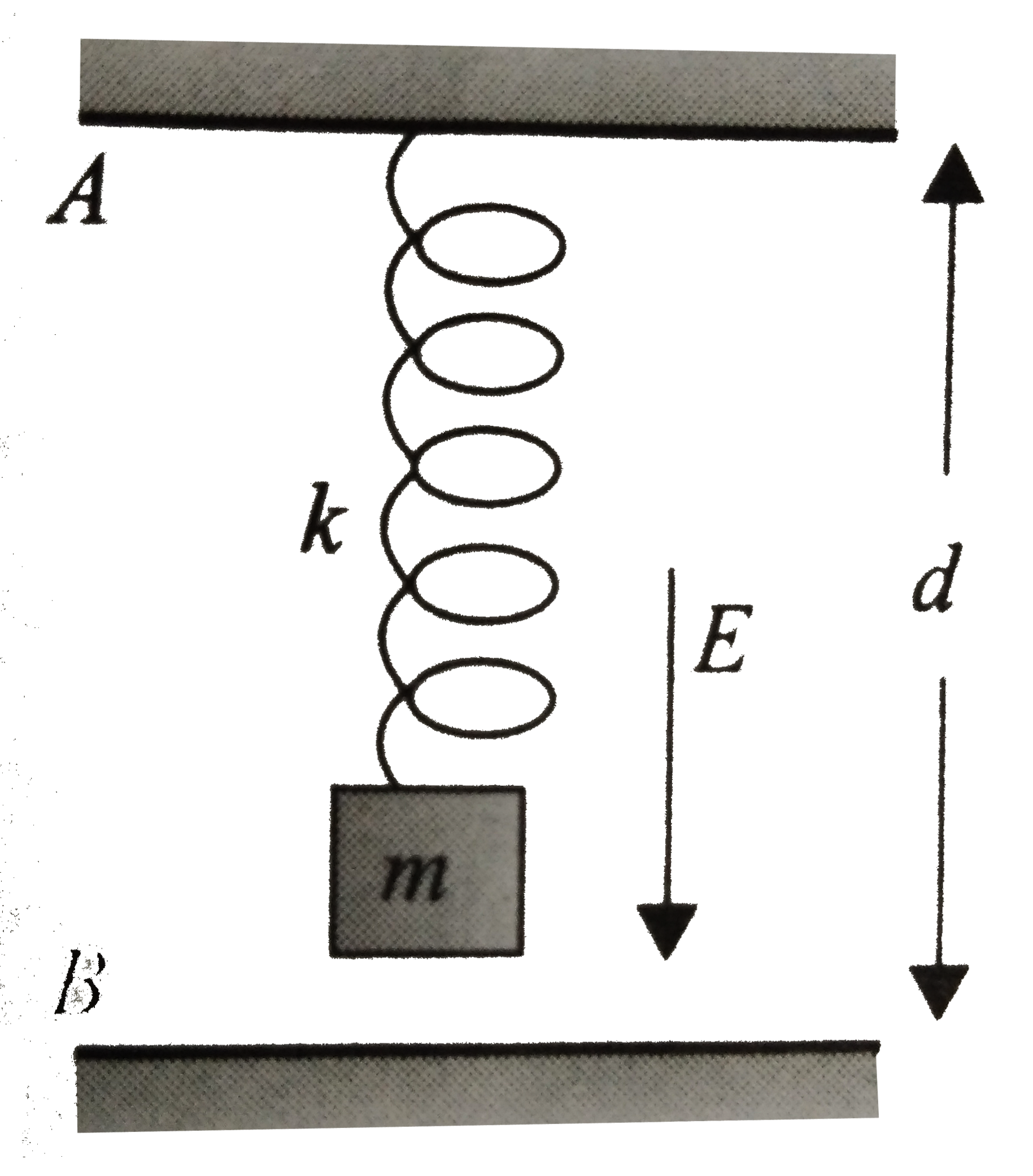 View Solution
View SolutionA body of mass 1kg suspended an ideal spring oscillates up and down. The amplitude of oscillation is 1 metre and the periodic time is 1.57 second. Determine.
View SolutionA force of 6.4 N stretches a vertical spring by 0.1 m. The mass (in kg) that must be suspended from the spring so that it oscillates with a time period of π4 second.
View SolutionA 0.50 gm ball carries a charge of magnitude 10μC. It is suspended by a string in a downward electric field of intensity 300N/C. If the charge on the ball is positive, then the tension in the string is (g=10ms−2)
View SolutionA charged oil drop weighing 1.6×10−15N is found to remain suspended in a uniform electric field of intensity 2×103NC−1. Find the charge on the drop.
View SolutionA block of mass 100g attached to a spring of stiffness 100N/m is lying on a frictionless floor as shown. The block is moved to compress the spring by 10cm and released. If the collision with the wall is elastic then find the time period of oscillations.
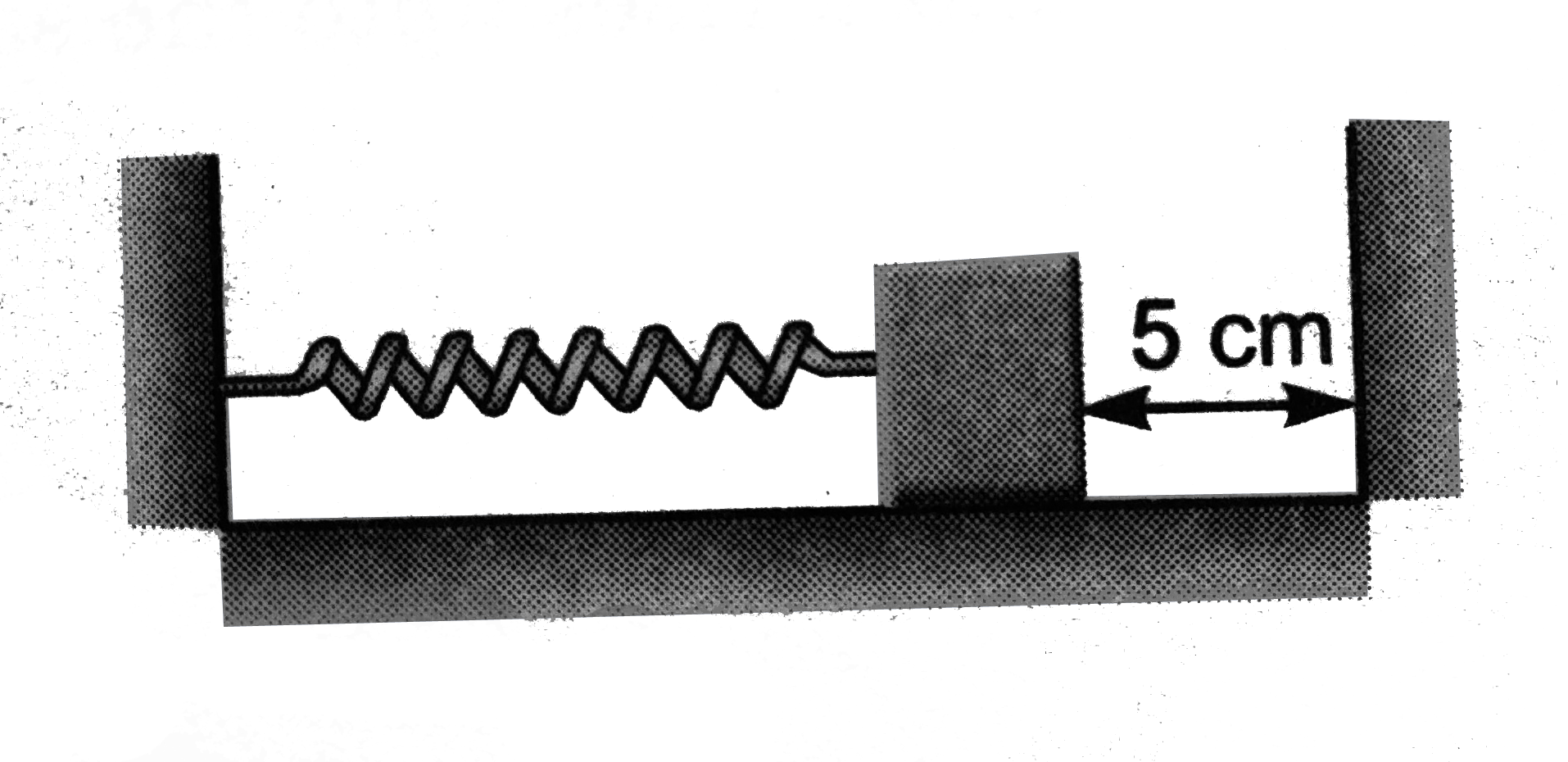 View Solution
View Solution
