A charged particle of mass m = 2 kg and charge 1μC is projected from a horizontal ground at an angle θ=45∘ with speed 10ms−1. In space, a horizontal electric field towards the direction of projection E=2×107NC−1 exists. The range of the projectile is
20 m
60 m
200 m
180 m
The correct Answer is:A
To solve the problem, we need to find the range of a charged particle projected in the presence of an electric field. Here’s a step-by-step solution:
Step 1: Identify the given values
- Mass of the particle, m=2kg
- Charge of the particle, q=1μC=1×10−6C
- Initial speed, u=10m/s
- Angle of projection, θ=45∘
- Electric field, E=2×107N/C
- Acceleration due to gravity, g=10m/s2
Step 2: Calculate the time of flight
The time of flight T for a projectile is given by the formula:
T=2usinθg
Substituting the values:
T=2×10×sin(45∘)10
Since sin(45∘)=1√2:
T=2×10×1√210=2√2=√2s
Step 3: Calculate the horizontal acceleration due to the electric field
The horizontal acceleration ax can be calculated using the formula:
ax=qEm
Substituting the values:
ax=(1×10−6)×(2×107)2=2×1012=10m/s2
Step 4: Calculate the horizontal component of the initial velocity
The horizontal component of the initial velocity ux is given by:
ux=ucosθ
Substituting the values:
ux=10cos(45∘)=10×1√2=10√2m/s
Step 5: Calculate the range
The range R can be calculated using the formula:
R=uxT+12axT2
Substituting the values:
R=(10√2)⋅√2+12⋅10⋅(√2)2
This simplifies to:
R=10+12⋅10⋅2=10+10=20m
Final Answer
The range of the projectile is R=20m.
---
- Mass of the particle, m=2kg
- Charge of the particle, q=1μC=1×10−6C
- Initial speed, u=10m/s
- Angle of projection, θ=45∘
- Electric field, E=2×107N/C
- Acceleration due to gravity, g=10m/s2
The time of flight T for a projectile is given by the formula:
T=2usinθg
Substituting the values:
T=2×10×sin(45∘)10
Since sin(45∘)=1√2:
T=2×10×1√210=2√2=√2s
The horizontal acceleration ax can be calculated using the formula:
ax=qEm
Substituting the values:
ax=(1×10−6)×(2×107)2=2×1012=10m/s2
The horizontal component of the initial velocity ux is given by:
ux=ucosθ
Substituting the values:
ux=10cos(45∘)=10×1√2=10√2m/s
The range R can be calculated using the formula:
R=uxT+12axT2
Substituting the values:
R=(10√2)⋅√2+12⋅10⋅(√2)2
This simplifies to:
R=10+12⋅10⋅2=10+10=20m
The range of the projectile is R=20m.
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - Assertion and Reason Type
8 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - Comprehension Type
94 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Multiple Correct Answer type
2 Videos
- MISCELLANEOUS VOLUME 5BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Integer
12 Videos
Similar Questions
A charged particle of mass m=1 kg and charge q=2μC is thrown from a horizontal ground at an angle θ=45∘ with the speed 25m/s. In space, a horizontal electric field E=2×107 V/m exists in the direction of motion. Find the range on horizontal ground of the projectile thrown. Take g=10m/s2.
View SolutionA charged particle of mass m=1 kg and charge q=2μC is thrown for a horizotal ground at an angle θ=45∘ with speed 20m/s. In space a horizontal electric field E=2×107 V/m exist. Find the range on horizontal ground of the projectile thrown.
View SolutionA particle of mass m=0.1kg and having positive charge q=75μC is suspended from a point by a thread of length l=10cm. In the space, a uniform horizontal electric field E=104NC−1 exists. The particle is drawn aside so that the thread becomes vertical and then it is projected horizontally with velocity v such that the particle starts to move along a circle with the same constant speed V. Calculate the radius of the circle and speed v(g=10ms−2).
View SolutionA particle of mass m and charge −q is projected from the origin with a horizontal speed v into an electric field of intensity E directed downward. Choose the wrong statement. Neglect gravity
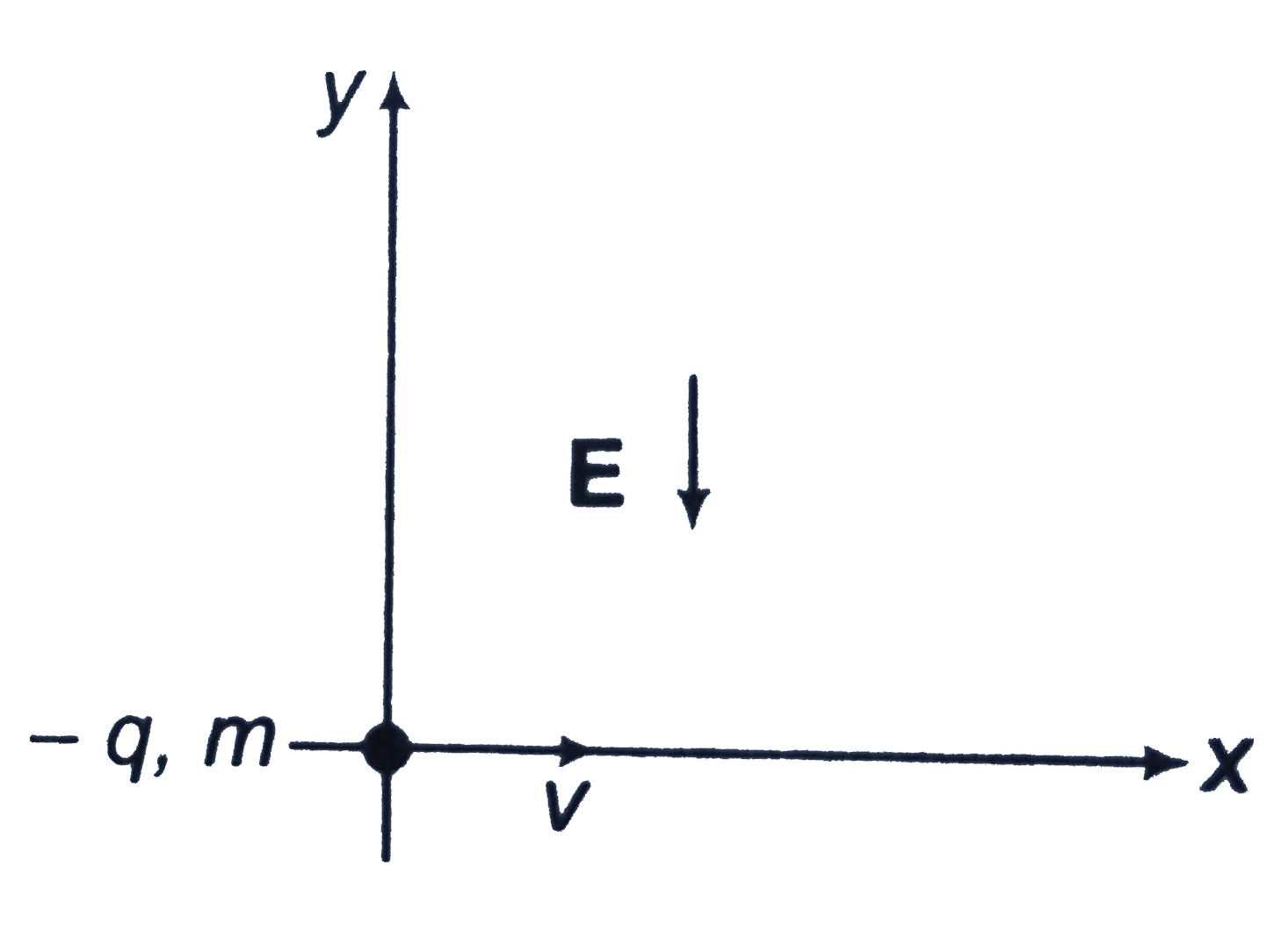
View SolutionA particle of mass m and charge −q is projected from the origin with a horizontal speed v into an electric field of intensity E directed downward. Choose the wrong statement. Neglect gravity

View SolutionA particle of mass 2 kg charge 1 mC is projected vertially with velocity k10ms−1. There is as uniform horizontal electric field of 104N/C, then
View SolutionFrom the ground, a projectile is fired at an angle of 60 degree to the horizontal with a speed of 20 m s−1 The horizontal range of the projectile is
View SolutionA particle is projected from horizontal making an angle of 53∘ with initial velocity 100ms−1. The time taken by the particle to make angle 45∘ from horizontal is
View SolutionA particle of mass 2 kg chrge 1 mC is projected vertially with velocity k10ms−1. There is as uniform horizontal electric field of 104N/C, then
View SolutionA particle of mass 2 kg chrge 1 mC is projected vertially with velocity k10ms−1. There is as uniform horizontal electric field of 104N/C, then
View Solution
