
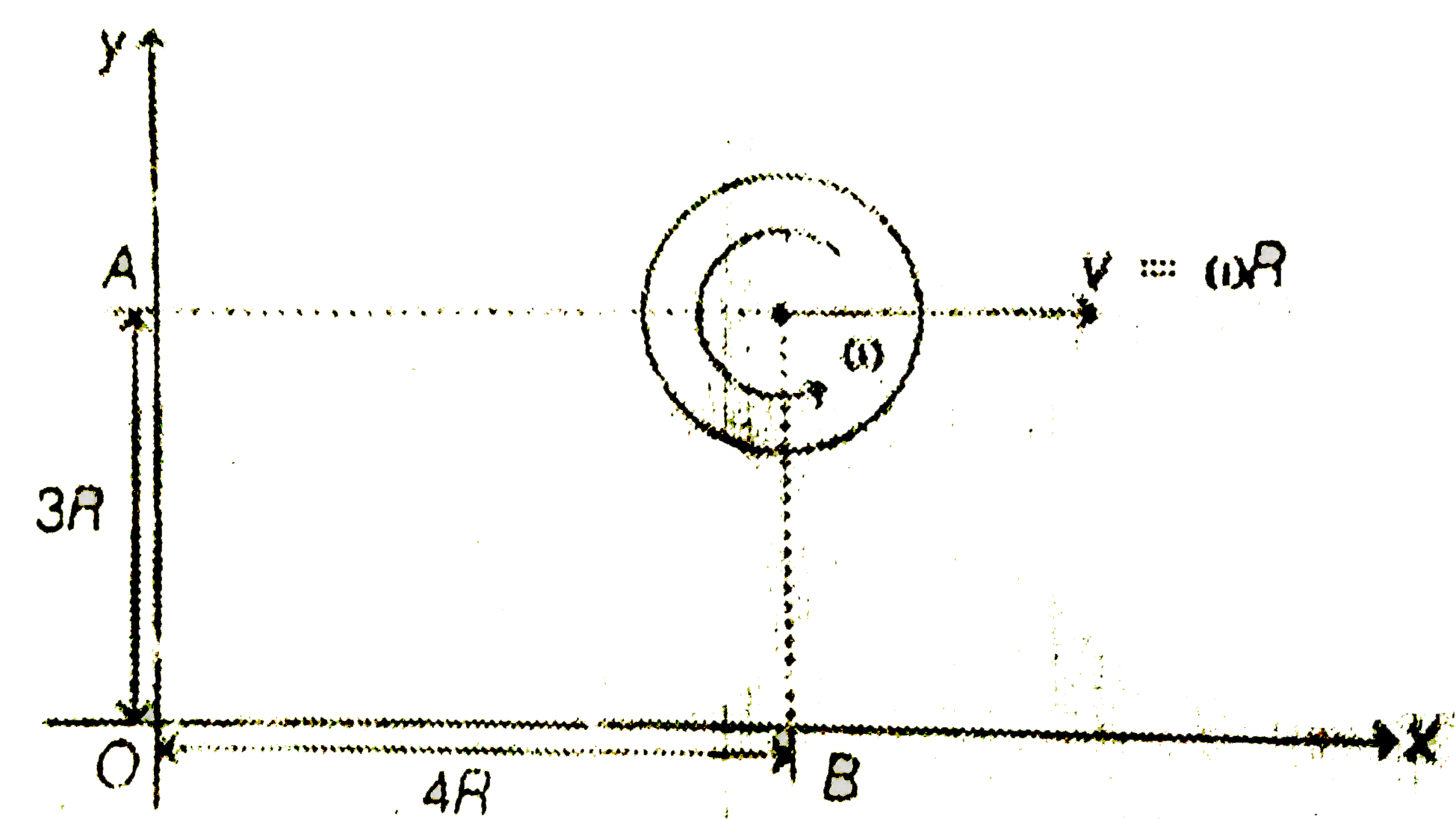
A cicular disc of mass m and radius R is set into motion on a horizontal floor with a linear speed v in the forward direction and an angular speed ω=vR in clockwise direction as shown in figure. Find the magnitude of the total angular momentum of the disc about bottom most point O of the disc.
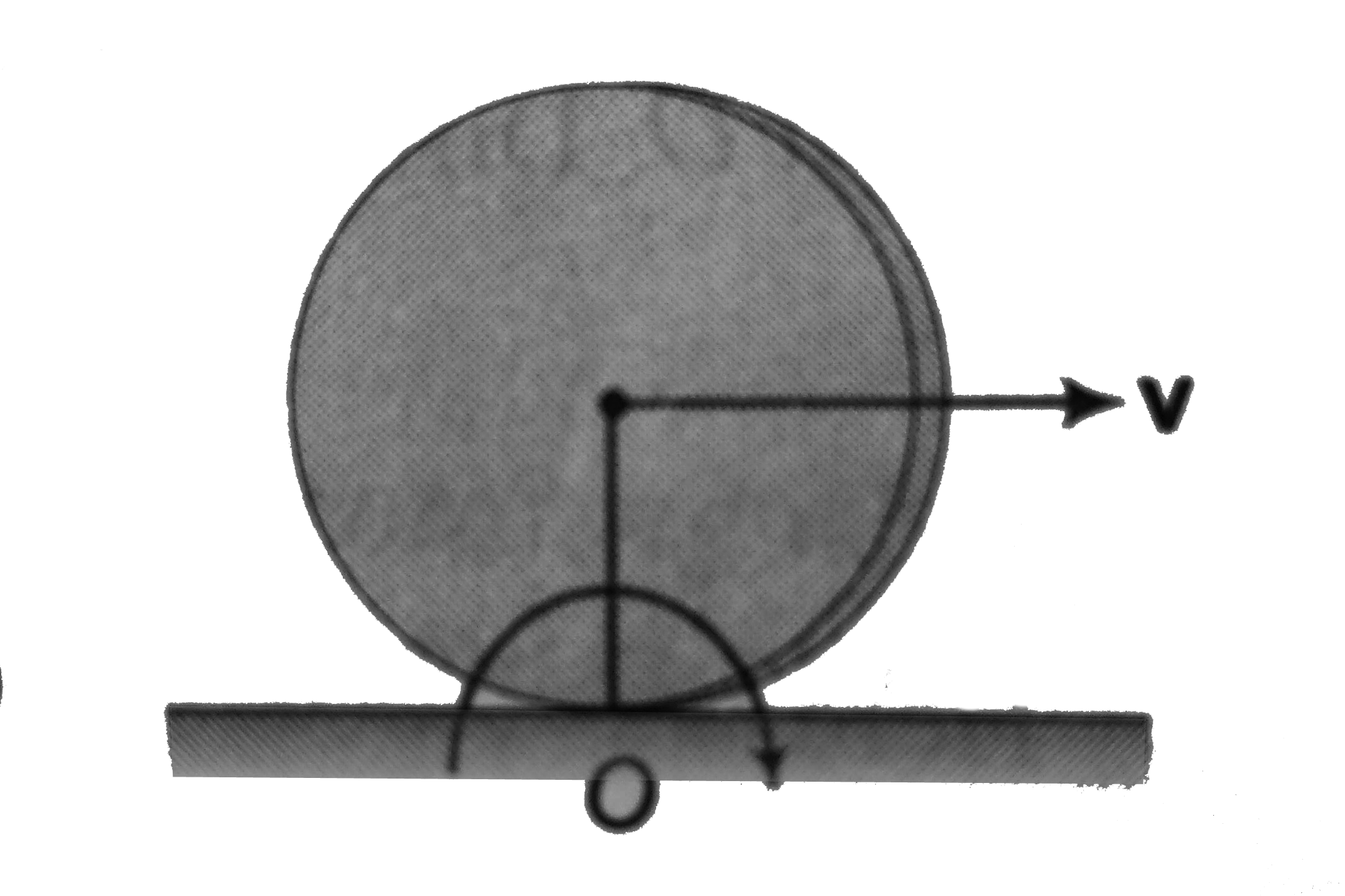
As we have discussed, angular momentum about O is the vector sum of two terms:
Iω and mvr⊥
Here, Iω=Icmω=(12mR2)(vR)=12mvR (perpendicular to paper inwards)
and mvr⊥=mvcr⊥=mRv (perpendicular to paper inwards)
Since, both the terms are in the same direction
Ltotal=12mvR+mvR
or Ltotal=32mvR (perpendicular to paper inwards)
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Solved Examples
25 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Miscellaneous Examples
2 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
A disc of mass M and Radius R is rolling with an angular speed ω on the horizontal plane. The magnitude of angular momentum of the disc about origin is:
View SolutionA solid sphere of radius R is set into motion on a rough horizontal surface with a inear speed v0 in forward direction and an angular with a linear speed v0 in forward directioin and an angular velocity ω0=v0/2R in counter clockwise direction as shown in figure. If coefficient of friction μ then find
a. the time after which sphere starts pure rolling,
b. the linear speed of sphere when it starts rolling and
c. the work down by friction over a long time.
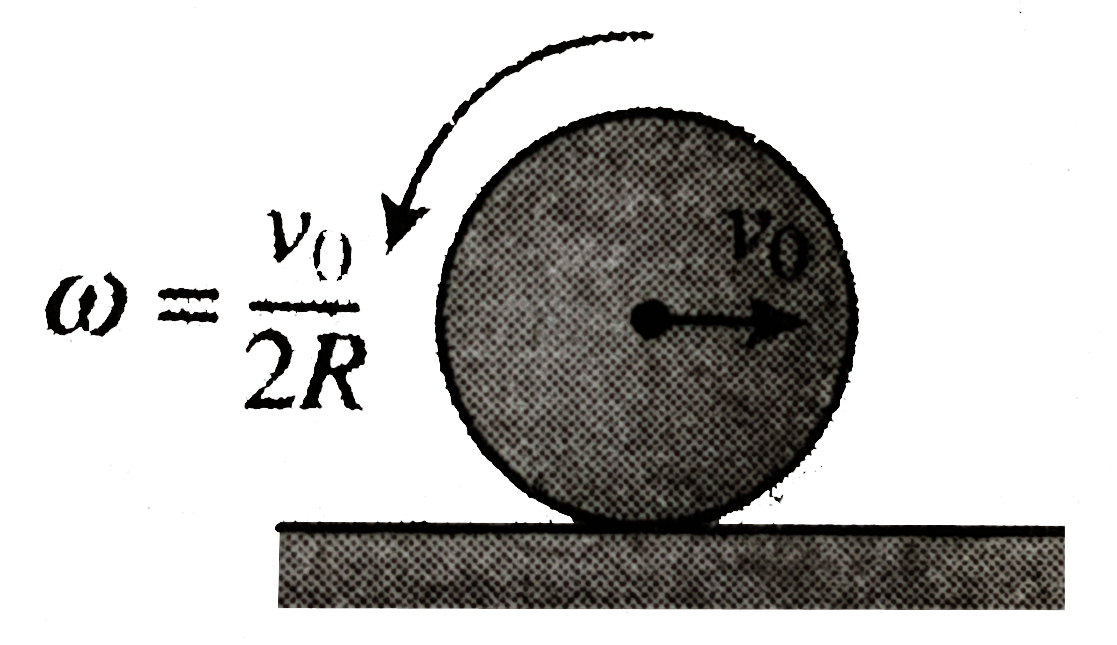
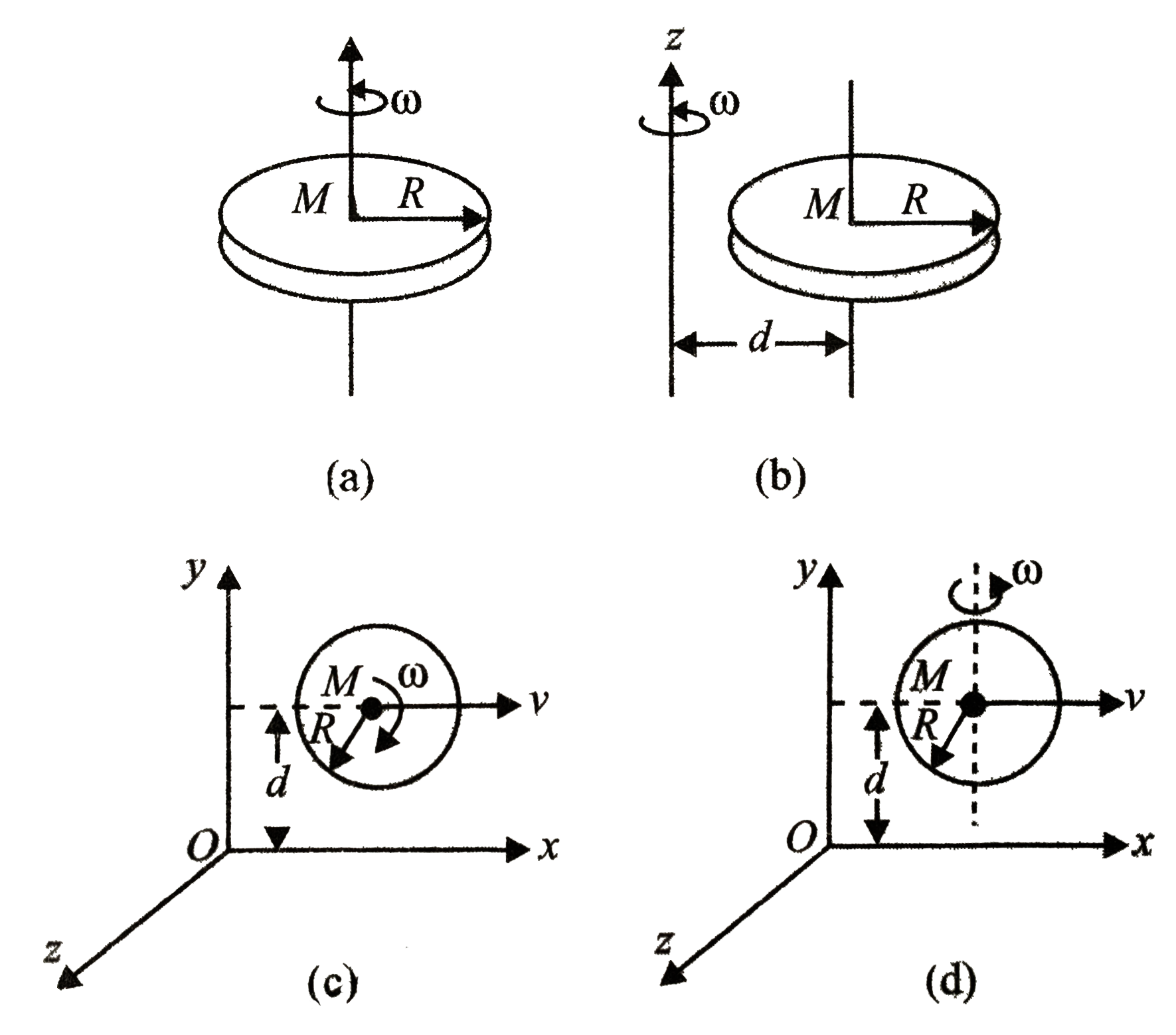
View SolutionFind the angular momentum of a disc about the axis shown in figure in the following situations.
View SolutionA uniform circular disc of mass m is set rolling on a smooth horizontal table with a uniform linear velocity v. Find the total K.E. of the disc.
View SolutionFind the ratio of magnitude of angular momentu of a rolling disc about origin to that about the point P on the disc, as shown in the figure.
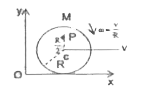
View SolutionA disc of mass m and radius R moves in the x−y plane as shown in Fig. The angular momentum of the disc about the origin O at the instant shown is

View SolutionA disc of mass M and radius R moves in the x-y plane as shown in the figure. The angular momentum of the disc at tihe instant shows is
View SolutionA uniform disc of mass m and radius R is released gentiy on a horizontal rough surface. Such that centre of the disc has velocity V0 towards right and angular velocity ω0 (anticlockwise) as shown.

Disc will certainly come back to its intial position if
View SolutionIn example number 12.16 suppose the disc starts rotating anticlockwise with the same angular velocity ω=vR, then what will be the angular momentum of the disc about bottommost in this new situation?
View Solution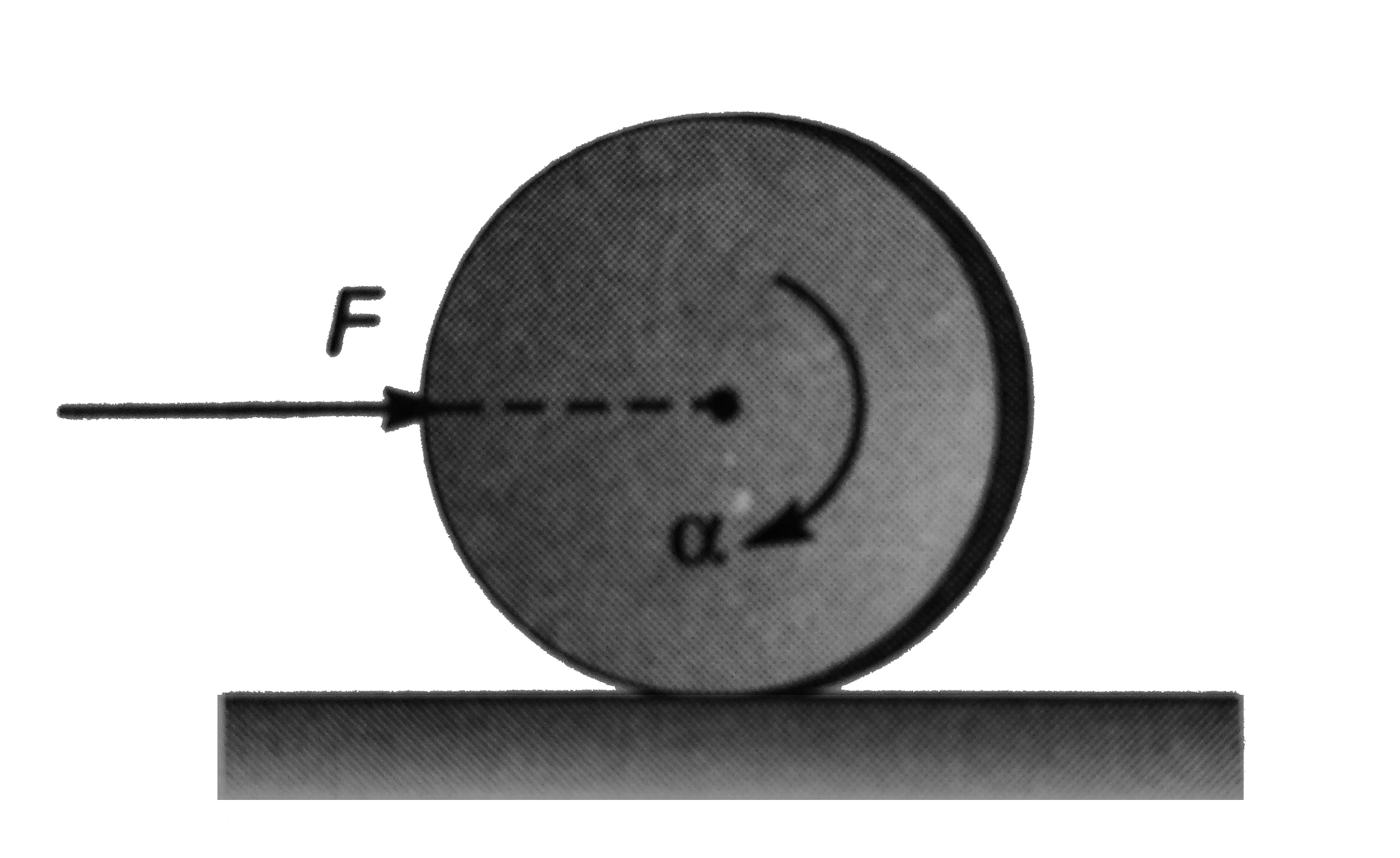
Consider a uniform disc of mass m, radius r rolling without slipping on a rough surface with linear acceleration a and angular acceleration α due to an external force F as shown in the figure coefficient of friction is μ.
Q. Angular momentum of the disc will be conserved about
View Solution
