A circular tube of uniform cross section is filled with two liquids densities ρ1 and ρ2 such that half of each liquid occupies a quarter to volume of the tube. If the line joining the free surfaces of the liquid makes an angle θ with horizontal find the value of θ.
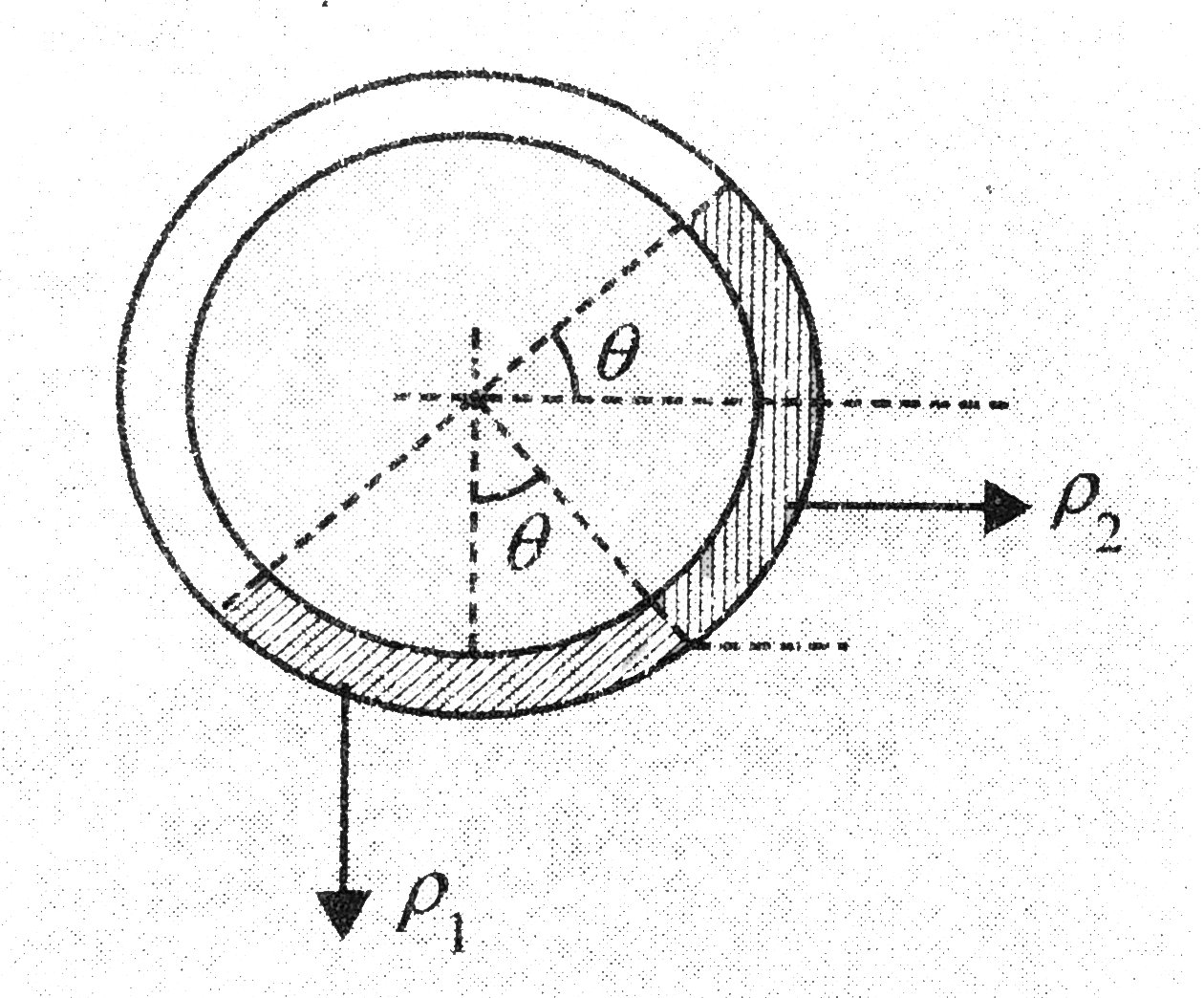
Let us find the pressure at the lowes point 1. Sicne the liquid has density ρ2 and height of liquid column is h′2 on the right and side of point 1 we have
P1=ρ1gh1……i

Since two liquid coluns of heights h1 and h2 and densities ρ1 and ρ2 are situated above point 1, on the left hand side, we have
P2=ρ1gh2+ρ2gh′2.............ii
Equating P1 and P2 from eqn i and ii we have
ρ1h2+ρ2h′2=ρ1h1...........iii
Substituting h′2=Rsinθ+Rcosθ,h2=R(1−cosθ) and h1=R(1−sinθ) in eqn iii we have
ρ1R(1−cosθ)+ρ2R(sinθ+cosθ)=ρ1R(1−sinθ)
This gives
cosθ+sinθcosθ−sinθ=ρ1ρ2⇒tanθ=ρ1−ρ2ρ1+ρ2
Then θ=tan−1(ρ1−ρ2ρ1−ρ3)
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Solved Examples
7 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.1
11 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A rectangular tube of uniform cross section has three liquids of densities ρ1,ρ2 and ρ3. Each liquid column has length l equal to length of sides of the equilateral triangle. Find the length x of the liquid of density ρ1 in the horizontal limb of the tube, if the triangular tube is kept in the vertical plane.

View Solution_E01_110_Q01.png)
The U-tube shown has uniform cross-section A liquid is filled in the two arms up to height h1 and h2 and then the liquid is allowed to move neglect viscosity and surface tension. When the levels equalize in the two arms, the liquid will
View SolutionIf two liquids of same mass but densities ρ1 and ρ2 respectively are mixed, then the density of the mixture is:
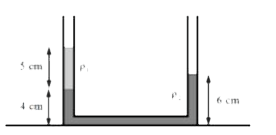
View SolutionA narrow U-tube placed on a horizontal surface contains two liquids of densities ρ1 and ρ2 . The columns of the liquids are indicated in the figure. The ratio ρ1ρ2 is equal to :
View SolutionThe figure represents a U-tube of uniform cross-section filled with two immiscible liquids. One is water with density ρw and the other liquid is of density ρ . The liquid interface lies 2cm above the base. The relation between ρ and ρw is
 .
.
View SolutionTwo non - viscous, incompressible and immiscible liquids of densities (rho) and (1.5 rho) are poured into the two limbs of a circular tube of radius ( R) and small cross section kept fixed in a vertical plane as shown in fig. Each liquid occupies one fourth the cirumference of the tube.
Find the angle (theta) that the radius to the interface makes with the verticles in equilibrium position.
View SolutionA vertical pole of length l , density ρ , area of cross section A floats in two immiscible liquids of densities ρ1and ρ2. In equuilibrium possition the bottom end is at the interface of the liquids. When the cylinder is displaced vertically, find the time period of oscillation.
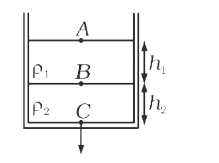
View SolutionA large tank is filled with two immiscible liquids of densities ρ1andρ2 A small hole is made at the base of the tank and liquid of density ρ2 starts flowing out of it. Three points A, B and C are marked on a vertical line. Velocity with which liquid leaves the hole is :
View SolutionThe U-tube shown in the figure has a uniform cross section. A liquid is filled up to heights h1 and h2 in the two arms and they are allowed to move. Neglecting viscosity and surface tension, find the state of the liquid when the levels equalize in the two arms. Find the correct statement.
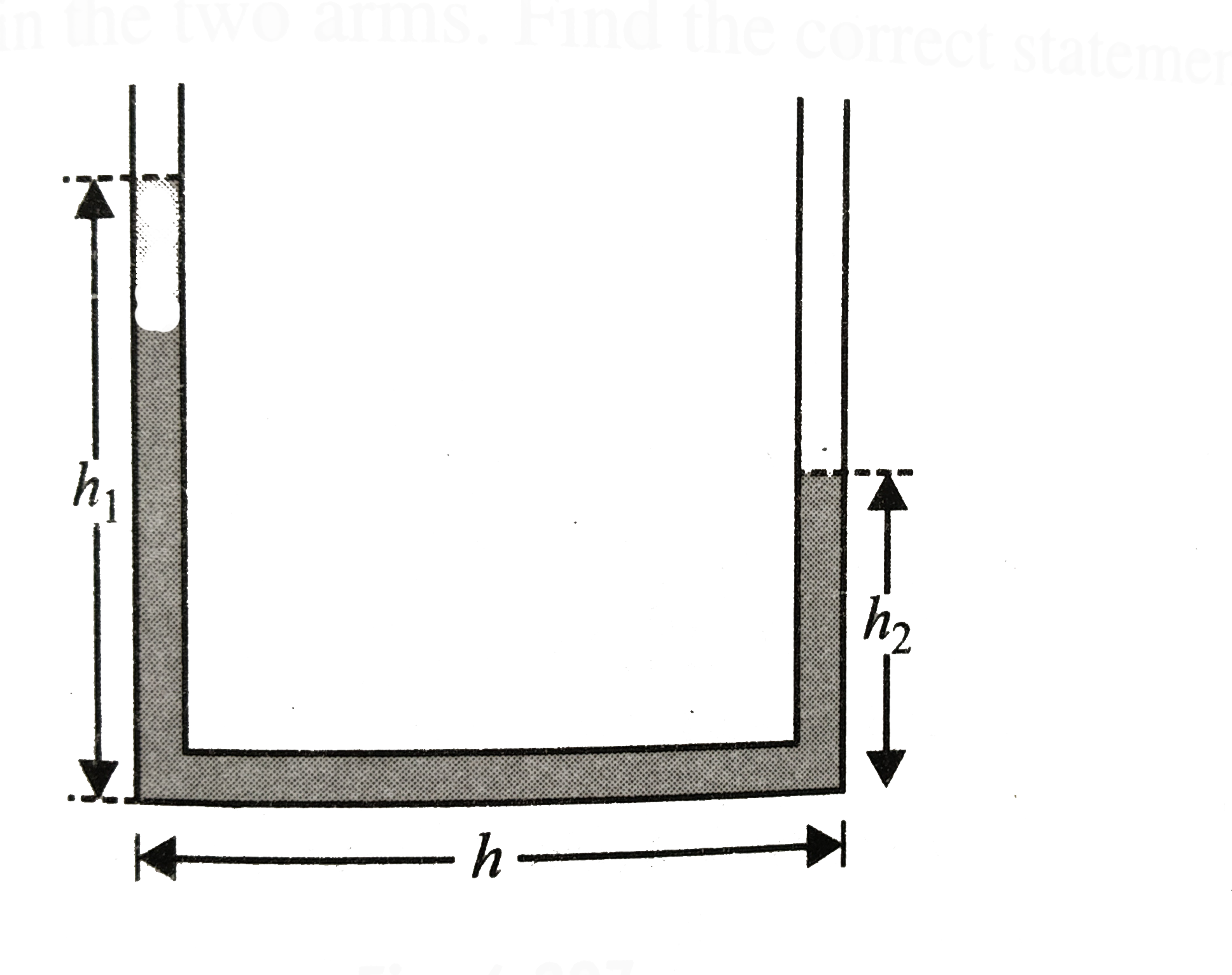
View SolutionA thin uniform circular tube is kept in a vertical plane. Equal volume of two immiscible liquids whose densites are ρ1 and ρ2 fill half of the tube as shown. In equilibrium the radius passing through the interface makes an angle of 30∘ with vertical. The ratio of densities (ρ1/ρ2) is equal to

View Solution
