Question

A fish situated at a depth h below the surface of water in a lake ,can see the outside objects in air through a circular aperature of radius r . What is the radius of the aperture in terms of h and n ,where ${\displaystyle n={}_a{n}_w}$? ${\displaystyle [{}_a{n}_w}$ = R.I. of water w.r.t. air]

Options

A. ` r = h sqrt( n^(2) - 1)`
B. `r = (h)/(sqrt(n^(2) - 1))`
C. `r = (h)/(n^(2) - 1)`
D. `r = (h)/(sqrt(n-1))`

Answer

B

Explanation

The fish can see outside objects through a circular aperture of radius r .

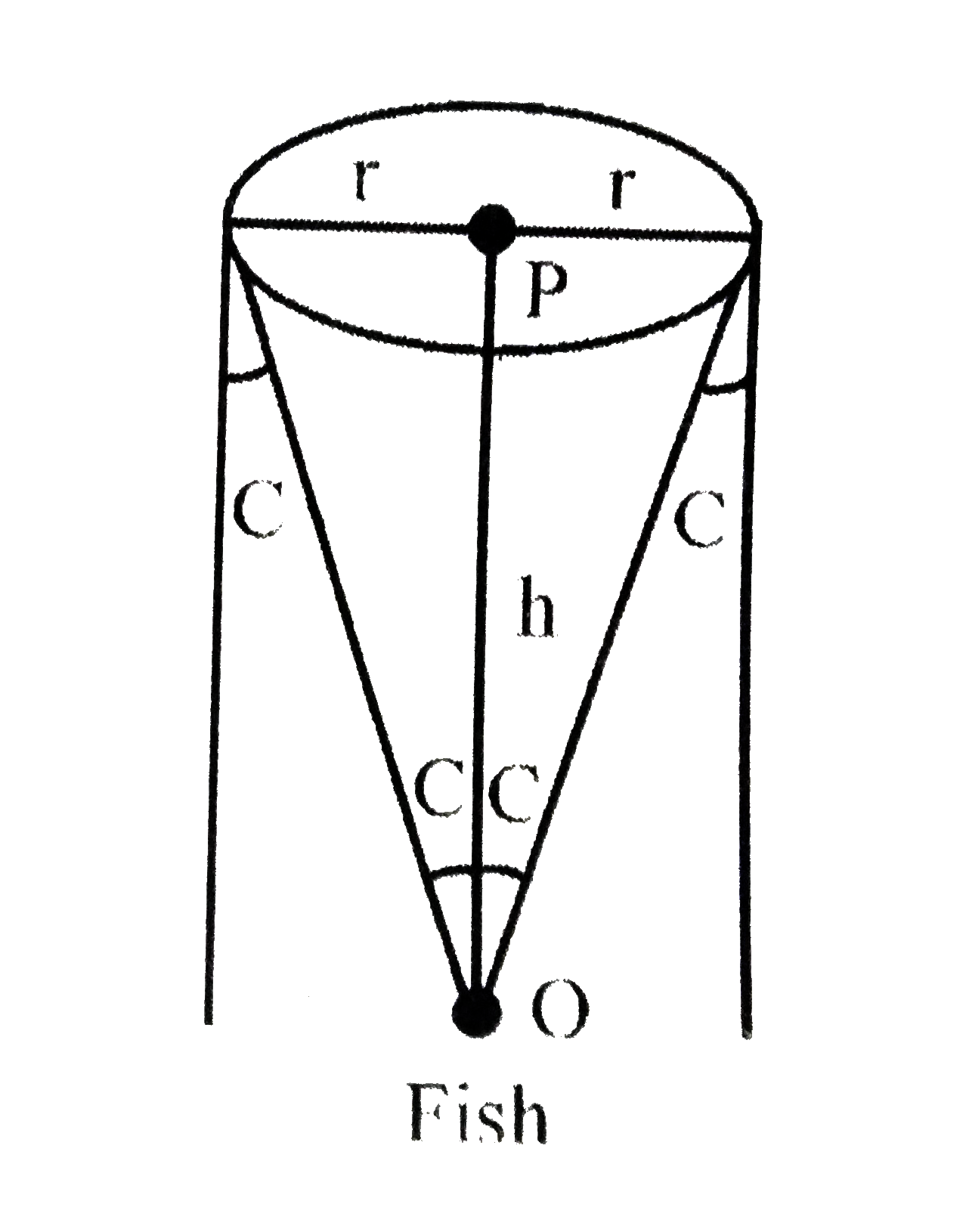
The rays of light are going from a denser medium to a rarer medium . If C is the critical angle , then from the figure , `tan C = (r)/(h)`
`therefore (sin C)/(cos C) = (r)/(h) therefore r = (h sin C)/(cos C) = (h sin C)/(sqrt(1- sin^(2)C))`
But `n = (1)/(sin C) therefore sin C = (1)/(n)`
`therefore r = ( h xx (1)/(n))/(sqrt(1 - ((1)/(n))^(2))) = (h)/(n sqrt((n^(2) - 1)/(n^(2))))`
`therefore r = (h)/(sqrt(n^(2) - 1))`