A helicopter takes off along the vertical with an acceleration a=3m/s2 and zero initial velocity. In a certain time the pilot switches off the engine. At the point of take off, the sound dies away in a time t2=30sec. Determine the velocity of the helicopter at the moment when its engine is switched off assuming that velocity of sound is 320 m/s.
The correct Answer is:[80m/s]
To solve the problem, we need to determine the velocity of the helicopter at the moment when its engine is switched off. We will follow these steps:
Step 1: Determine the distance traveled by the helicopter before the engine is switched off.
The helicopter takes off with an initial velocity u=0 and an acceleration a=3m/s2. The distance s traveled by the helicopter in time t can be calculated using the equation of motion:
s=ut+12at2
Substituting the values:
s=0⋅t+12⋅3⋅t2=32t2
Step 2: Relate the time taken by the sound to travel the same distance.
When the engine is switched off, the sound takes time t′ to travel the distance s back to the ground. The speed of sound is given as vs=320m/s. The distance s can also be expressed in terms of the time t′:
s=vs⋅t′=320⋅t′
Step 3: Establish the relationship between t and t′.
According to the problem, the total time from when the helicopter takes off to when the sound reaches the ground is given as:
t+t′=30seconds
From this, we can express t′ in terms of t:
t′=30−t
Step 4: Substitute t′ into the distance equation.
Now, we can substitute t′ into the equation for distance s:
32t2=320⋅(30−t)
Step 5: Rearranging the equation.
Expanding and rearranging gives:
32t2+320t−9600=0
To eliminate the fraction, we can multiply the entire equation by 2:
3t2+640t−19200=0
Step 6: Solve the quadratic equation.
We can use the quadratic formula t=−b±√b2−4ac2a where a=3, b=640, and c=−19200.
Calculating the discriminant:
b2−4ac=6402−4⋅3⋅(−19200)
=409600+230400=640000
Now, applying the quadratic formula:
t=−640±√6400002⋅3
=−640±8006
Calculating the two possible values for t:
1. t=1606=803seconds (valid)
2. t=−14406=−240seconds (not valid)
Step 7: Calculate the velocity of the helicopter.
Now that we have t=803, we can find the velocity v of the helicopter at the moment the engine is switched off using the formula:
v=u+at
Substituting the known values:
v=0+3⋅803=80m/s
Final Answer:
The velocity of the helicopter at the moment when its engine is switched off is 80m/s.
---
=409600+230400=640000
=−640±8006
2. t=−14406=−240seconds (not valid)
Topper's Solved these Questions
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Advance MCQs with One or More Options Correct17videos
KINEMATICS
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Unsolved Numerical94videos
GRAVITATION
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - exercise 1.33videos
LINEAR MOMENTUM & ITS CONSERVATION
Similar Questions
Knowledge Check
- Question 1 - Select One or More
A particle is moving rectilineraly so that its acceleration is given as a=3t2+1m/s2. Its initial velocity is zero.
AThe velocity of the particle at t=1 sec will be 2m/sBThe displacement of the particle in 1 sec will be 2m.CThe particle will continoue to move in positive direction.DThe particle come back to its starting point after some time. - Question 2 - Select One
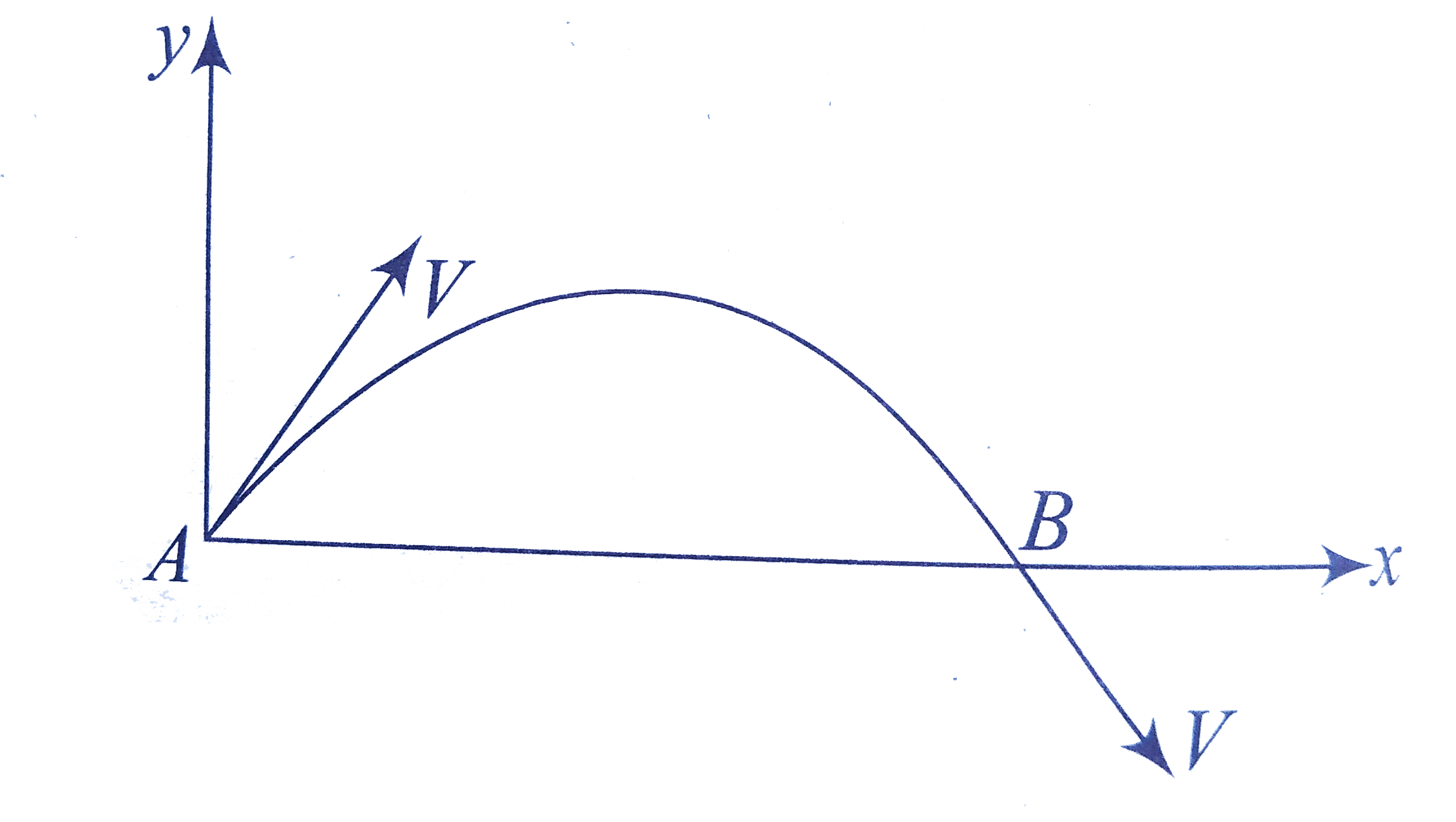
The velocity of a projectile at the initial point A is (2ˆi+3ˆj)m/s. Its velocity (in m/s) at point B is
 A−2ˆi−3ˆjB−2ˆi+3ˆjC2ˆi−3ˆjD2ˆi+3ˆj
A−2ˆi−3ˆjB−2ˆi+3ˆjC2ˆi−3ˆjD2ˆi+3ˆj - Question 3 - Select One
The acceleration of paticle varies with time as :
a(t)=3t2+4
If the initial velocity of particle is 2m/s, find the velocity of particle at t=3sec.A41m/sB4m/sC39m/sD27m/s
A jet plane starts from rest with an acceleration of 3ms−2 and makes a run for 35 s before taking off. What is the minimum length of the runway and what is the velocity of the jet at take off ?
View SolutionThe velocity of a projectile at the initial point A is (2ˆi+3ˆj)m/s.Its velocity (in m//s) at point B is
 View Solution
View SolutionVelocity of a body moving with uniform acceleration of 3m/s2 is changed through 30 m/s in certain time. Average velocity of body during this time is 30 m/s. Distance covered by it during this time is
View SolutionA body has an initial velocity of 3m/s and has an acceleration of 1m/sec2 normal to the direction of the initial velocity. Then its velocity 4 seconds after the start is
View SolutionA rocket is fired upward from the earth's surface such that it creates an acceleration of 20m/s2. If after 5 s its engine is switched off, the maximum height of the rochet from the earth's surface would be
View Solution

