A horizontal tube of length I closed at both ends contains an ideal gas of molecular weight M, The tube is roatated at a constant angular velocity ω about a vertical axis passing through an end. Assuming the temperature to be uniforme and constant, show that
p2=p1eMω2l22RT,
where p_(2) and p_(1)denote the pressures at the free end and the fixed end respetively.
Consider an element of the gas between the cross sections at distances x and x+dxfrom the fixed end . If p be the pressure at x and p+dpatx+dx,the force acting in the element towards the centre is Adp, where A is the cross sectional area. As this element is going in a circle of radius X,
Adp=(dm)(ω2)x...(i)
where dm=mass of the element. Using pV=nRT on this element,
pAdx=dmMRT
or,dm=MpARTdx.
Putting in (i),
Adp=MpART(ω2)xdx
or,∫p2p1dpp=∫l0m(ω2)RTxdx
Inp2p1=M(ω2)l22RT
(p2=p1)eM2ωl22RT.

Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - KINETIC THEORY OF GASESEXERCISE - Short Answer14videos
KINETIC THEORY OF GASES
- BOOK - HC VERMACHAPTER - KINETIC THEORY OF GASESEXERCISE - Objective 117videos
KINETIC THEORY OF GASES
- BOOK - HC VERMACHAPTER - KINETIC THEORY OF GASESEXERCISE - Exercises62videos
KINETIC THEORY OF GASES
- BOOK - HC VERMACHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Exercises19videos
INTRODUCTION TO PHYSICS
- BOOK - HC VERMACHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Short Answer15videos
LAWS OF THERMODYNAMICS
Similar Questions
A horizontally oriented tube AB of length l rotates with a constant angular velocity ω about a stationary vertical axis OO′ passing through the end A (figure). The tube is filled with an ideal fluid. The end A of the tube is open, the closed end B has a very small orifice. Find the velocity of the fluid relative to the tube as a function of the column "height" h.
 View Solution
View SolutionA horizontal cylinder closed from one end is rotated with a constant angular velocity ω about a vertical axis passing through the open end of the cylinder. The outside air pressure is equal to p0, the temperature to T, and the molar mass of air to M. find the air pressure as a function of the distance r from the rotate axis. The molar mass is assumed to be independent of r.
View Solution
Knowledge Check
- Question 1 - Select One
A uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
A12mω2xB12mω2(1−x2l)C12mω2l(1−x2l2)D12mω2l[1−xl] - Question 2 - Select One
For an L shaped conducting rod placed in an uniform magnetic field →B rotating with constant angular velocity ω about an axis passing through one of its ends A and normal to the plane of the conductor induced emf
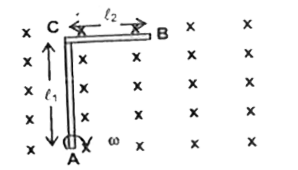 Aacross AC equals ωBl21Bacross AC equals 12ωBl21Cacross BC equals zeroDNone of the above is correct
Aacross AC equals ωBl21Bacross AC equals 12ωBl21Cacross BC equals zeroDNone of the above is correct - Question 3 - Select One
A thin uniform copper rod of length l and cross-section area A and mass m rotates uniformly with an angular velocity ω in a horizontal plane about a vertical axis passing through one of its ends. The elongation of the rod will be
Amω2l26AYBmω2l23AYCmω2l2AYDmω2l22AY
A conducting rod of length l is rotating with constant angular velocity ω about point O in a uniform magnetic field B as shown in the figure . What is the emf induced between ends P and Q ?
 View Solution
View SolutionA horizontal tube with closed ends is rotated with a constant angular velocity ω about a vertical axis passing through one of its ends. The tube contains carbon dioxide at a temperature T=300K. The length of the tube is l=100cm. Find the value ω at which the ratio of molecular concentrations at the opposite ends of the tube is equal to η=2.0.
View SolutionA horizontally oriented bent thin tube ABC of length 1.4m rotates with a constant angular velocity ω about a stationary vertical axis OO' passing through the end A as shown in figure. The portion BC of tube is parallel to OO' and its length is equal 0.4m. At t=0, AB part of tube is filled with an ideal fluid. The end A of tube is open, the closed end C has a very small orifice. Find the maximum height reached by fluid relative to end C. (take g=10/s2 and ω=10rad/s)
 View Solution
View SolutionA metallic rod of length lis hinged at one end and the other end is rotated with a constant angular speed ω about an axis passing through the hinge. A constant and uniform magnetic field B, parallel to the axis of rotation exists everywhere. The induced emf between the two ends of rod is 12Blω2.
View SolutionA copper rod of length l is rotated about one end perpendicular to the uniform magnetic field B with constant angular velocity ω . The induced e.m.f. between its two ends is
View Solution

