A mass M is suspended as shown in fig. The system is in equilibrium. Assume pulleys to be massless. K is the force constant of the spring.
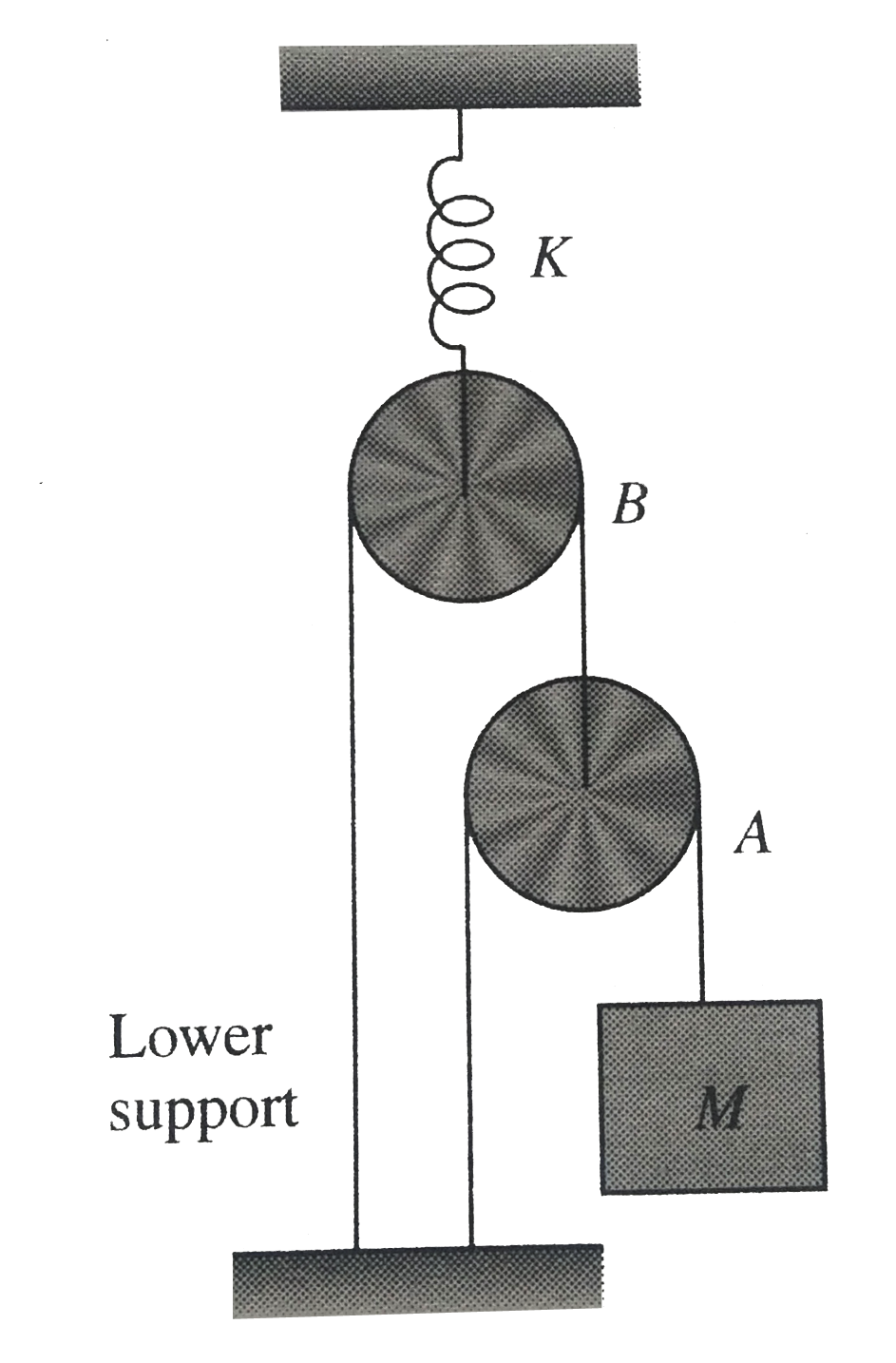
Find the net tension force acting on the lower support.
Mg
2Mg
3Mg
4Mg
The correct Answer is:C
Total tension on lower support:
T1+T=2Mg+Mg=3Mg.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer5videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Assertion-reasoning15videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE10videos
MISCELLANEOUS VOLUME 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type1videos
NEWTON'S LAWS OF MOTION 2
Similar Questions
Whole system shown is at rest. Pulley and string are massless. Net force on the pulley by the string is?
View SolutionOn applying a force F the mass M is displaced vertically down by from equilibrium position, Find the force F in terms of the force constant k of the spring and displacement y for the cases (A) and (B) as shown in
View Solution
Knowledge Check
- Question 1 - Select One
A mass M is suspended as shown in fig. The system is in equilibrium. Assume pulleys to be massless. K is the force constant of the spring.
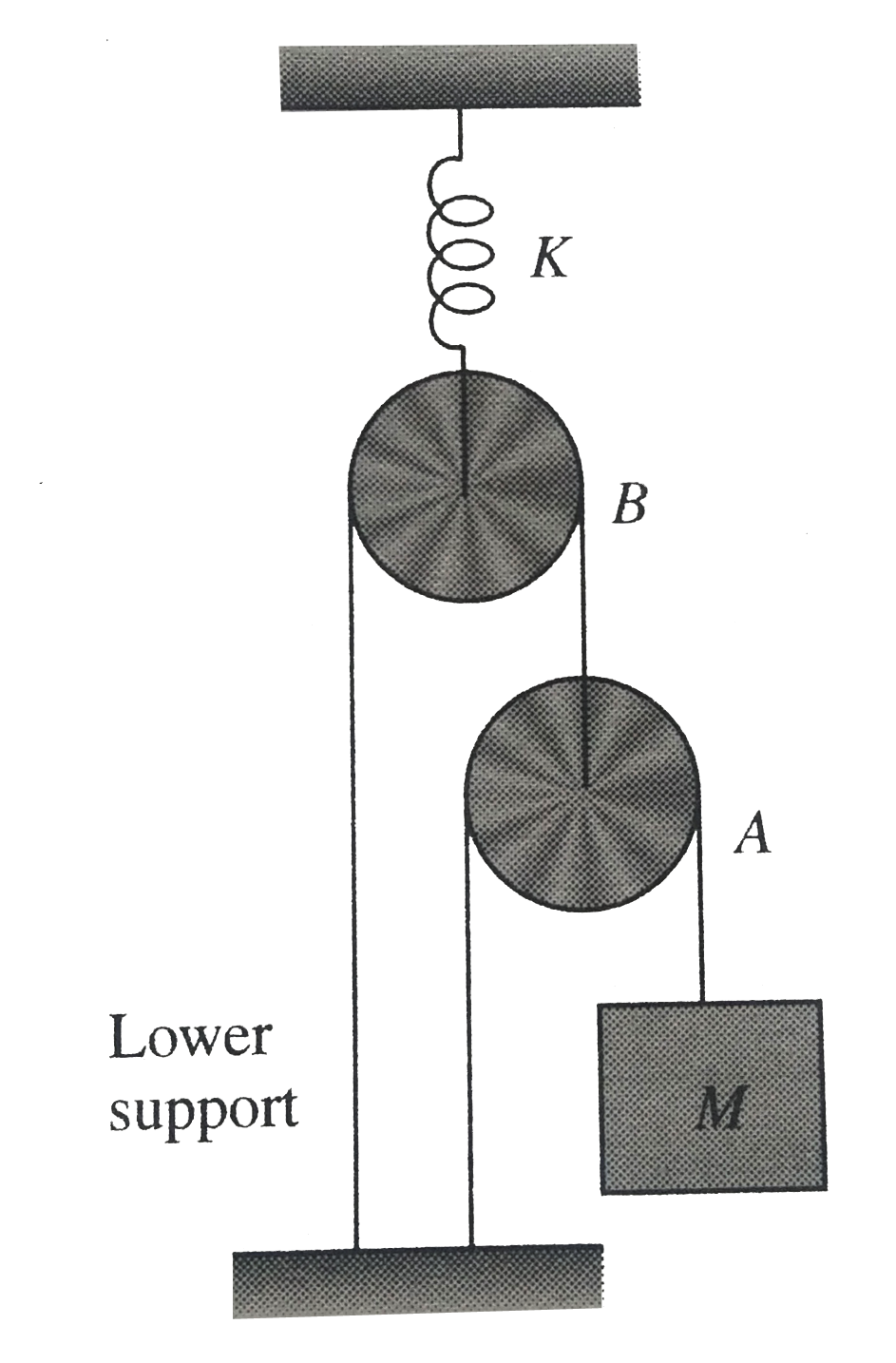
The extension produced in the spring is given byA4Mg/KBMg/KC2Mg/KD3Mg/K - Question 2 - Select One
A mass M is suspended as shown in fig. The system is in equilibrium. Assume pulleys to be massless. K is the force constant of the spring.
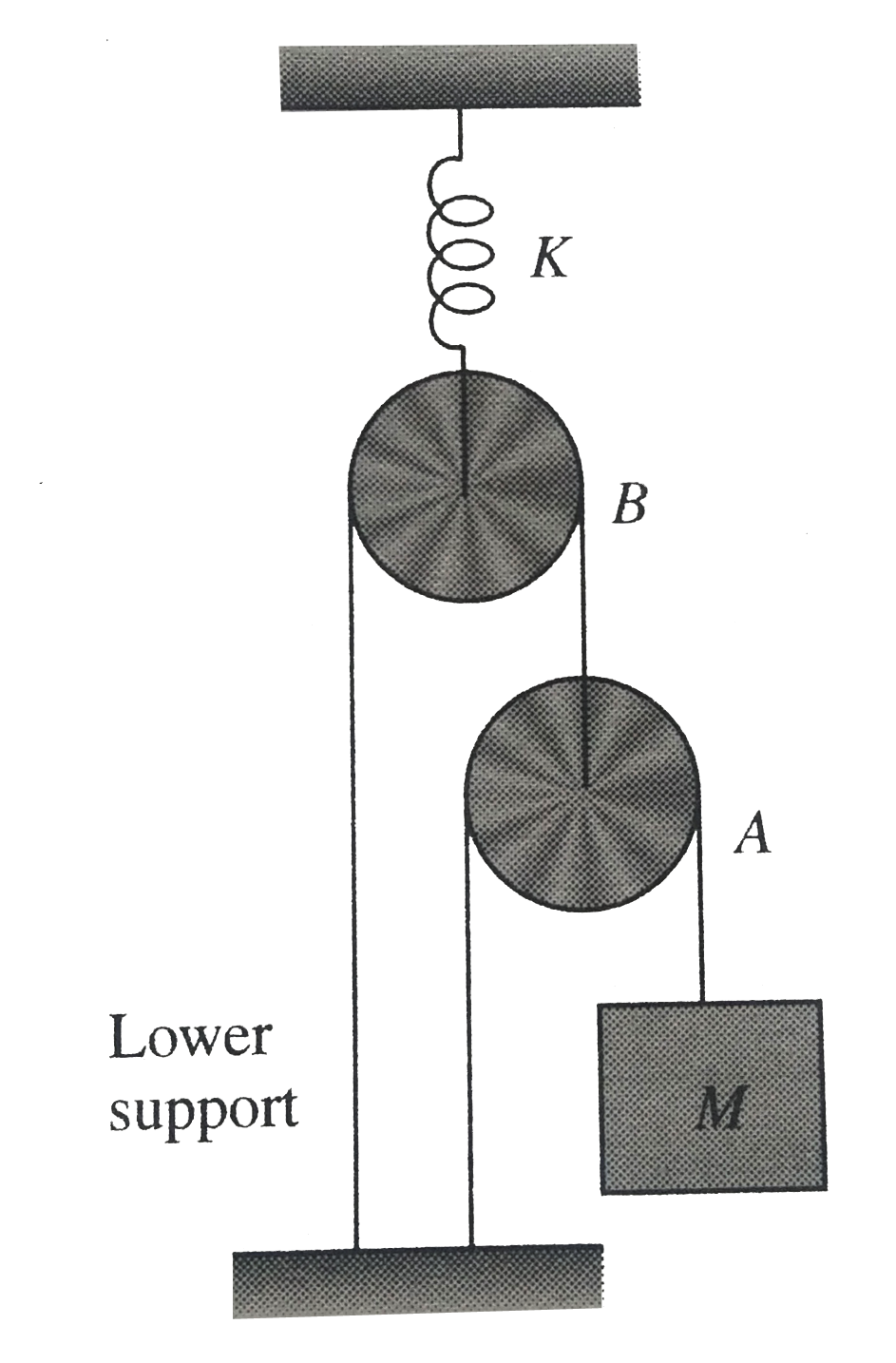
If each of the pullies A and B has mass M, then find the net tension force acting on the lower support. Asumme pulleys to be frictionaless.A2MgB6MgC3MgD4Mg - Question 3 - Select One
What will be the force constant of the spring system shown in figure?
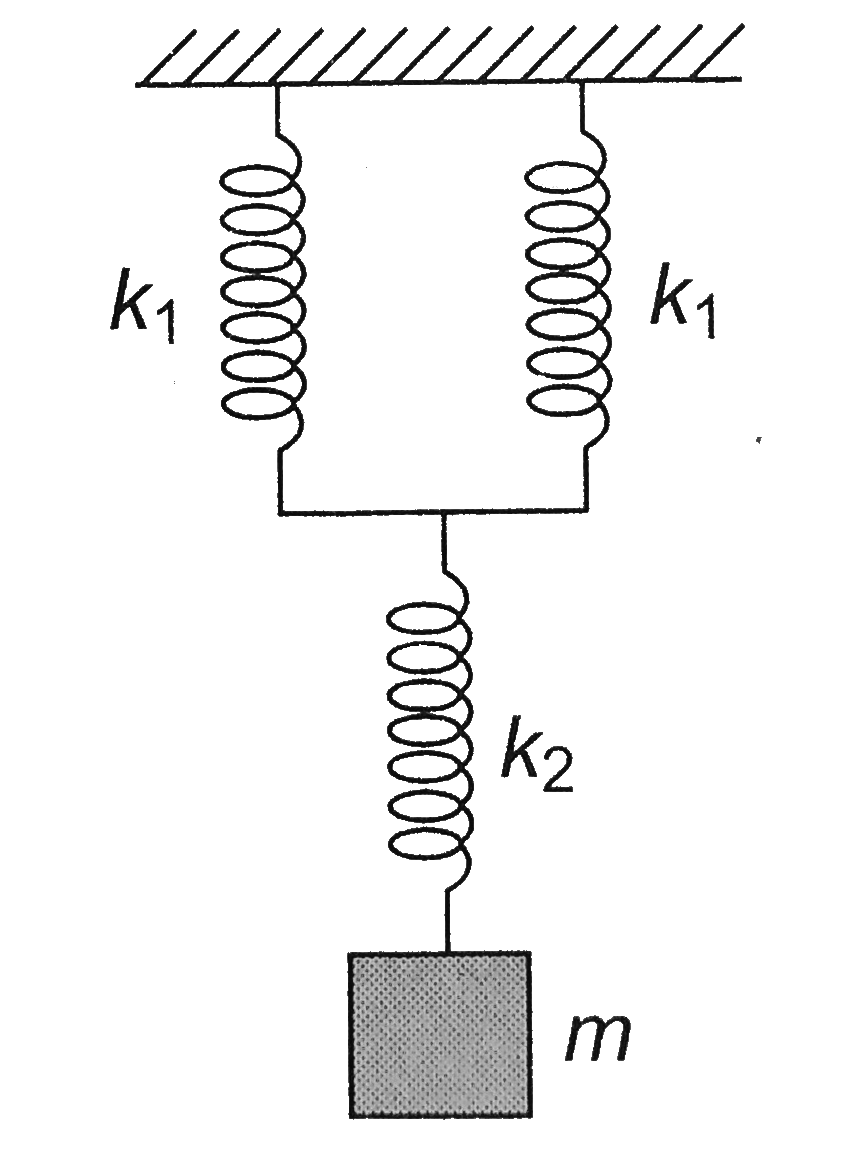 A[1k1+1k2]B[12k1+1k2]−1C[1k1+1k2]−1D[12k1+1k2]
A[1k1+1k2]B[12k1+1k2]−1C[1k1+1k2]−1D[12k1+1k2]
Two masses of 5 kg and 10 kg are suspended from a fixed support as shown in the figure. The system is pulled downwards with a force of 150 N applied to the lower mass. The string attached to the support breaks and the system accelerates downwards. If the downward force continues to act, the acceleration of the center of mass of the system is :
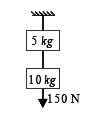 View Solution
View SolutionFind the reading of spring balance as shown in fig. Assume that mass M in equilibrium. (All surfaces are smooth).
 View Solution
View SolutionIn the shown mass pulley system, pulleys and string are massless. The one end of the string is pulled by the force F = mg. The acceleration of the block will be :
 View Solution
View Solution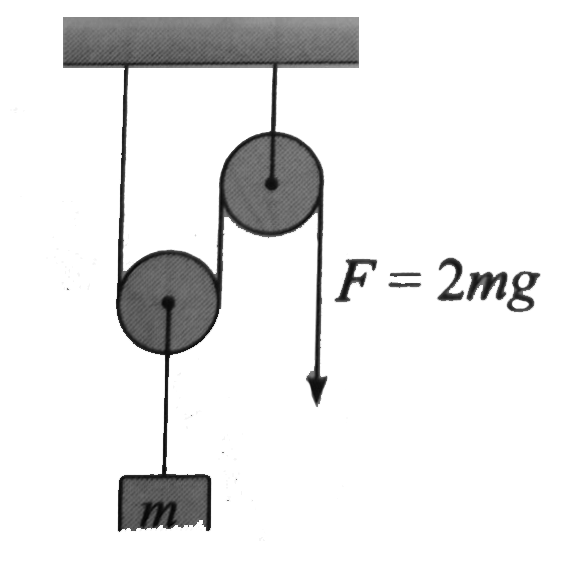
In the shown mass pulley system, pulleys and string are massless. The one end of the string is pulleed by the force F=2mg. The acceleration of the block will beView SolutionThe period of the free oscillations of the system shown here if mass m1 is pulled down a little and force constant of the spring is k and masses of the fixed pulleys are negligible, is
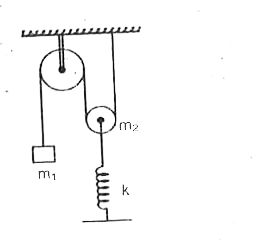 View Solution
View Solution

