A particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom

Match the following
Table-1Table-2(A)Velocity at B(P)7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5 mg(T)None
The correct Answer is:A, B, C, D
v2B=u2A−2ghAB=(9gR)−(2gR)=7gR
∴ vB=√7gR
Further, TB=mv2BR=7mg
Again, v2C=v2A−2ghAC=(9gR)−2(2R)=5gR
∴ vC=√5gR
Further, TC+mg=mv2CR
∴TC=4mg
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery19videos
CIRCULAR MOTION
- BOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Taking it together67videos
CIRCULAR MOTION
- BOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective21videos
CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION
- BOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct27videos
COMMUNICATION SYSTEM
Similar Questions
A particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom. Match the following:

Column-1 Column-2(A)Velocity at B(P) 7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5mg(T)NoneView SolutionMatch the following.
∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)Coefficient of viscosity(P)[M2L−1T−2](B)Surface tension(Q)[ML0T−2](C)Modulus of elasticity(R)[ML−1T−2](D)Energy per unit volume(S)Noneof a fiuid∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣View Solution
Knowledge Check
- Question 1 - Select One
A particle is suspended from a string of length R. It is given a velocity u=3√gR at the lowest point.

column−I column−II(A)Velocity at B (P)7mg(B) Velocity at C (Q)√5qR(C)Tension in string at B(R) (R)√7gR(D)Tension in string atC(S) (S)4mgAA-R, B-Q, C-P, D-SBA-Q, B-R, C-S, D-PCA-R, B-P, C-Q, D-SDA-P, B-S, C-R, D-Q
A particle of mass m is suspended from a string so that it can move in a circular path in vertical plane. It is given v=3√glat the bottom most point. Match the values of tension in string at A,B,C,D
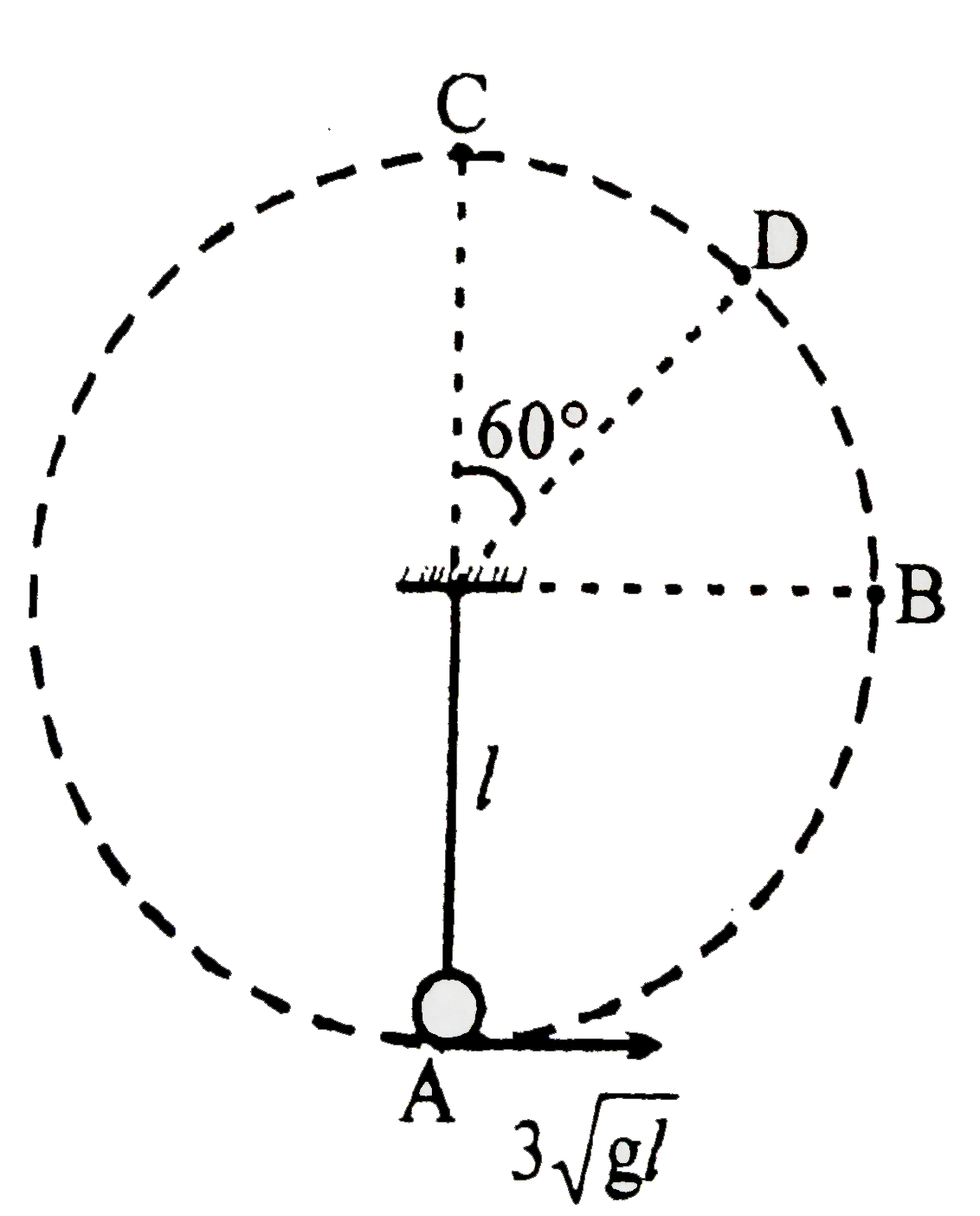
Column IColumn II(A) TA(P) 10 mg ),((B) T_(B),,(Q)7mg),((C) T_(C),,(R)4mg),((D) T_(D),,(S)5mg),(,,(T)5.5mgView SolutionA heavy particle hangs from a point O, by a string of length a. It is projected horizontally with a velocity u=√(2+√3)ag . The angle with the downward vertical, string makes where string becomes slack is :
View SolutionA particle of mass m is suspended by a string of length l from a fixed rigid support. Particle is imparted a horizontal velocity u=√2gl. Find the angle made by the string with the vertical when the acceleration of the particle is inclined to the string by 45∘?
View SolutionIn th efigure shown in fig. 10.33 , a bob attached with a light string of radius R is given as initial velocity u=√4gR at the bottommost point.
(a) At what height string will slack.
(b) What is velocity of the bob just befare slacking of string.
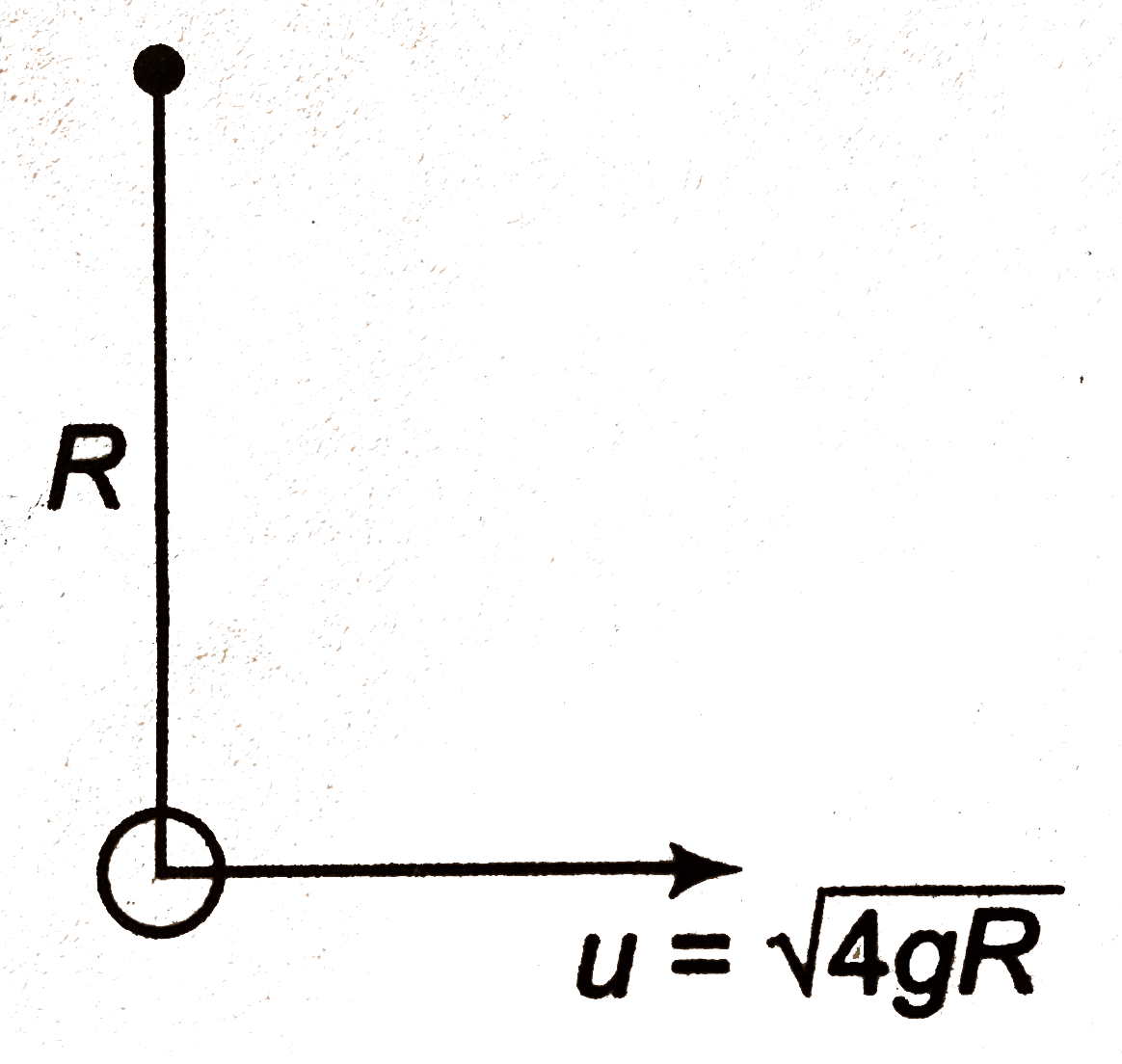 View Solution
View SolutionA particle is projected from earth surface with a velocity √4gR3 in upward direction where R is the radius of earth. Find the velocity of particle when it is at half its maximum height.
View SolutionMatch the following:
Column-IColumn-II(A) Root mean square velocity(p)√3Pd(B) Average velocity(q)√3RTm(C) Most probable velocity(r)√8Pπd(D) Velocity possessed by maximum fraction of molecules(s)√2RTmView SolutionA particle of mass 1kg has velocity →v1=(2t)ˆi and another particle of mass 2kg has velocity →v2=(t2)ˆj
Column IColumn II(A)Netforce on centre of mass at 2 s(p)209unit(B)Velocity of centre of mass at 2 s(q)√68 unit(C)Displacement of centre of mass in 2s(r)√803unit(s)NoneView Solution

