A particle of mass m moves on the x- axis as follows : it starts from rest at t=0, from the point x=0, and comes to rest at t=1 at the point x=1. No other information is available about its motion at intermediate times (0<t<1) . If α denotes the instantaneous accelartion of the particle , then :
A. α cannot remain positive for all t in the interval
B. |α| cannot exceed 2 at any point in its path.
C. |α| must be >=4 at some point or points in its path.
D. α must change sign during the motion, but no other assertion can be made with the information given.
The correct Answer is:A, D
To solve the problem, we need to analyze the motion of a particle that starts from rest, moves along the x-axis, and comes to rest again after a certain time. Here's a step-by-step breakdown of the solution:
Step 1: Understand the Initial and Final Conditions
- The particle starts from rest at t=0 and x=0. This means:
- Initial velocity u=0
- Initial position x0=0
- The particle comes to rest at t=1 and x=1. This means:
- Final velocity v=0
- Final position xf=1
Step 2: Analyze the Motion
Since the particle starts and ends at rest, it must accelerate at some point and then decelerate to come to rest. This implies that the acceleration cannot be positive throughout the entire motion.
Step 3: Evaluate the Acceleration
- Let α(t) denote the instantaneous acceleration of the particle.
- Since the particle starts from rest and comes to rest again, the acceleration must change sign during the motion:
- Initially, the particle must accelerate (positive acceleration) to gain speed.
- After reaching a certain speed, it must decelerate (negative acceleration) to come to rest.
Step 4: Check the Options
1. Option A: "Alpha cannot remain positive for all t in the interval (0,1)."
- This statement is true because the particle must decelerate to come to rest at t=1. Therefore, option A is correct.
2. Option B: "Alpha cannot exceed 2 at any point of its path."
- To evaluate this, we can use the kinematic equation x=ut+12αt2. For the entire motion from t=0 to t=1:
1=0⋅1+12α(1)2⟹α=2 m/s2
- However, since the particle can accelerate more than this value before decelerating, option B is incorrect.
3. Option C: "Mod alpha must be greater than 4 at some point of its path."
- We can analyze the motion further, but since we found that the maximum acceleration can be 2 m/s², this statement is not necessarily true. Therefore, option C is incorrect.
4. Option D: "Alpha must change sign during the motion."
- This statement is true because the particle must accelerate and then decelerate, indicating that the acceleration must change from positive to negative. Therefore, option D is correct.
Conclusion
The correct options are A and D.
- The particle starts from rest at t=0 and x=0. This means:
- Initial velocity u=0
- Initial position x0=0
- The particle comes to rest at t=1 and x=1. This means:
- Final velocity v=0
- Final position xf=1
Since the particle starts and ends at rest, it must accelerate at some point and then decelerate to come to rest. This implies that the acceleration cannot be positive throughout the entire motion.
- Let α(t) denote the instantaneous acceleration of the particle.
- Since the particle starts from rest and comes to rest again, the acceleration must change sign during the motion:
- Initially, the particle must accelerate (positive acceleration) to gain speed.
- After reaching a certain speed, it must decelerate (negative acceleration) to come to rest.
1. Option A: "Alpha cannot remain positive for all t in the interval (0,1)."
- This statement is true because the particle must decelerate to come to rest at t=1. Therefore, option A is correct.
- To evaluate this, we can use the kinematic equation x=ut+12αt2. For the entire motion from t=0 to t=1:
1=0⋅1+12α(1)2⟹α=2 m/s2
- However, since the particle can accelerate more than this value before decelerating, option B is incorrect.
- We can analyze the motion further, but since we found that the maximum acceleration can be 2 m/s², this statement is not necessarily true. Therefore, option C is incorrect.
- This statement is true because the particle must accelerate and then decelerate, indicating that the acceleration must change from positive to negative. Therefore, option D is correct.
The correct options are A and D.
Topper's Solved these Questions
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Linked Comprehension Type
35 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Integer Type
17 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single correct anwer type
14 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
A particle of mass 0.1 kg is subjected to a force F which varies with distance x as shown. If it starts its journey from rest at x = 0, then its speed at X = 12 m is
View SolutionA particle starts from rest at time t=0 and moves on a straight line with acceleration as plotted in fig. The speed of the particle will be maximum after time.

View SolutionA particle moves along the x - axis according to x=A[1+sinωt]. What distance does is travel in time interval from t=0 to t=2.5π/ω ?
View SolutionA particle starts from the origin at time t = 0 and moves along the positive x-axis. The graph of velocity with respect to time is shown in figure. What is the position of the particle at time t = 5s ?

View SolutionA particle is moving along the x-axis whose acceleration is given by a=3x−4, where x is the location of the particle. At t = 0, the particle is at rest at x=4/3m. The distance travelled by the particles in 5 s is
View SolutionA particle is kept at rest at the origin. A constant force →F starts acting on it at t=0. Find the speed of the particle at time t.
View Solution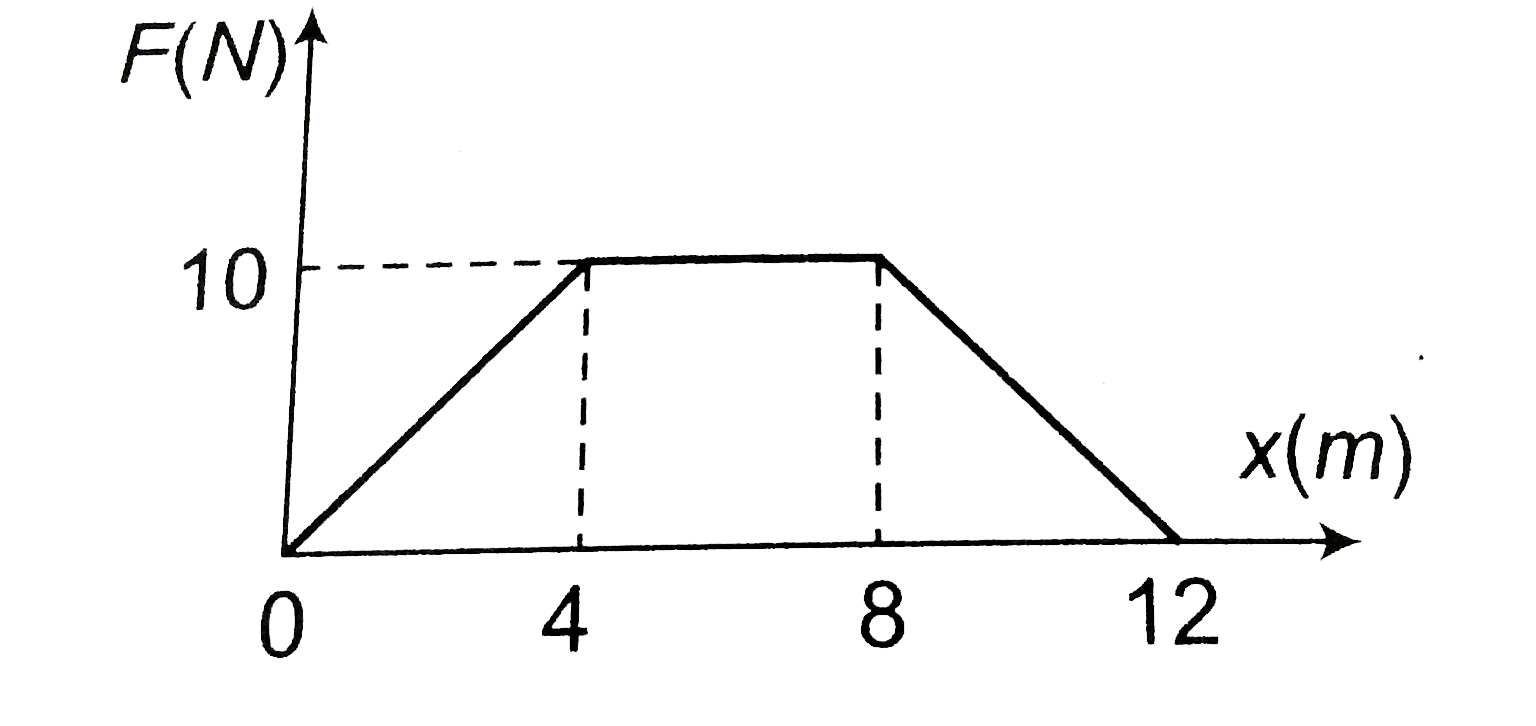
A particle of mass 0.1 kg is subjected to a force which varies with distance as shown in figure. If it starts its journey from rest at x=0, its velocity at x=12m is
View Solution
A particle of mass 0.1 kg is subjected to a force which varies with distance as shown in figure. If it starts its journey from rest at x=0, its velocity at x=12m is
View SolutionA graph of acceleration versus time of a particle starting from rest at t=0 is as shown in figure. The speed of the particle at t=14s is
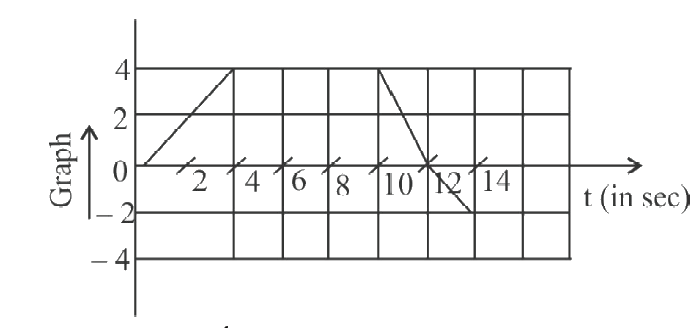
View SolutionStarting from rest, the acceleration of a particle is a=2(t-1). The velocity of the particle at t=5s is :-
View Solution
