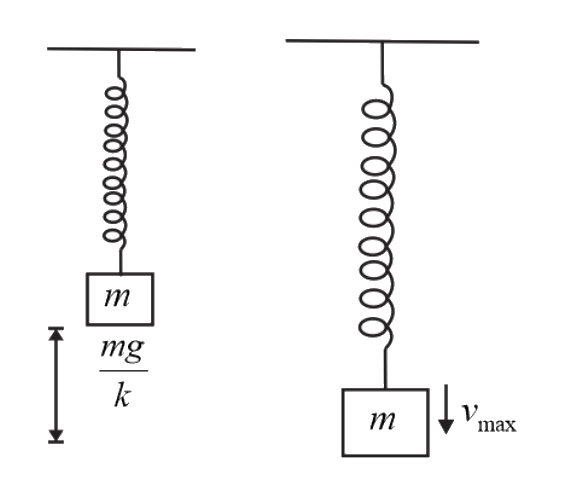
A particle suspended from a vetical spring oscillastes 10 times per sencon. At the highest point of oscillation the spring becomes unstretched. A. Find the maximum speed of the block. B. Find the speed when the spring is stretche by 0.20 cm. Take g=π2ms−2
(a) When a spring in equilibrium, its extension =mgk
At highest point of block, spring is in its natural length
Hence, amplitude A=mgk
f=10Hz,ω=2πf=20πrad/s
ω=√km⇒km=ω2
vmax=Aω=mkgω=gωω2=gω=π220π
=π20m/s
A=mgk=gω2=π2400π2=0.25100m=0.25cm
(b) When extension is 0.20cm, displacement from equilibrium position =0.25−0.20=0.05cm
x=0.05cm
v=ω√A2−x2=20π√(0.25)2−(0.05)2
=2π√6cm/s
=15.4cm/s
Topper's Solved these Questions
Similar Questions
A particle suspended from a vertical spring oscillates 10 times per second. At the highest point of oscillation , the spring becomes upstretched . Find the speed when the spring is stretched by 0.20 cm . (Take , g=π2m/s2)
 View Solution
View SolutionFrequency of a particle executing SHM is 10Hz. The particle is suspended form a vertical spring. At the highest point of its oscillation the spring is unstretched. Find the maximum speed of the particle: (g=10m/s2)
View Solution
Knowledge Check
- Question 1 - Select One
A particle suspended from a vertical spring oscillates 8 times per second. At the highest point of oscillation the spring becomes unstretched. The maximum speed of the block will be
A10 cm/sB20 cm/sC30 cm/sD40 cm/s - Question 2 - Select One
Frequency of a particle executing SHM is 10 Hz. The particle is suspended from a vertical spring. At the highest point of its oscillation the spring is unstretched. Maximum speed of the particle is (g=10m/s2
A2πm/sBπm/sC1πm/sD12πm/s - Question 3 - Select One
Frequency of a particle executing SHM is 10 Hz. The particle is suspended from a vertical spring. At the highest point of its oscillation the spring is unstretched. Maximum speed of the particle is (g=10ms)
A2πmsBπmsC1πmsD12π/ms
The block shown in figure is released from rest. Find out the speed of the block when the spring is compressed by 1 m
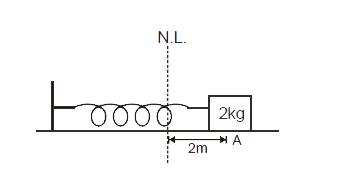 View Solution
View SolutionIn the given arrangement the block is released from the position where the spring is unstretched. The speed of the block when it has descended through 2cm is x10. Find the value of x.
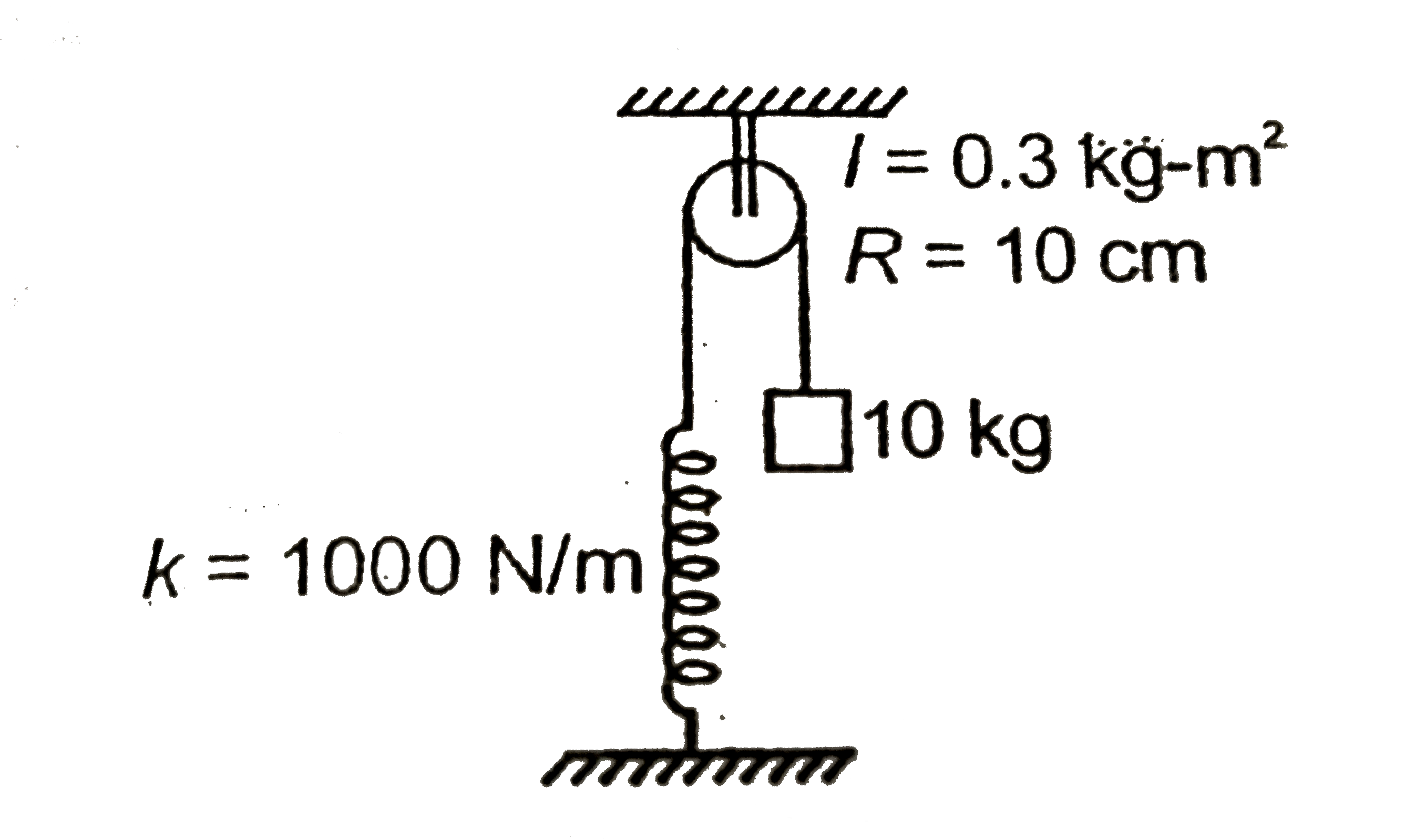 View Solution
View SolutionA mass m suspended from a vertical spring oscillates with a period of 4 s. The mass is then kept at rest. Through what dis"tan"ce the spring will be stretched ? [Take pi^(2)=10]
View SolutionA mass is suspended from a vertica spring which is executing SHM of frequency 5 Hz.
The spring is unstretched at the highest point of oscillation. Maximum speed of the mass is (take, acceleration due to gravity, g=10m/s2 )View SolutionA ball of mass 2kg hanging from a spring oscillates with a time period 2π seconds. Ball is removed when it is in equilibrium position, then spring shortens by
View Solution

