A particle with charge 2.0 C movess through a uniform magnetic field. At one instant the velocity of the particle is (2.0ˆi+4.0ˆj+6.0ˆk) m/s and the magnetic force on the particle is (4.0ˆi−20ˆj+12ˆk) N.
The x and y components of the magnetic fields are equal. What is →B ?
The correct Answer is:→B=(−3.0ˆi−3.0ˆj−4.0ˆk)T
To find the magnetic field vector →B given the information in the problem, we will follow these steps:
Step 1: Define the magnetic field vector
Since the x and y components of the magnetic field are equal, we can express the magnetic field vector as:
→B=x^i+x^j+z^k
Step 2: Write the force equation
The magnetic force →F on a charged particle moving in a magnetic field is given by the equation:
→F=q(→v×→B)
where q is the charge of the particle, →v is its velocity, and →B is the magnetic field.
Step 3: Substitute known values
Given:
- Charge q=2.0C
- Velocity →v=(2.0^i+4.0^j+6.0^k)m/s
- Force →F=(4.0^i−20.0^j+12.0^k)N
We can substitute these values into the force equation:
(4.0^i−20.0^j+12.0^k)=2.0(→v×→B)
Step 4: Calculate the cross product →v×→B
Using the determinant method for the cross product:
→v×→B=∣∣
∣
∣∣^i^j^k246xxz∣∣
∣
∣∣
Calculating the determinant, we have:
→v×→B=^i(4z−6x)−^j(2z−6x)+^k(2x−4x)
=(4z−6x)^i−(2z−6x)^j−2x^k
Step 5: Substitute back into the force equation
Now substituting →v×→B back into the force equation:
(4.0^i−20.0^j+12.0^k)=2.0((4z−6x)^i−(2z−6x)^j−2x^k)
This simplifies to:
(4.0^i−20.0^j+12.0^k)=(8z−12x)^i−(4z−12x)^j−4x^k
Step 6: Equate coefficients
Now we equate the coefficients of ^i, ^j, and ^k:
1. For ^i:
8z−12x=4(1)
2. For ^j:
−4z+12x=−20(2)
3. For ^k:
−4x=12(3)
Step 7: Solve the equations
From equation (3):
x=−3
Substituting x=−3 into equation (1):
8z−12(−3)=4⟹8z+36=4⟹8z=4−36⟹8z=−32⟹z=−4
Step 8: Write the magnetic field vector
Now substituting x and z back into the magnetic field vector:
→B=−3^i−3^j−4^k
Final Answer
Thus, the magnetic field vector is:
→B=−3.0^i−3.0^j−4.0^kT
Since the x and y components of the magnetic field are equal, we can express the magnetic field vector as:
→B=x^i+x^j+z^k
The magnetic force →F on a charged particle moving in a magnetic field is given by the equation:
→F=q(→v×→B)
where q is the charge of the particle, →v is its velocity, and →B is the magnetic field.
Given:
- Charge q=2.0C
- Velocity →v=(2.0^i+4.0^j+6.0^k)m/s
- Force →F=(4.0^i−20.0^j+12.0^k)N
(4.0^i−20.0^j+12.0^k)=2.0(→v×→B)
Using the determinant method for the cross product:
→v×→B=∣∣
∣
∣∣^i^j^k246xxz∣∣
∣
∣∣
→v×→B=^i(4z−6x)−^j(2z−6x)+^k(2x−4x)
=(4z−6x)^i−(2z−6x)^j−2x^k
Now substituting →v×→B back into the force equation:
(4.0^i−20.0^j+12.0^k)=2.0((4z−6x)^i−(2z−6x)^j−2x^k)
This simplifies to:
(4.0^i−20.0^j+12.0^k)=(8z−12x)^i−(4z−12x)^j−4x^k
Now we equate the coefficients of ^i, ^j, and ^k:
1. For ^i:
8z−12x=4(1)
2. For ^j:
−4z+12x=−20(2)
3. For ^k:
−4x=12(3)
From equation (3):
x=−3
8z−12(−3)=4⟹8z+36=4⟹8z=4−36⟹8z=−32⟹z=−4
Now substituting x and z back into the magnetic field vector:
→B=−3^i−3^j−4^k
Thus, the magnetic field vector is:
→B=−3.0^i−3.0^j−4.0^kT
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Multiple
2 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Comphension Based
2 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Comprehension type
8 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False
3 Videos
Similar Questions
A charged particle moves in a uniform magnetic field B=(2ˆi−3ˆj) T
View SolutionAn electron moves through a uniform magnetic fiedl given by B=Bxˆi+(3Bx)ˆj. At a particular instant, the electron has the velocity v=(2.0ˆi+4.0ˆj) m/s and the magnetic force acting on its is (6.4×10−19N)ˆk Find Bx.
View SolutionThe angular velocity of a particle is →w=4ˆi+ˆj−2ˆk about the origin. If the position vector of the particle is 2ˆi+3ˆj−3ˆk, then its linear velocity is
View SolutionAn electron moves through a uniform magnetic fields given by →B=Bxˆi+(3.0Bx)ˆj. At aparticular instant, the electron has velocity →v=(2.0ˆi++4.0ˆj)m/s and the magnetic force acting on it is (6.4×10−19N)ˆk. The value of Bx (in T) will
View SolutionThe velocity of a particle is v=6ˆi+2ˆj−2ˆk The component of the velocity parallel to vector a=ˆi+ˆj+ˆk in vector from is
View SolutionA particle of spectfic charge α enters a uniform magnetic field B=−B0ˆk with velocity V=v0ˆi from the origin Find the time dependence of velocity and position of the particle
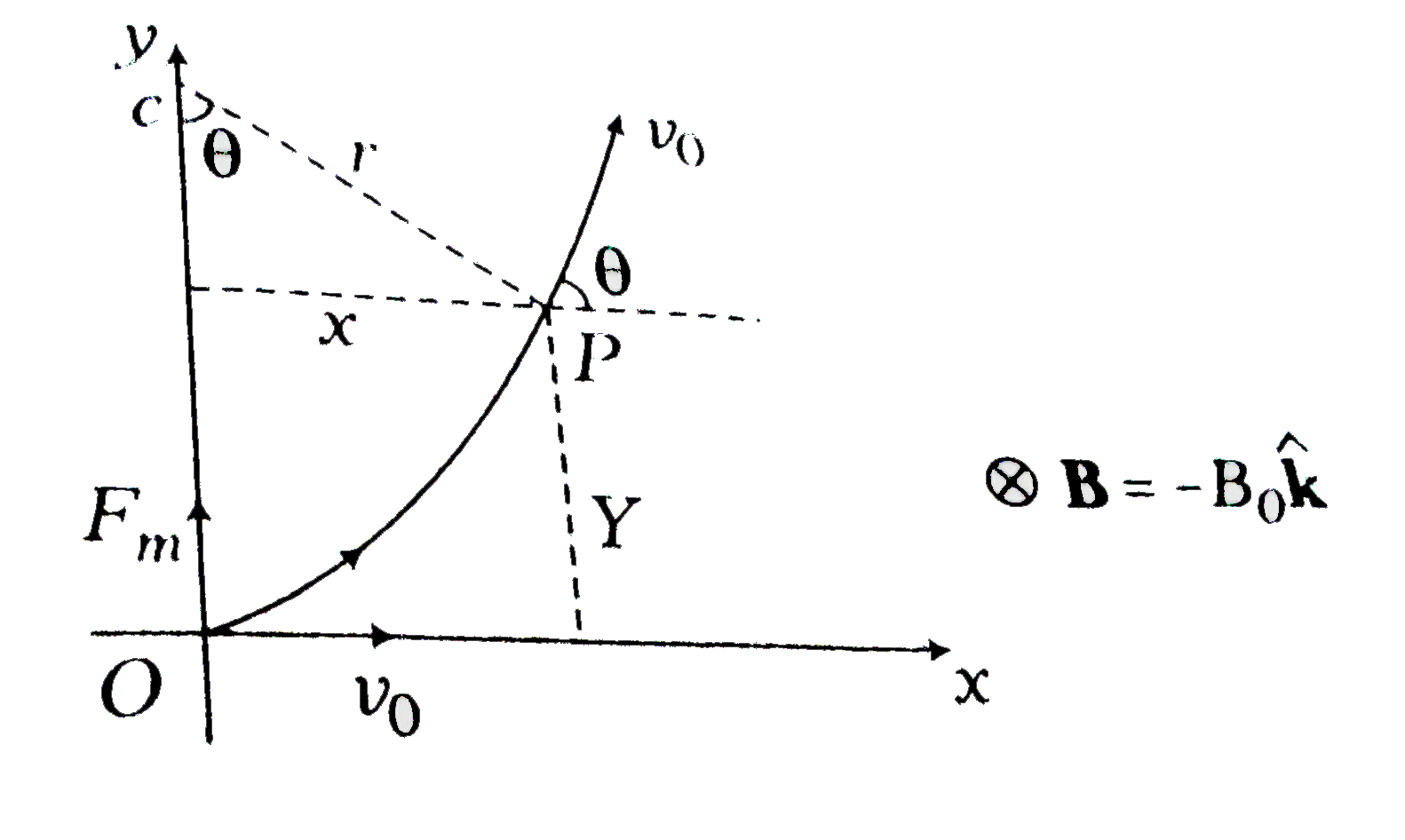
View SolutionA particle with charge 7.80μC is moving with velocity v=−(3.80×103ms)ˆj. The magnetic force on the particle is measured to be F=+(7.60×10−3N)ˆi−(5.20×10−3N)ˆk
(a) Calculate the components of the magnetic field you can find from this information.
(b) Are the components of the magnetic field that are not determined by the measurement of the force? Explain.
(c) Calculate the scalar product B.F. What is the angle between B and F
View SolutionA particle is moving in such a way that at one instant velocity vector of the particle is 3ˆi+4ˆjm/s and acceleration vector is −25ˆi−25ˆjm/s? The radius of curvature of the trajectory of the particle at that instant is
View SolutionA particle of specific charge alpha enters a uniform magnetic field B=−B0ˆk with velocity v=v0ˆi from the origin. Find the time dependence of velocity and position of the particle.

View SolutionAssertion In a uniform magnetic field B=B0ˆk, if velocity of a charged particle is v0ˆi at t = 0, then it can have the velocity v0ˆj at some other instant.
Reason In uniform magnetic field, acceleration of a charged particle is always zero.
View Solution
