Question

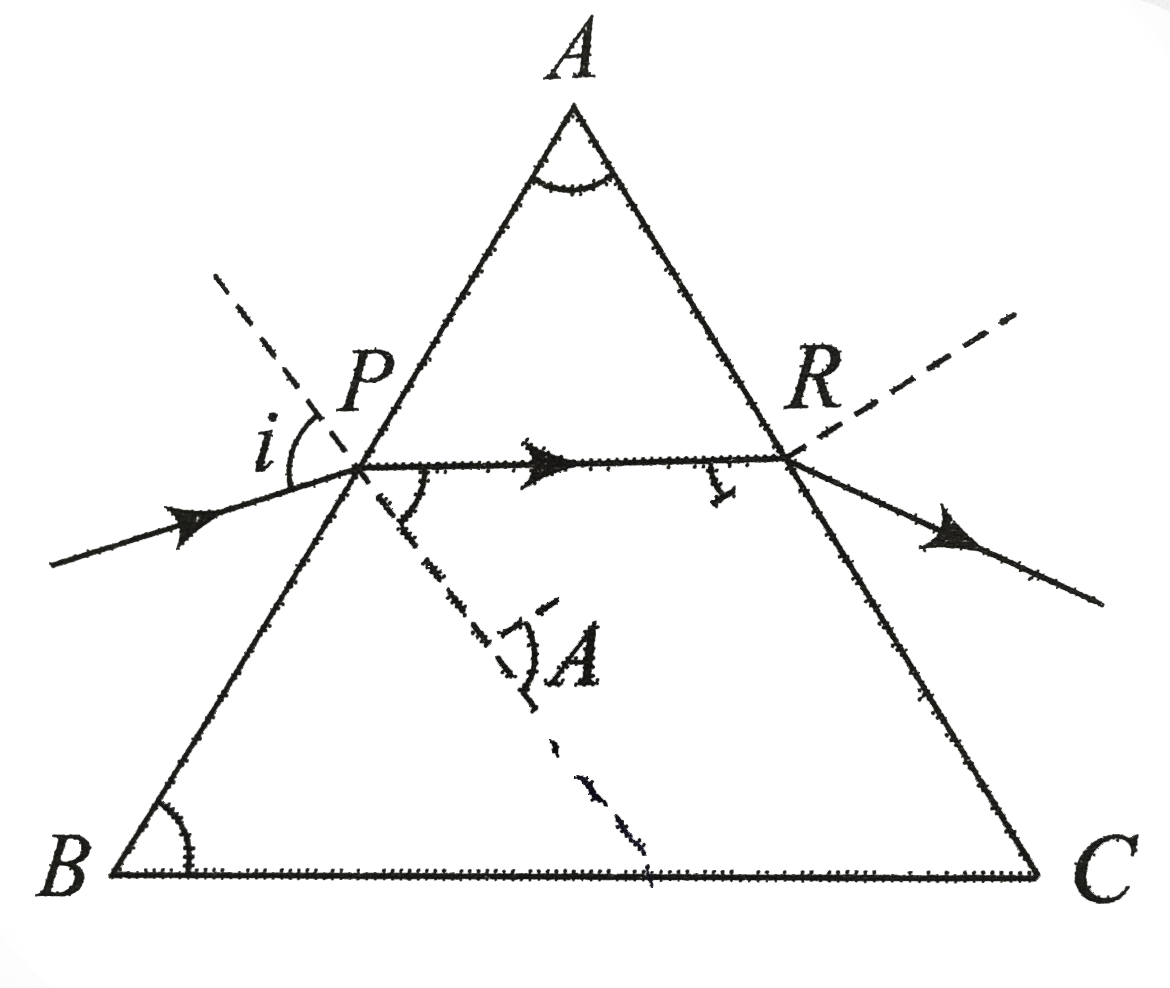
A ray of light undergoes deviation of ${30}^{\circ }$ when incident on an equilateral prism of refractive index $\sqrt{2}.$ what is the angle subtended by the ray inside the prism with the base of the prism?

Explanation

To solve the problem step by step, we will follow the logical deductions based on the properties of an equilateral prism and the concept of minimum deviation.

Step 1: Understand the Given Information
We have an equilateral prism with:
- Refractive index \( n = \sqrt{2} \)
- Angle of the prism \( A = 60^\circ \) (since it’s equilateral)
- Angle of deviation \( \delta = 30^\circ \)

Step 2: Check if the Situation is Minimum Deviation
To determine if the deviation is at its minimum, we can use the formula for minimum deviation:
\( n = \frac{\sin\left(\frac{\delta + A}{2}\right)}{\sin\left(\frac{A}{2}\right)} \)
Substituting the known values:
\( n = \frac{\sin\left(\frac{30^\circ + 60^\circ}{2}\right)}{\sin\left(\frac{60^\circ}{2}\right)} = \frac{\sin(45^\circ)}{\sin(30^\circ)} \)
Using the known values of sine:
\( \sin(45^\circ) = \frac{\sqrt{2}}{2}, \quad \sin(30^\circ) = \frac{1}{2} \)
Thus,
\( n = \frac{\frac{\sqrt{2}}{2}}{\frac{1}{2}} = \sqrt{2} \)
Since this matches the given refractive index, we confirm that the situation is indeed one of minimum deviation.

Step 3: Determine the Angles of Refraction
In the case of minimum deviation, the angles of refraction at both faces of the prism are equal:
\( R_1 = R_2 = R \)
From the geometry of the prism, we know:
\( R = \frac{A}{2} = \frac{60^\circ}{2} = 30^\circ \)

Step 4: Find the Angle Subtended by the Ray Inside the Prism
The angle subtended by the ray inside the prism with the base can be determined by considering the geometry:
- The angle of incidence at the first face is \( i = R + \text{angle subtended} \)
- Since the ray is refracted at an angle \( R = 30^\circ \) and the total angle at the base of the prism is \( 60^\circ \), the angle subtended by the ray inside the prism with the base is:
\( \text{Angle subtended} = 60^\circ - R = 60^\circ - 30^\circ = 30^\circ \)

Final Answer
The angle subtended by the ray inside the prism with the base of the prism is \( 30^\circ \).

---