A rod of length 1.05 m having negliaible mass is supported at its ends by two wires of steel (wire A) and aluminium (wire B) of equal lengths as shown in fig. The cross-sectional area of wire A and B are 1mm2 and 2mm2, respectively . At what point along the rod should a mass m be suspended in order to produce (a) equal stresses and (b) equal strains in both steel and aluminium wires. Given,
Ysteel=2×1011Nm−2andY−(aluminium)=7.0×1010N−2
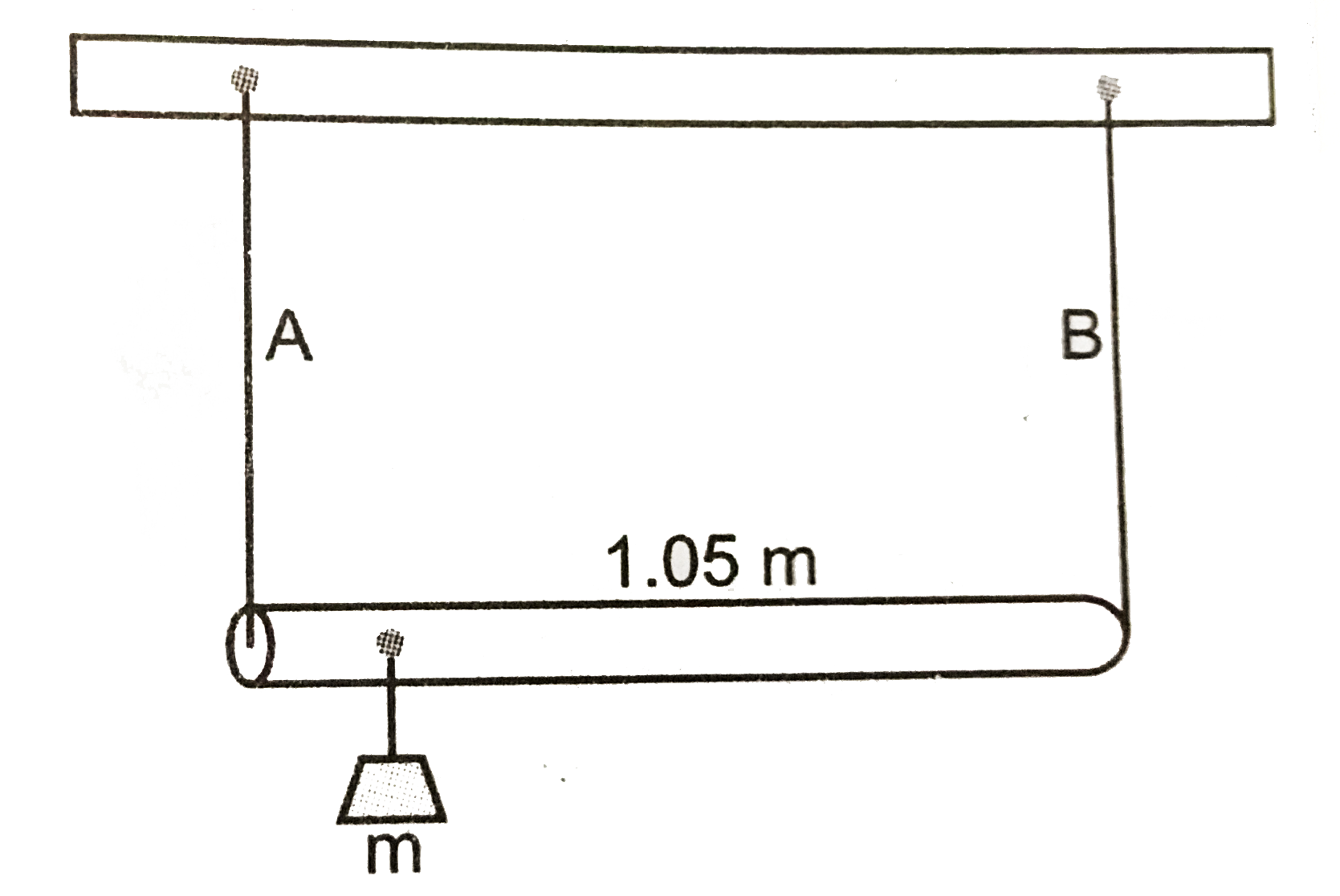
Mass m should by suspended close to wire A to have equal stresses in both the wires
Mass m should be suspended close to B to have equal stresses in both the wires
Mass m should be suspended at the middle of the wires to have equal stresses in both the wires
Mass m should be suspended close to wire A to have strain in both wires
The correct Answer is:B, D
Let the mass is placed at x from the end B.

Let TA and TB be the tensions in wire A and wire B respectively.
For the rotational equilibrium of the system,
Στ=0 (Total torque = 0)
⇒ TBx−TA(l−x)=0
⇒ TBTA=l−xx ....(i)
Stress in wire A=SA=TAaA
Stress in wire B=SB=TBaB
where aA and aB are cross-sectional areas of wire A and B respectively.
By equation aB=2aA
Now, for equal stress SA=SB
⇒ TAaA=TBaB⇒TBTA=aBaA=2
⇒ l−xx=2⇒lx−1=2
⇒ x=l3⇒l−x=l−l/3=2l3
Hence, mass m should be placed closer to B.
For equal strain, (Strain)A=(Strain)B
⇒(YA)SA=YBSB (where YA and YB are Young modulii)
⇒YsteelTA/aA=YAlTB/aB
⇒ YsteelYAl=TATB×aBaA=(xl−x)(2aAaA)
⇒ 200×10970×109=2xl−x⇒207=2xl−x
⇒ 107=xl−x⇒10l−10x=7x
⇒ 17x=10l⇒x=10l17
l−x=l−10l17=7l17
Hence, mass m should be placed closer to wire A.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LONG SHORT ANSWER TYPE QUESTION
16 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Long Answer Type Questions
3 Videos
- MOTION IN A PLANEBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Multiple Choice Questions
37 Videos
Similar Questions
A point mass m is suspended usin two wires of different material as shown in the figure. If corss-section of wire-1 and sore-2 are 3mm2and√3mm2 respectively, which of the following is correct?

View SolutionTwo wires of equal length and cross-section area suspended as shown in figure. Their Young's modulus are Y1 and Y2 respectively. The equavalent Young's modulus will be

View Solution
Knowledge Check
A light rod of length 2 m is suspended from ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross-section 0.1 cm sq. and the other of brass of cross-section 0.2 cm sq. Along the rod at what distance a weight may be hung to produce equal stresses in the wires?
(YSteel=2×1011Nm−2,YBass=1×1011Nm−2)A43m from steel wireB43m from brass wireC1 m from steel wireD14m from brass wire
An iron rod B of length 1 m and mass 1 kg is suspended with help of two wires as shwon in figure. The area of cross-section of iron wire is 0.3 cm2 and of copper wire is 0.6cm2.
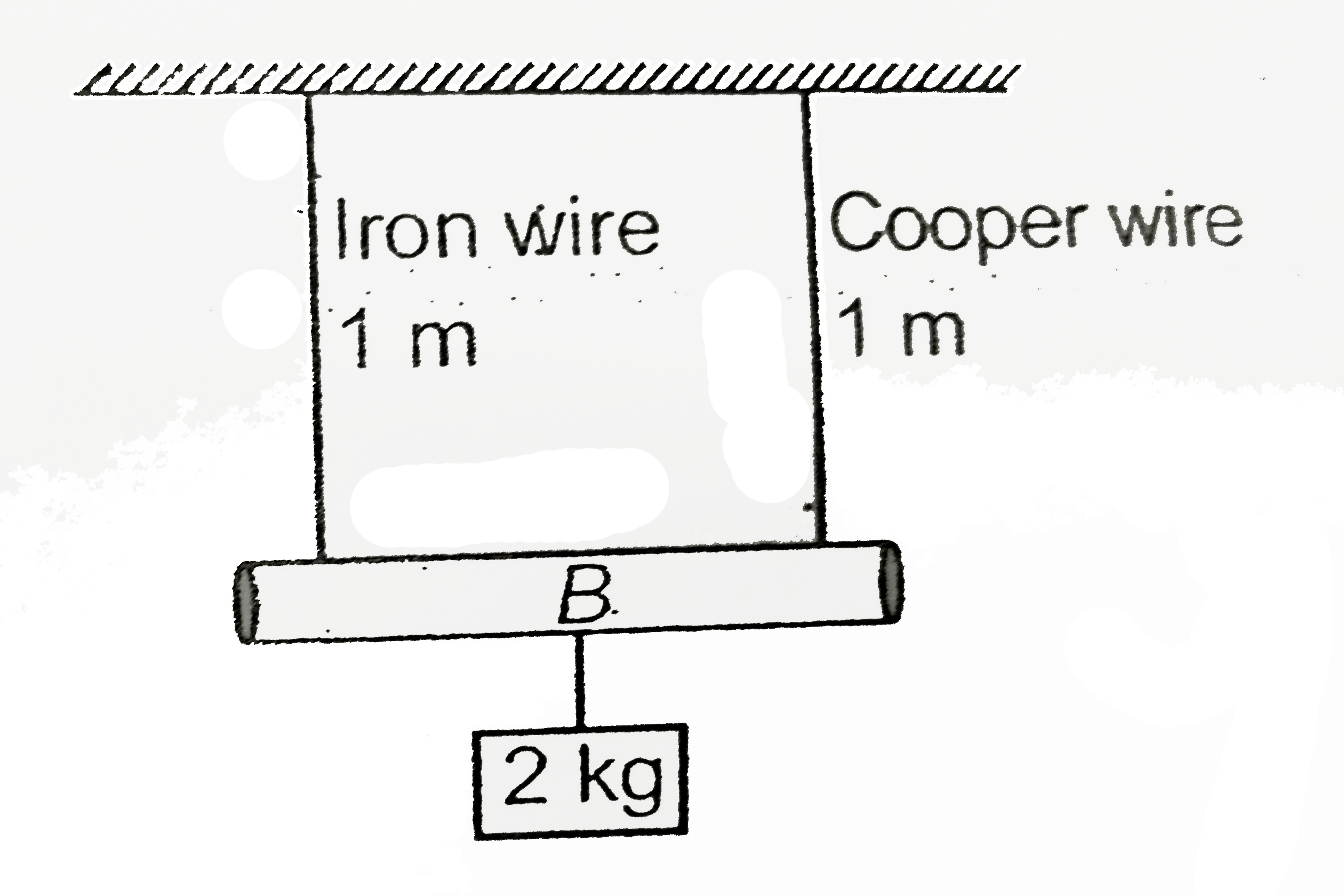
At what distance from iron wire a weight can be hung to produce equal stress in wires?
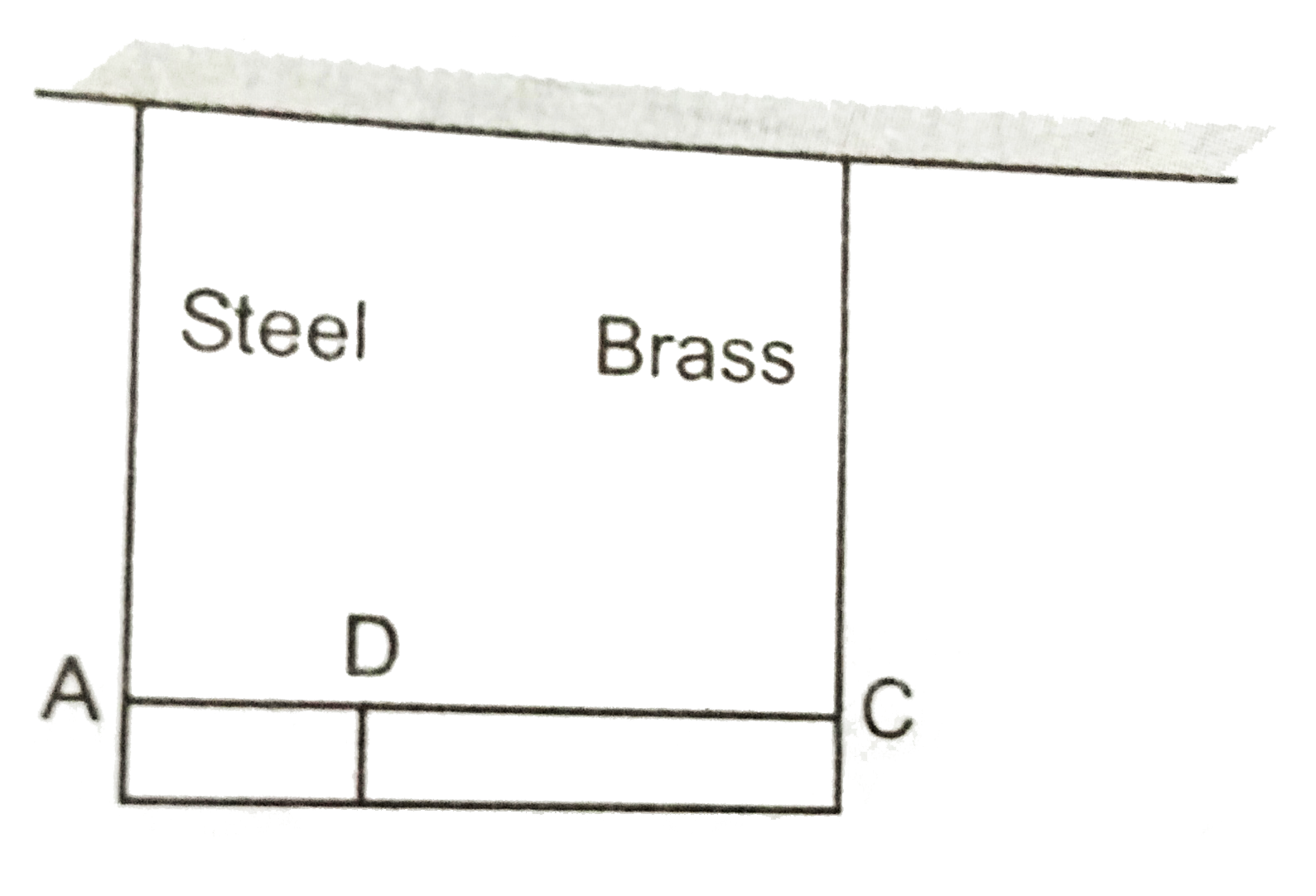
View SolutionA light rod AC of length 2.00 m is suspended from the ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross-section 10−3m2 and the other is of brass of cross-section 2×10−3m2. The position of point D from end A along the rod at which a weight may be hung to produce equal stress in both the wires is [Young's modulus of steel is 2×1011Nm2 and for brass is 1×1011Nm−2]
View SolutionTwo wires of the same material and same length have radii 1 mm and 2 mm respectively. Compare :
their resistances,
View SolutionA steel wire and a copper wire of equal length and equal cross- sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of
copper/steel
(a) the stresses developed . In the two wires,
(b) the strains developed. (Y of steel =2×1011N/m2)
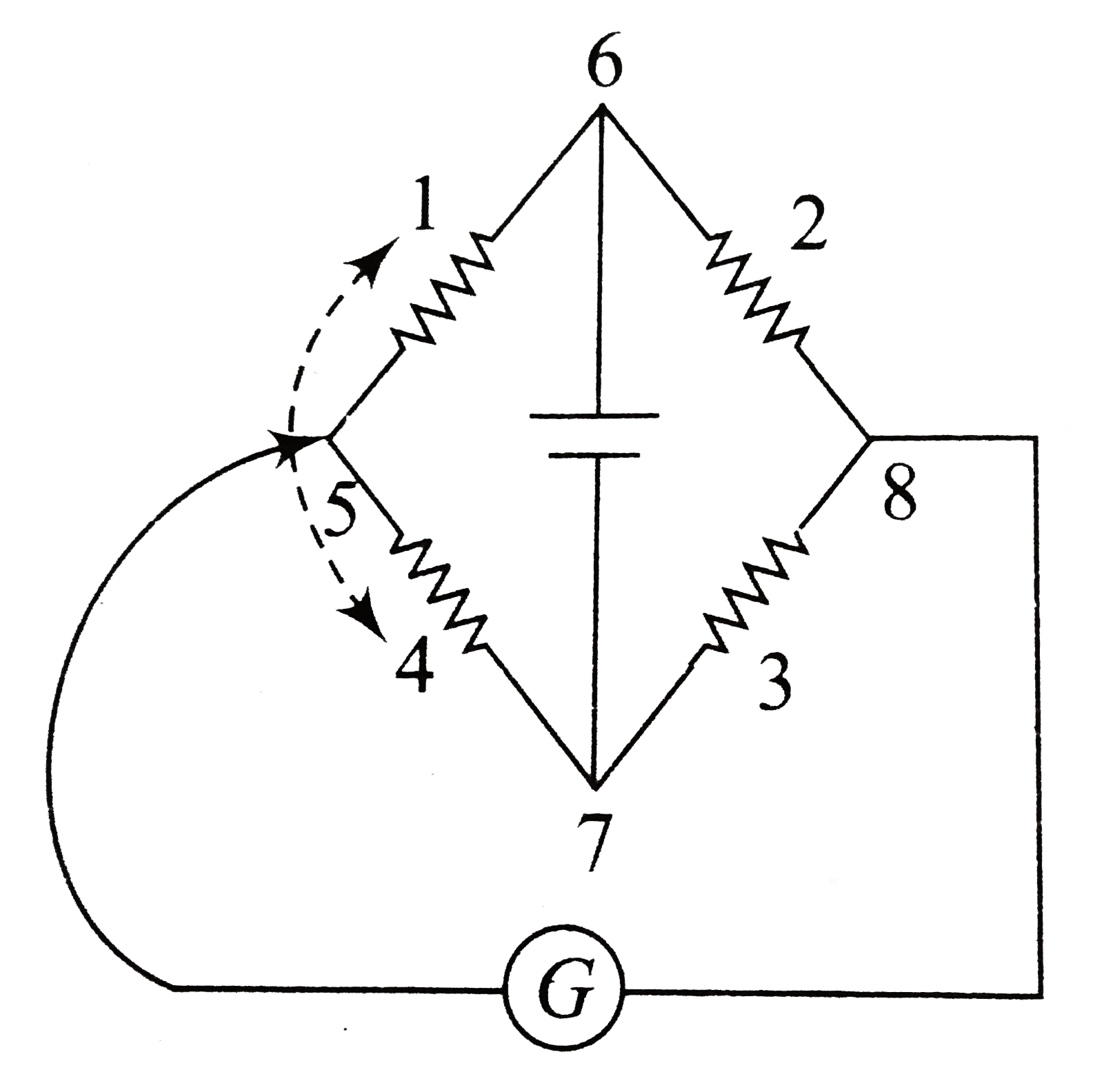
View SolutionFour wires of equal length 2 m are arranged as shown in the figure. Wires , 2, 3 and 4 are of equal cross-sectional area and wire 1 is of half the cross section of these wires. By how much distance pointer at point 5 must be moved to get null point ?
View SolutionA wire of length 1m and radius 1mm is subjected to a load. The extension is x.The wire is melted and then drawn into a wire of square cross - section of side 2mm Its extension under the same load will be
View SolutionAn iron rod B of length 1 m and mass 1 kg is suspended with help of two wires as shwon in figure. The area of cross-section of iron wire is 0.3 cm2 and of copper wire is 0.6cm2.
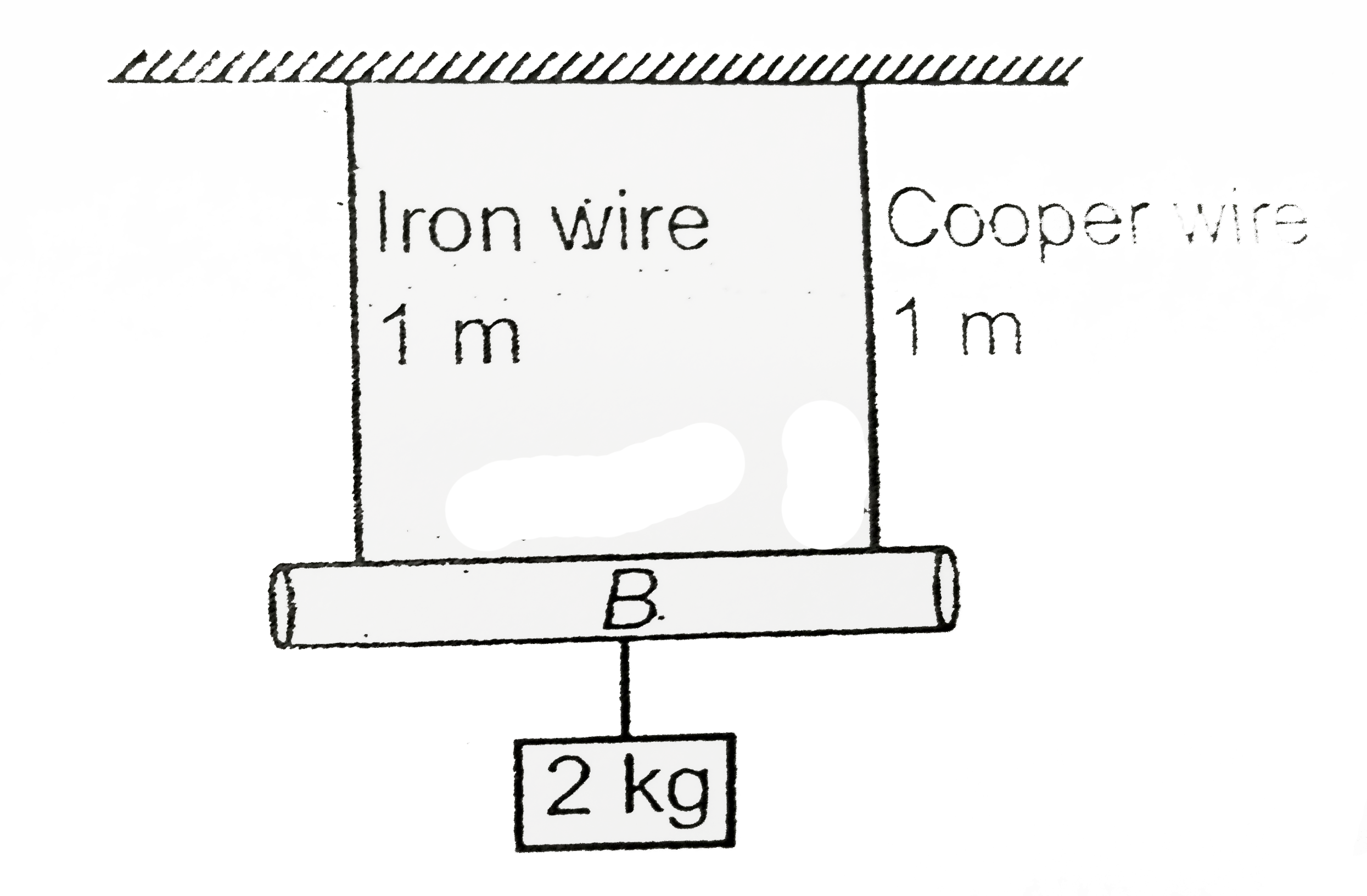
In the above question, if Yiron:YCu=2:1, then the ratio of elastics energy per unit volume stored in iron wire to copper wire is
View Solution
