A solid sphere rolling on a rough horizontl surface with a lilner speed v collides elastically with a fixed, smooth, vertical wall. Find the speed of the sphere after it has started pure rolling in the backward direction.
The correct Answer is:C
To solve the problem of a solid sphere rolling on a rough horizontal surface that collides elastically with a fixed, smooth vertical wall, we will follow these steps:
Step 1: Understand the Initial Conditions
The sphere is initially rolling with a linear speed v and has an angular velocity ω. For a solid sphere, the relationship between linear speed and angular speed is given by:
v=Rω
where R is the radius of the sphere.
Step 2: Analyze the Collision
Since the collision with the wall is elastic, the linear momentum in the direction perpendicular to the wall is conserved. After the collision, the sphere will rebound with the same speed but in the opposite direction. Thus, the linear speed immediately after the collision will be:
v′=−v
Step 3: Determine the Angular Velocity After Collision
The angular momentum of the sphere about its center of mass before the collision is given by:
Li=Iω=25mR2ω
Substituting ω=vR:
Li=25mR2(vR)=25mRv
After the collision, the sphere will have a linear speed of −v and will continue to rotate clockwise. The angular momentum after the collision will be:
Lf=Iω′=25mR2ω′
where ω′ is the new angular velocity.
Step 4: Condition for Pure Rolling
For the sphere to start pure rolling in the backward direction, the condition v′=Rω′ must be satisfied. Thus:
−v=Rω′
This implies:
ω′=−vR
Step 5: Apply Conservation of Angular Momentum
Using the conservation of angular momentum about the point of contact, we can set up the equation:
Li=Lf
Substituting the expressions for Li and Lf:
25mRv=25mR2(−vR)+m(−v)R
Simplifying this equation:
25mRv=−25mRv+m(−v)R
Combining like terms gives:
25mRv+25mRv=−mvR
45mRv=−mvR
Dividing through by mR (assuming m≠0 and R≠0):
45v=−v′
Thus:
v′=−45v
Step 6: Calculate the Final Speed
After the sphere has started pure rolling in the backward direction, we find the speed of the sphere:
v′=37v
Final Answer
The speed of the sphere after it has started pure rolling in the backward direction is:
37v
The sphere is initially rolling with a linear speed v and has an angular velocity ω. For a solid sphere, the relationship between linear speed and angular speed is given by:
v=Rω
where R is the radius of the sphere.
Since the collision with the wall is elastic, the linear momentum in the direction perpendicular to the wall is conserved. After the collision, the sphere will rebound with the same speed but in the opposite direction. Thus, the linear speed immediately after the collision will be:
v′=−v
The angular momentum of the sphere about its center of mass before the collision is given by:
Li=Iω=25mR2ω
Substituting ω=vR:
Li=25mR2(vR)=25mRv
Lf=Iω′=25mR2ω′
where ω′ is the new angular velocity.
For the sphere to start pure rolling in the backward direction, the condition v′=Rω′ must be satisfied. Thus:
−v=Rω′
This implies:
ω′=−vR
Using the conservation of angular momentum about the point of contact, we can set up the equation:
Li=Lf
Substituting the expressions for Li and Lf:
25mRv=25mR2(−vR)+m(−v)R
Simplifying this equation:
25mRv=−25mRv+m(−v)R
Combining like terms gives:
25mRv+25mRv=−mvR
45mRv=−mvR
Dividing through by mR (assuming m≠0 and R≠0):
45v=−v′
Thus:
v′=−45v
After the sphere has started pure rolling in the backward direction, we find the speed of the sphere:
v′=37v
The speed of the sphere after it has started pure rolling in the backward direction is:
37v
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for short Answer21videos
ROTATIONAL MECHANICS
- BOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective -214videos
ROTATIONAL MECHANICS
- BOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer13videos
REST AND MOTION : KINEMATICS
- BOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer15videos
SIMPLE HARMONIC MOTION
Similar Questions
A solid sphere rolls without slipping on a rough horizontal floor, moving with a speed v. It makes an elastic collision with a smooth vertical wall. After impact
View SolutionA solid sphere rolling on a rough horizontal surface. Acceleration of contact point is zero.
A solid sphere can roll on the smooth surface.View Solution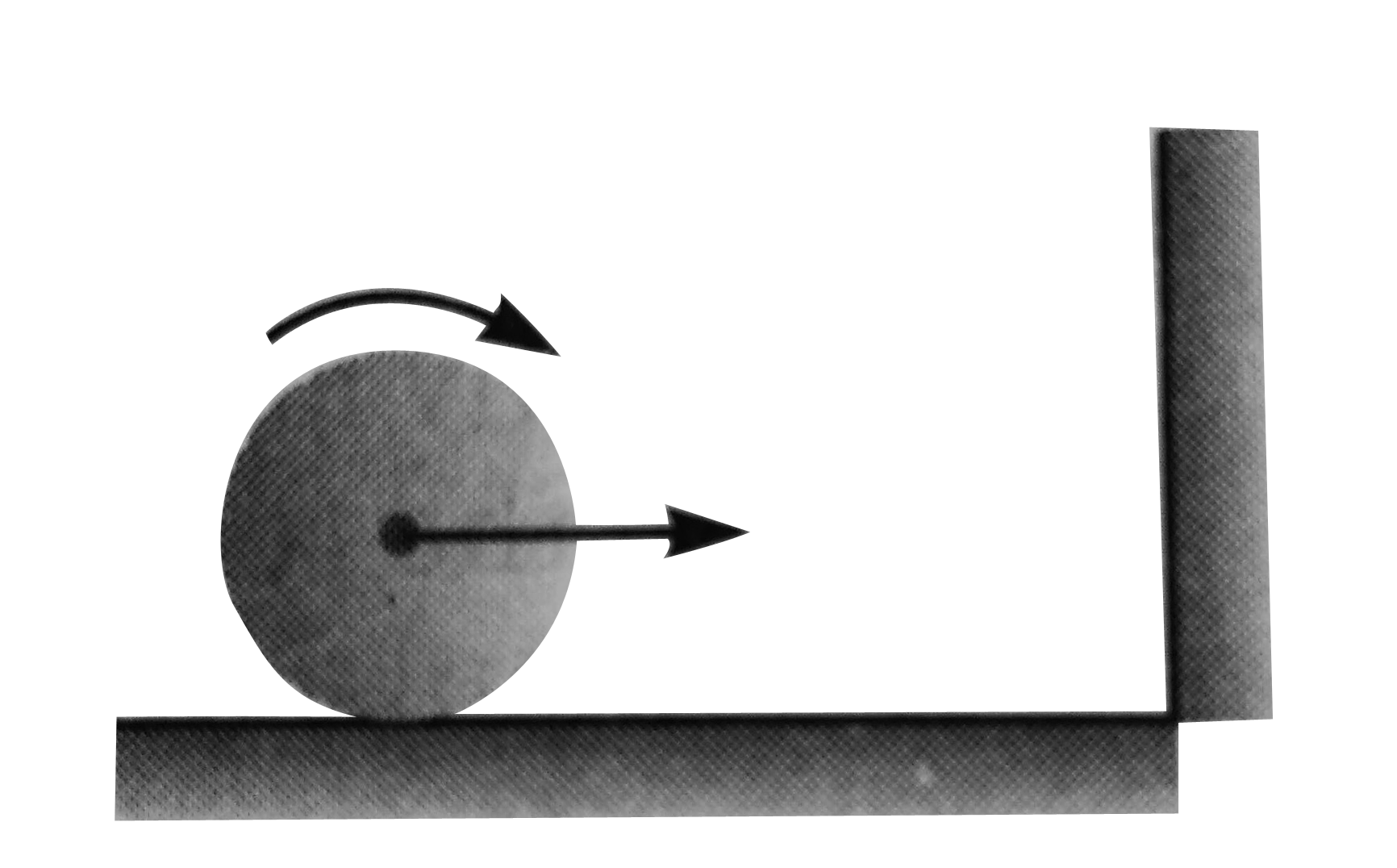
A solid sphere is rolling purely on a rough horizontal surface (coefficient of kinetic friction =μ) with speed of centre =u. It collides inelastically with a smooth vertical wall at a certain moment, the coefficient of restituting being 12. The sphere will begin pure rolling after a time.View SolutionA solid sphere of radius 2.45m is rotating with an angular speed of 10rad/s. When this rotating sphere is placed on a rough horizontal surface then after sometime it starts pure rolling. Find the linear speed of the sphere after it starts pure rolling.
View SolutionA sphere rolling on a horizontal rough surface Collides elastically with a smooth vertical wall, as shown in Fig. State which of the following statements is true or false.
 a. After collision, the velocity of the centre of mass gets reversed.
a. After collision, the velocity of the centre of mass gets reversed.
b. Angular momentum of the sphere about the point of contact with the wall is conserved.
c. Angular momentum of the sphere about a stationary point on the horizontal surface is conserved.
d. Just after collision the point of contact with the horizontal surface is moving towards the wall.
e. After collision the friction force acts on the sphere such that it decreases the linear speed and increases the angular speed.
f. Finally, when the sphere starts rolling, it is moving away from the wall.View SolutionA sphere rolls on a horizontal surface. Is there any point of the sphere which has a vertical velocity?
View SolutionA uniform sphere of radius R is palced on a rough horizontal surface and given a linear velocity v0 and an angular velocity ω0 as shown . If the angular velocity and the linear velocity both become zero simultaneously, then
View SolutionA sphere rolling on a horizontal rough surface collides elastically with a smooth vertical wall, as shown in figure. During collision, angular momentum of the sphere is conserved about ____________. (Any point O on the surface/point of contact P)
 View Solution
View SolutionA solid homogeneous sphere is moving on a rough horizontal surface, partily rolling and partially sliding. During this kind of motion of the sphere.
View SolutionA small uniform solid sphere A rolls down a fixed surface starting at a height h and collides elastically with a sphere B which is identical in size to A but has twice its mass. The speed of the sphere B, just after the collision is
 View Solution
View Solution

