Question

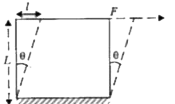
A square lead slab of side 50cm and thickness 5.0cm is subjected to a shearing force (on its narrow face) of magnitude $9.0\times {10}^4$ N. The lower edge is riveted to the floor. How much is the upper edge displaced, if the shear modulus of lead is $5.6\times {10}^{90}$ Pa?

Explanation

To solve the problem, we need to calculate the displacement (ΔL) of the upper edge of the lead slab when a shearing force is applied. We will use the formula for shear strain and shear modulus.

1. Identify Given Values:
- Side of the square slab (L) = 50 cm = 0.50 m
- Thickness of the slab (x) = 5 cm = 0.05 m
- Shearing force (F) = 9.0 × 10^4 N
- Shear modulus (G) = 5.6 × 10^9 Pa

2. Calculate the Area (A) of the face subjected to shearing force:
- Since the slab is square, the area can be calculated as:
\( A = L \times x = 0.50 \, \text{m} \times 0.05 \, \text{m} = 0.025 \, \text{m}^2 \)

3. Calculate the Shear Stress (τ):
- Shear stress is given by the formula:
\( \tau = \frac{F}{A} \)
- Substituting the values:
\( \tau = \frac{9.0 \times 10^4 \, \text{N}}{0.025 \, \text{m}^2} = 3.6 \times 10^6 \, \text{Pa} \)

4. Relate Shear Stress to Shear Strain (φ):
- The relationship between shear stress and shear strain is given by:
\( G = \frac{\tau}{\phi} \)
- Rearranging gives:
\( \phi = \frac{\tau}{G} \)
- Substituting the values:
\( \phi = \frac{3.6 \times 10^6 \, \text{Pa}}{5.6 \times 10^9 \, \text{Pa}} = 6.42857 \times 10^{-4} \)

5. Calculate the Displacement (ΔL):
- Shear strain (φ) is also defined as:
\( \phi = \frac{\Delta L}{L} \)
- Rearranging gives:
\( \Delta L = \phi \times L \)
- Substituting the values:
\( \Delta L = 6.42857 \times 10^{-4} \times 0.50 \, \text{m} = 3.214285 \times 10^{-4} \, \text{m} = 0.0003214285 \, \text{m} \approx 0.321 \, \text{mm} \)

Final Answer:
The upper edge of the lead slab is displaced approximately 0.321 mm.

---