A steel wire of mass μ per unit length with a circular cross-section has a radius of 0.1cm. The wire is of length 10m when measured lying horizontal, and hangs from a hook on the wall. A mass fo 25kg is hung from the free end of the wire. Assume the wire to be uniform and laterla strain << logitudinal strain. If density of steel is 7860kgm−3 and Young's modulus is 2×1011N/m2 then the extension in the length fo the wire is
To solve the problem of finding the extension in the length of the steel wire, we will break it down into steps.
Step 1: Calculate the mass of the wire
The mass per unit length of the wire is denoted as μ. We can calculate the mass of the wire using its density and volume.
1. Calculate the volume of the wire:
V=Cross-sectional area×Length
The cross-sectional area A of the wire (circular cross-section) is given by:
A=πr2
where r=0.1cm=0.001m.
A=π(0.001)2=π×10−6m2
The length of the wire is L=10m.
V=π×10−6×10=1.0×10−5m3
2. Calculate the mass of the wire:
Using the density ρ=7860kg/m3:
m=ρ×V=7860×1.0×10−5=0.0786kg
Step 2: Calculate the extension due to the weight of the wire
The weight of the wire acts at its midpoint. The force due to the weight of the wire is:
F1=m⋅g=0.0786⋅9.81≈0.771N
The extension due to its own weight can be calculated using the formula:
Δl1=F1⋅L2A⋅Y
where Y is Young's modulus =2×1011N/m2.
Substituting the values:
Δl1=0.771⋅102⋅π×10−6⋅2×1011
Calculating the area:
A=π×(0.001)2=3.14×10−6m2
Now substituting:
Δl1=0.771⋅102⋅3.14×10−6⋅2×1011≈0.000123m=1.23×10−4m
Step 3: Calculate the extension due to the mass hung from the wire
The total force due to the mass is:
F2=mmass⋅g=25⋅9.81=245.25N
The extension due to the mass can be calculated using:
Δl2=F2⋅LA⋅Y
Substituting the values:
Δl2=245.25⋅103.14×10−6⋅2×1011≈0.39m
Step 4: Calculate the total extension
The total extension Δl is the sum of both extensions:
Δl=Δl1+Δl2≈1.23×10−4+0.39≈0.39m
Final Answer
The total extension in the length of the wire is approximately 0.39m.
The mass per unit length of the wire is denoted as μ. We can calculate the mass of the wire using its density and volume.
V=Cross-sectional area×Length
The cross-sectional area A of the wire (circular cross-section) is given by:
A=πr2
where r=0.1cm=0.001m.
A=π(0.001)2=π×10−6m2
The length of the wire is L=10m.
V=π×10−6×10=1.0×10−5m3
Using the density ρ=7860kg/m3:
m=ρ×V=7860×1.0×10−5=0.0786kg
The weight of the wire acts at its midpoint. The force due to the weight of the wire is:
F1=m⋅g=0.0786⋅9.81≈0.771N
The extension due to its own weight can be calculated using the formula:
Δl1=F1⋅L2A⋅Y
where Y is Young's modulus =2×1011N/m2.
Δl1=0.771⋅102⋅π×10−6⋅2×1011
Calculating the area:
A=π×(0.001)2=3.14×10−6m2
Now substituting:
Δl1=0.771⋅102⋅3.14×10−6⋅2×1011≈0.000123m=1.23×10−4m
The total force due to the mass is:
F2=mmass⋅g=25⋅9.81=245.25N
The extension due to the mass can be calculated using:
Δl2=F2⋅LA⋅Y
Substituting the values:
Δl2=245.25⋅103.14×10−6⋅2×1011≈0.39m
The total extension Δl is the sum of both extensions:
Δl=Δl1+Δl2≈1.23×10−4+0.39≈0.39m
The total extension in the length of the wire is approximately 0.39m.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LONG SHORT ANSWER TYPE QUESTION
16 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Long Answer Type Questions
3 Videos
- MOTION IN A PLANEBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Multiple Choice Questions
37 Videos
Similar Questions
A block of mass M is suspended from a wire of length L, area of cross-section A and Young's modulus Y. The elastic potential energy stored in the wire is
View SolutionA steel wire 2m long is suspended from the ceiling. When a mass is hung from its lower end, the increase in length recorded is 1cm. Determine the strain in the wire.
View Solution
Knowledge Check
In the question number 46, if the mass M is hung the free end of the wire, then the extension produc in the wire is
AμgL2+MgL2YAB2μgL2+MgLYACμgL2+2MgL2YADμgL2+MgLYAThe area of a cross-section of steel wire is 0.1cm−2 and Young's modulus of steel is 2x1011Nm−2. The force required to stretch by 0.1% of its length is
A1000 NB2000 NCÂ 4000 ND5000NA mild steel wire of length 2L is strethched, within its elastic limit horizontally between two pillars(figure). A mass of m is suspended form the midpont of the wire. Strain in the wire is
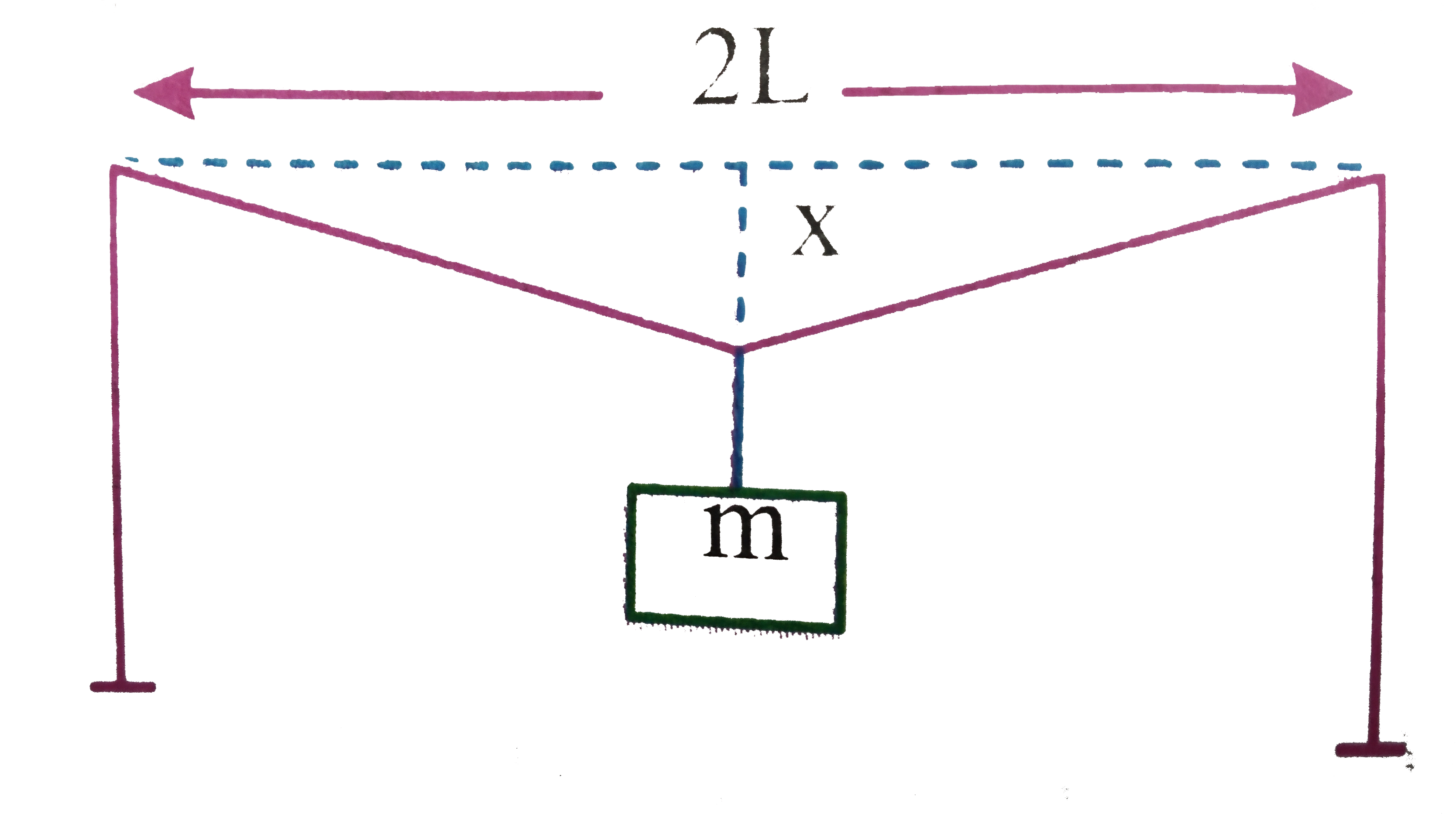 Ax22L2BxLCx2LDx22L
Ax22L2BxLCx2LDx22L
A steel wire 4.0m in length is stretched through 2.0mm.The cross -sectional area of the wire is 2.0mm2.If young's modulus of steel is 2.0×1011N/m2
(a) the energy density of wire,
(b) the elastic potential energy stored in the wire.
View SolutionA metal wire of length L is suspended vertically from a rigid support. When a bob of mass M is attached to the lower end of wire, the elongation of the wire is l:
View SolutionA wire elongates by 1.0 mm when a load W is hanged from it. If this wire goes over a pulley and two weights W each are hung at the two ends the elongation of the wire will be
View SolutionA mild steel wire of length 1.0m and cross sectional area 2L is strethched, within its elastic limit horizontally between two pillars(figure). A mass of m is suspended form the midpont of the wire. Strain in the wire is

View SolutionIn figure the upper wire is made of steel and the lower of copper. The wires have equal cross section. Find the ratio of the longitudinal strains developed in the two wires.

View Solution
