A stone is dropped from the top of a tower of height 60 m. At the same time, another stone is thrown up from the foot of the tower with a velocity 20 m/s. Calculate the displacement of the centre of mass of the two stones at time of collision. (g=10 m/s2)
Initially the centre of mass of the two stones is at a height of 30 m from the ground. Acceleration of the centre of mass
=m×g+m×gm+m=g (downward)
Let the stones colide after time t.
At that time distance travelled by the first stone =12gt2
and distance travelled by the second stone = ut−12gt2
Now, 12gt2+ut−12gt2=60
∴ t=60u=6020=3s
Initital speed of the centre of mass,
ucm=m×0+m×20m+m = 10 m/s (upward)
∴ Displacement of the centre of mass
=ucm×t−12gt2=10×3−12×10×9=−15m
Therefore, the centre of mass will move downward by 15 m.
Topper's Solved these Questions
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
18 Videos
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - EXERCISE (Multiple Choice Questions )
14 Videos
- SIMPLE HARMONIC MOTIONBOOK - CHHAYA PUBLICATIONCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - CBSE SCANNER
16 Videos
- SUPERPOSITION OF WAVESBOOK - CHHAYA PUBLICATIONCHAPTER - SUPERPOSITION OF WAVESEXERCISE - CBSE Scanner
14 Videos
Similar Questions
A stone is released from the top of a tower of height 1960 m. calculate the time taken by the stone to hit the ground.
View SolutionA stone is dropped from the top of a tower 200 m high. At the same time, another stone is projected vertically upwards from the ground with a velocity of 40m/s. Calcuate when and where the two stones will mest.
View SolutionA stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25ms.Calculate when and where the two stones will meet.
View SolutionA stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25m/s. Calculate when and where the two stone will meet.
View SolutionA stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25m/s. Calculate when and where the two stones will meet.
View Solution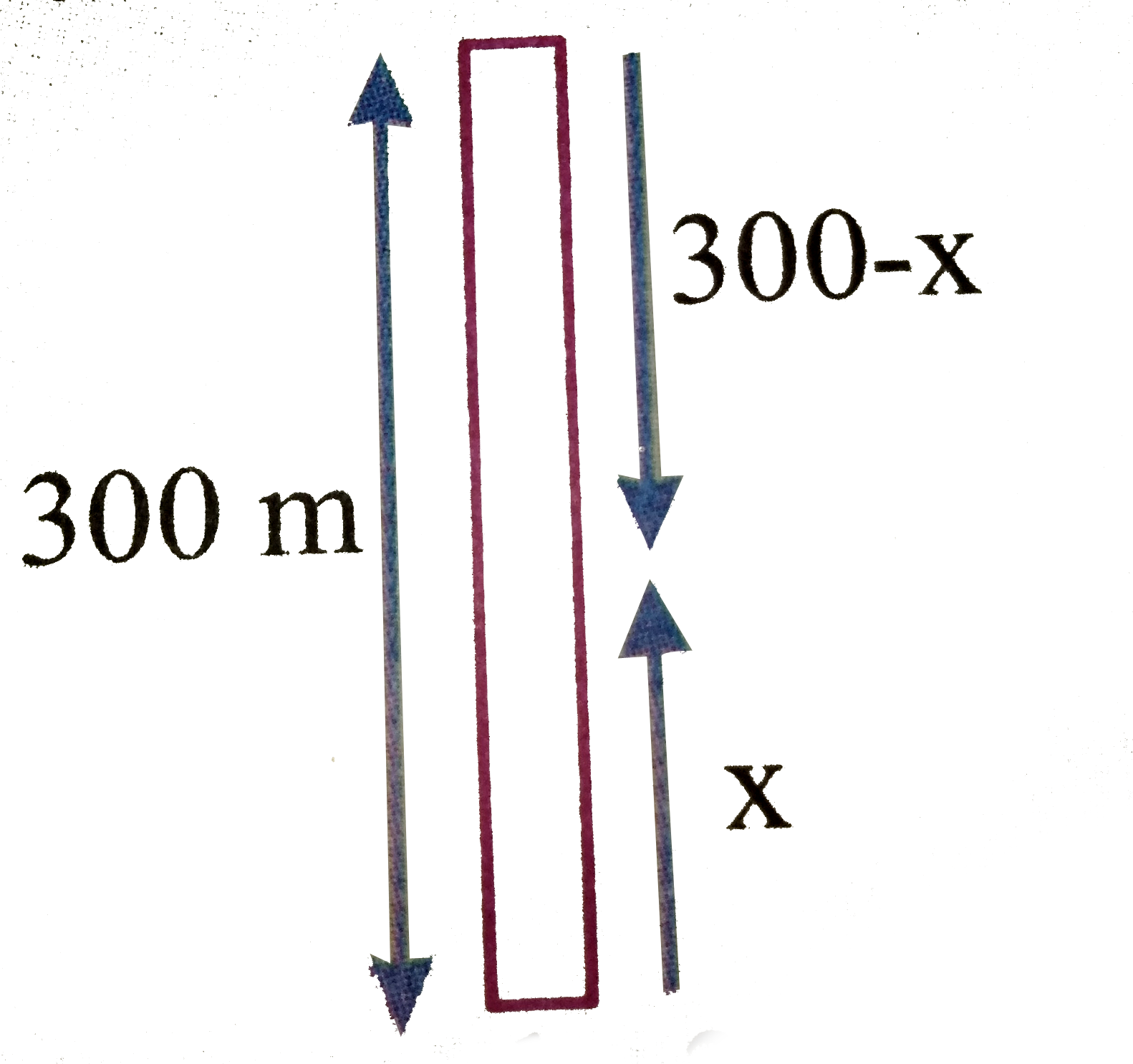
A stone is allowed to fall from the top of a tower 300 m height and at the same time another stone is projected vertically up from the ground with a velocity 100m/s. Find when and where the two stones meet?
View SolutionA stone is dropped from the top of a tower of height 100 m. The stone penetrates in the sand on the ground through a distance of 2m. Calculate the retardation of the stone.
View SolutionA stone is dropped from the top of a tower of height 100 m. The stone penetrates in the sand on the ground through a distance of 2m. Calculate the retardation of the stone.
View SolutionA stone is allowed to fall from the top of a tower 300 m height and at the same time another stone is projected vertically up from the ground with a velocity 100ms−1 . Find when and where the two stones meet ?
View SolutionA stone is dropped from a tower of height 200 m and at the same time another stone is projected vertically up at 50 m/s. The height at which they meet ? [g=10m/s2]
View Solution
