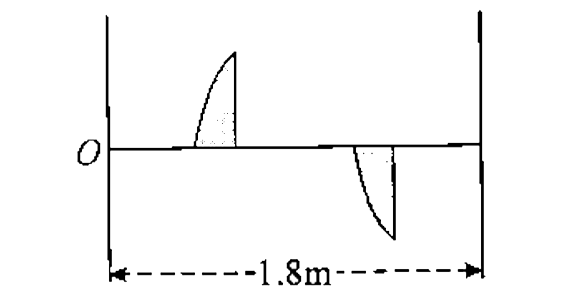
A thin plano-convex lens of focal length f is split into two halves. One of the halves is shifted along the optical axis as shown in figure. The separation between object and image planes is 1.8 m. The magnification of the image, formed by one of the ball lens is 2. Find the focal length of the lens and separation between the two halves. Draw the ray diagram for image formation.
The correct Answer is:D
For both the halves, position of object and image is same. Only difference is of
magnification. Magnification for one of the halves is given as 2(>1). Thus can be for the first
one, because for this, |v|>|u|. Therefore, magnification, |m|=|v/u|>1.
So, for the first half
|v/u|=2
|v|=2|u|
or Let u=−x,
then v=+2x
and |u|+|v|=1.8m
i.e. 3x=1.8m
or x=0.6m
Hence, u=−0.6m
and v=+1.2m
using 1f=1v−1u=11.2−1−0.6=10.4
∴f=0.4m
For the second half, 1f=11.2−d−1−(0.6+d)
or 10.4=11.2−d−1−(0.6+d)
Solving this, we get d=0.6m
Magnification for the second half will be
m2=vu=0.6−(1.2)=−12
and magnification for the first half is
m2=vu=1.2−(0.6)=−2
the ray diagram is as follows:

Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Solved Example
15 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Exercise 31.1
3 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Example Type 10
1 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
Similar Questions
Knowledge Check
A concave lens forms the image of an object such that the distance between the object and image is 10cm and the magnification produced is 1/4. The focal length of the lens will be
A−6.2cmB−4.4cmC−8.6cmD−10cm
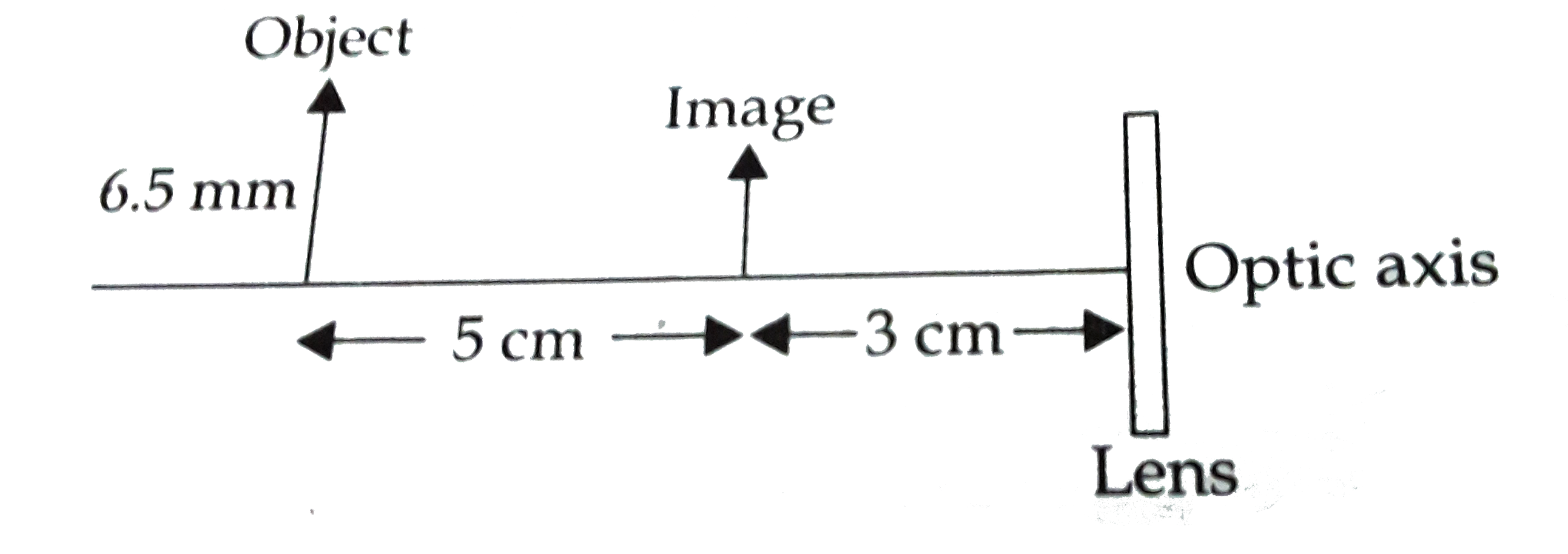
Shown an object and its image formed by a thin lens. Then the nature and focal length of lens is
View SolutionThe distance between an object and its upright image is 20cm. if the magnification is 0.5, what is the focal length of the lens that is being used to form the image?
View SolutionA convex lens of focal length f is placed somewhere in between an object and a screen. The distance between the object and the screen is x. If the numerical value of the magnification produced by the lens is m, then the focal lnegth oof the lens is .
View SolutionA cocave lens forms the image of an object such that the distance between the object and image is 10 cm and the magnification produced is 1/4. the focal length of the lens will be
View SolutionThe distance between the object and its real image from the convex lens is 60 cm and the height of image is two times the height of object . The focal length of the lens is
View SolutionGraph of position of image vs position of point object from a convex lens is shown. Then, focal length of the lens is
_E01_287_Q01.png)
View SolutionA lens forms an upright and diminished image of an object when the object is placed at the focal point of the given lens.
Draw a ray diagram to show the image formation.
View Solution
